Additional Homework Problems

CDP2-A_B Solution

1. Over what range of conversions are the plug-flow reactor and CSTR volumes identical?

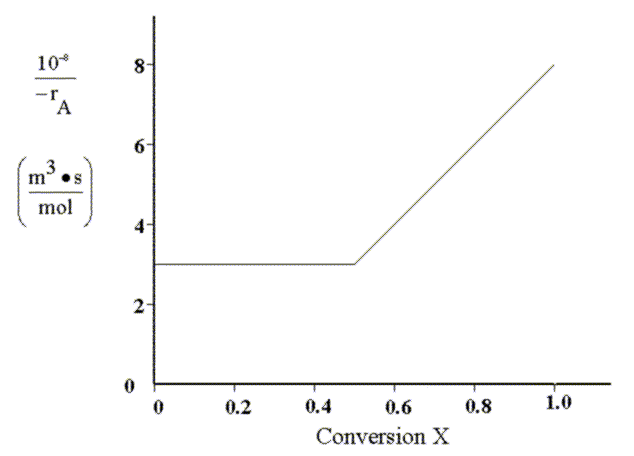
   So that it is easier to visualize the solution, we first plot the inverse of the reaction rate versus conversion. This type of plot is often called a "Levenspiel Plot.":

   

    

   Recalling the mole balance equations for a CSTR and a PFR:

   

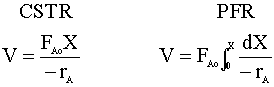
   Until the conversion (X) reaches 0.5, the reaction rate is independent of conversion and the reactor volumes will be identical.

   i.e.

   

    

2. What conversion will be achieved in a CSTR that has a volume of 90 L?

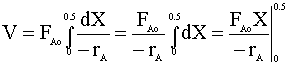
   For now, we will assume that conversion (X) will be less that 0.5. We start with the CSTR mole balance:

   

    

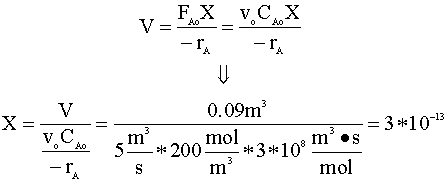
   Our calculated conversion is extremely small.

    

3. What plug-flow reactor volume is necessary to achieve 70% conversion?

   This problem will be divided into two parts, as seen below:

   

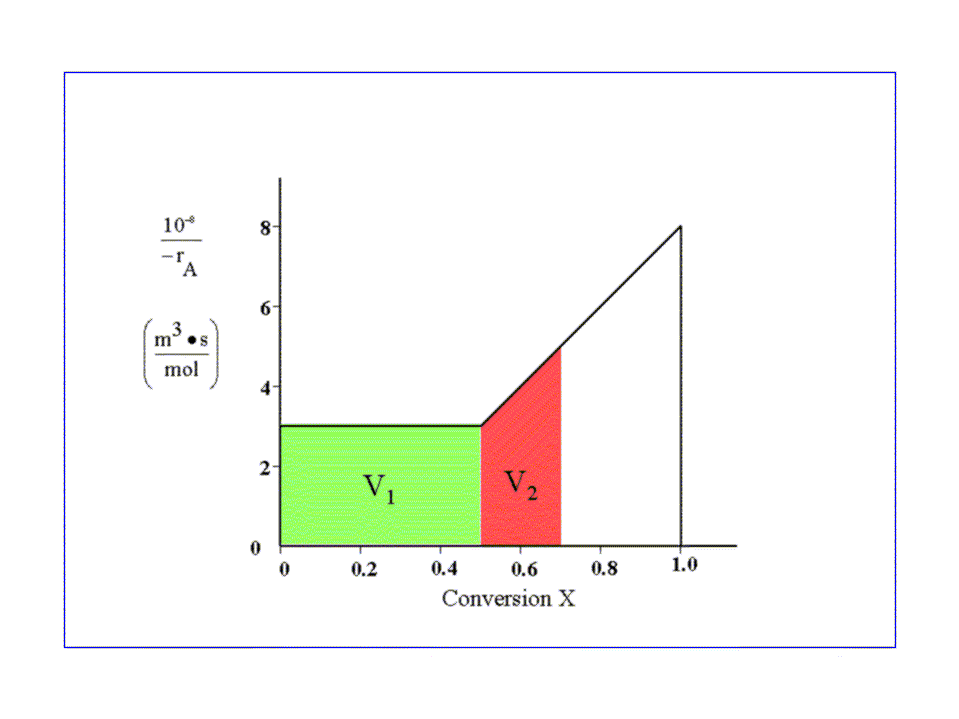
   1. The PFR volume required in reaching X=0.5 (reaction rate is independent of conversion).

      

       

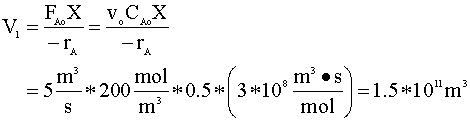
   2. The PFR volume required to go from X=0.5 to X=0.7 (reaction rate depends on conversion).

   

    

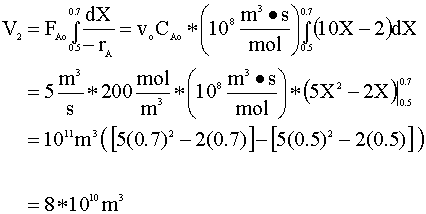
   Finally, we add V₂ to V₁ and get:

   V_tot = V₁ + V₂ = 2.3*10¹¹ m³

    

4. What CSTR reactor volume is required if effluent from the plug-flow reactor in part (c) is fed to a CSTR to raise the conversion to 90%?

   

    

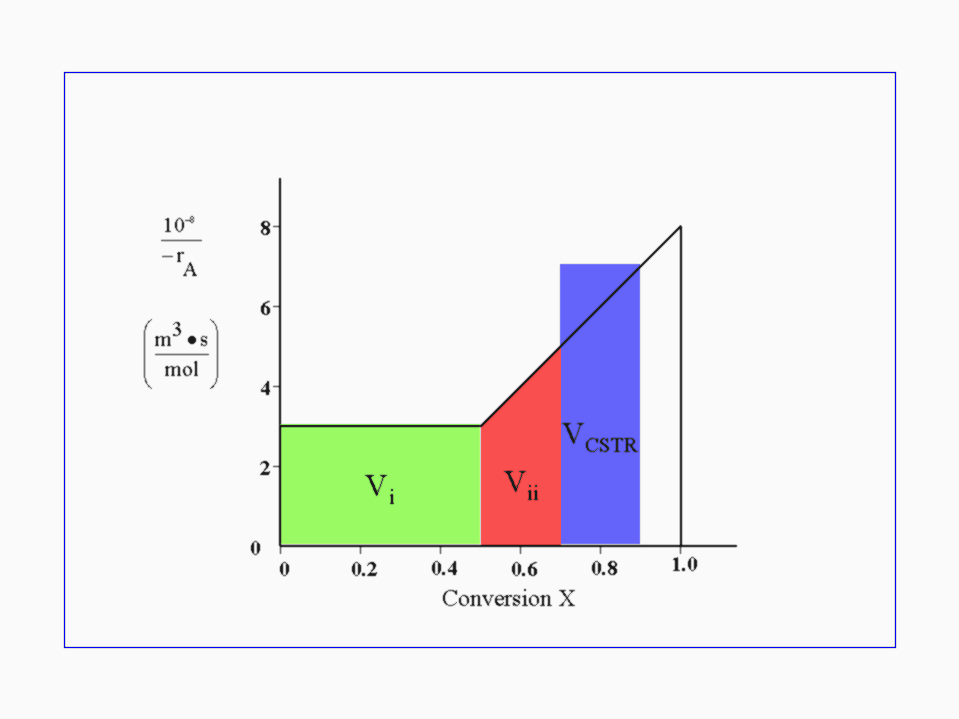
   We notice that the new inverse of the reaction rate (1/-r_A) is 7*10⁸. We insert this new value into our CSTR mole balance equation:

   

    

5. If the reaction is carried out in a constant-pressure batch reactor in which pure A is fed to the reactor, what length of time is necessary to achieve 40% conversion?

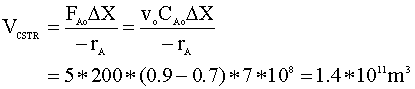
   We will begin with the mole balance on a batch system. Since there is no flow into or out of the system, it can be written as:

   

    

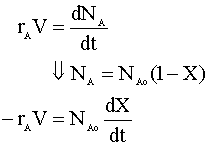
   From the stoichiometry of the reaction we know that V = V_o(1+e X) and e is 1. We insert this into our mole balance equation and solve for time (t):

   

    

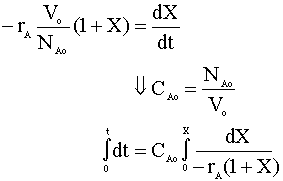
   After integration, we have:

   

    

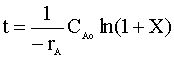
   Inserting the values for our variables:

   

    

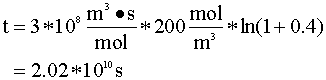
   That is 640 years.

    

6. Plot the rate of reaction and conversion as a function of PFR volume.

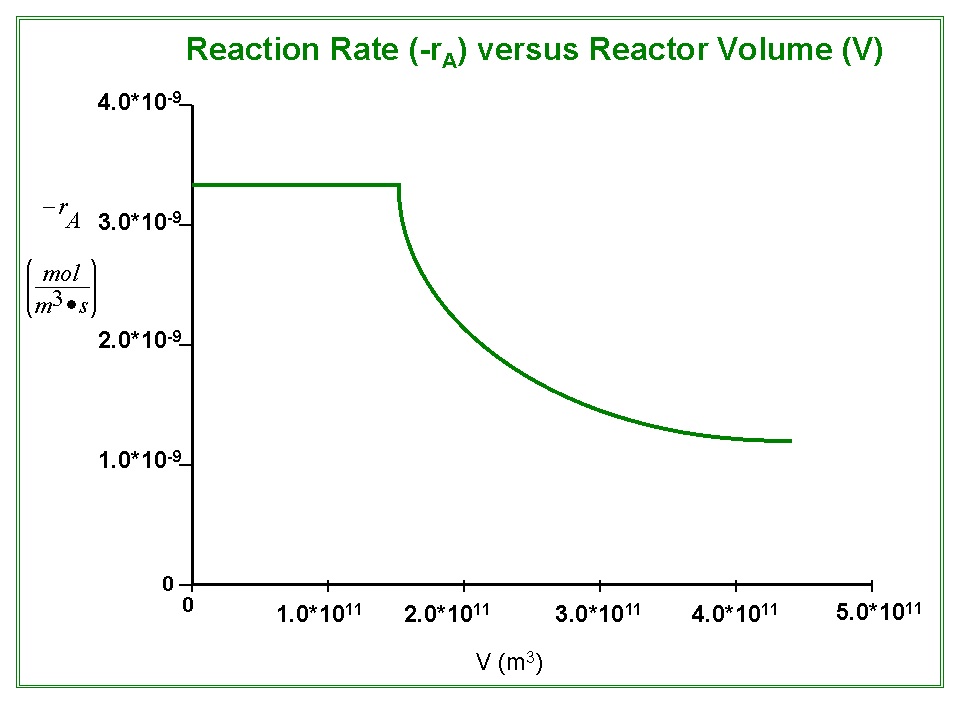
   The following graph plots the reaction rate (-r_A) versus the PFR volume:

   

    

   Below is a plot of conversion versus the PFR volume. Notice how the relation is linear until the conversion exceeds 50%.

   

    

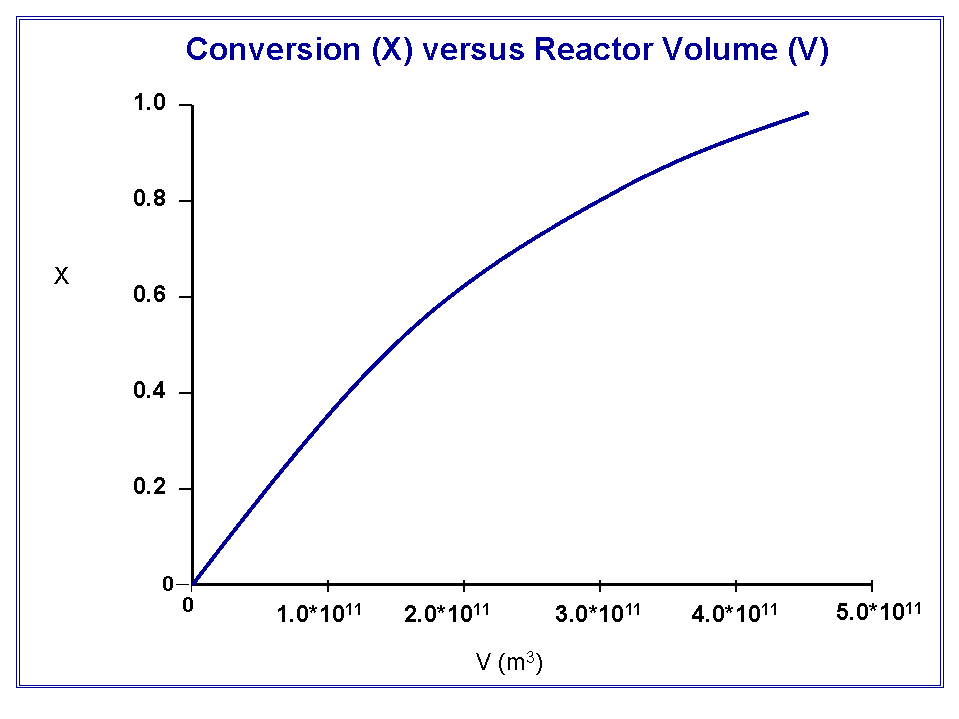
   The volume required for 99% conversion exceeds 4*10¹¹ m³.

    

7. Critique the answers to this problem.

   The rate of reaction for this problem is extremely small, and the flow rate is quite large. To obtain the desired conversion, it would require a reactor of geological proportions (a CSTR or PFR approximately the size of the Los Angeles Basin), or as we saw in the case of the batch reactor, a very long time.