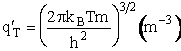������������������������������������������������
(T1)�������������������������������
����� To show
�������������������������������������������������������
������������������������������������������������
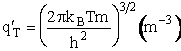(T1)
����� Translational Energy
����� We solve the Schr�dinger equation for the energy of a molecule trapped in an infinite potential well. This situation is called �a particle in a box�. For a particle in a box of length a
��������������������������������������������������������� V(X)
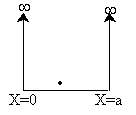
����� The potential energy is zero everywhere except at the walls where it is infinite so that the particle cannot escape the box. Inside the box
���������������������������������������������������������������
��������������������������������������������������������
(T2)
����� The box is a square well potential where the potential is zero between x = 0 and x = a but infinite at x = 0 and x = a (A5p392). The solution is to the above equation is
����������������������������������������������������� 
����������������������������������������������
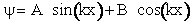(T3)
����������� where�����������������������������������������������
�������������������������������������������������������������
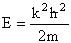(T4)
������������������������������������������������������������ 
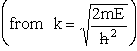
����� We now use the boundary conditions
|
|
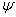= 0, sin 0
= 0, and cos 0=1
�����therefore� B = 0
����� the wave equation is now
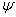����������� = A sin kx
����� At� x = a 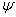= 0
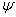����� will be zero provided
����� therefore ka = n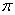
����������� where n is an integer, 1, 2, 3
����������� 
����������������������������������������������������������������������������������������������������������������������������
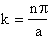(T5)
����� Substituting (T5) into (T4) we see that only certain energy states are allowed
�������������������������������������������������������������������
������������������������������������������������������������
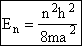(T6)
����� For particle in a a 3-D box of sides a, b, and c
�������������������������������������������������������� 
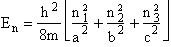
����� Back to one dimension
������������������������������������������������������������������
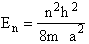
����� Therefore relative to the lowest energy level n = 1, the energy is
�������������������������������������� 
�������������������������������
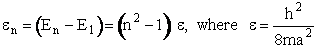(T7)
����� Then
������������������������������������������������������������� 
������������������������������������������������������
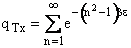(T8)
��������������������������������������������������������������
���������������
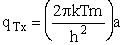(T9)���������������������
Return to Transition State Theory
