Transition State Theory

Derivation of Vibrational Partition Function q_v

  To show

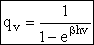
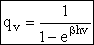
                                                                                                          (Derive) (A12)

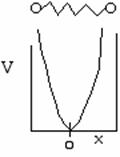
      Again we solve the wave equation for two molecules undergoing oscillation about an equilibrium position x = 0. The potential energy is shown below as a function of the displacement from the equilibrium position x = 0 (A5p402)

      The uncertainty principle says that we cannot know exactly where the particle is located. Therefore zero frequency of vibration in the ground state, i.e. u = 0 is not an option (A5p402 and pA22). When v_o is the frequency of vibration, the ground state energy is

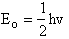                                                                                                                                    (V1)

      Harmonic oscillator (A5p402)

      Spring Force 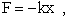 potential energy from equilibrium position x = 0

      the solution is of the form for t=0 then x=0

      where

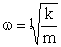
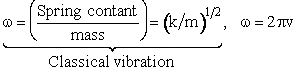
      The potential energy is

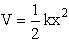                                                                                                                                    (V2)

      We now want to show

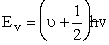                                                                                                                          (V3)

      We now solve the wave equation:

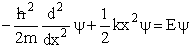                                                                                                      (V4)

      to find the allowable energies, .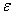

      Let , , 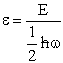where , , and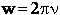

      With these changes of variables Eqn. (A15) becomes

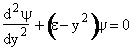                                                                                                                    (V5)

      The solutions to this equation (A5 pA22, i.e., Appendix 8)will go to infinity unless

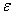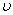                                                                        = 2+1

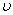                                                                  = 0, 1, 2, 3 . . .

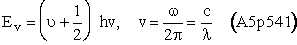                                                     [c = speed of light]

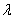                                                                  = wave length

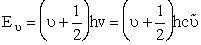                                                                                                   (V6)

      Measuring energy relative to the zero point vibration frequency, i.e., 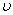 = 0

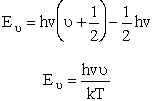
      Substituting for 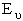 in the partition function summation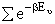

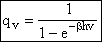                                                                        (V7)

      For , we can make the approximation

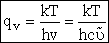                                                                                                                          (V8)

      For m multiple frequencies of vibration

      Order of Magnitude and Representative Values

      For H₂O  we have three vibrational frequencies with corresponding wave numbers, .

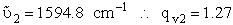
      and

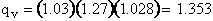
Return to Transition State Theory