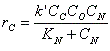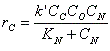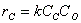7. Reaction Mechanisms, Pathways, Bioreactions and Bioreactors
Additional Homework Problems
CDP7-CB
Streptomyces a mycelial organism, is grown commercially in a batch fermentor using glucose as the primary nutrient to produce various antibiotics. Air is bubbled through the liquid containing these mycelial cells such that the fermentor is analogous to a slurry reactor. The growth rate of the cells is a function of oxygen concentration. The cells grow in clumps and consequently oxygen must diffuse through the clumps to reach the inner core of the clump. As it diffuses it is consumed by the outer core, analogous to diffusion with reaction in a catalyst pellet. The growth rate of the cells is
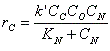
Cc = concentration of cells, g/dm3
Co = concentration of oxygen (O2), mol/dm3
CN = concentration of nutrient, mol/dm3
rc = cell growth rate, g/s/dm3
Because the nutrient is in excess
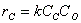
- Develop equations for the percentage resistance of each of the oxygen transport steps for
- slow oxygen consumption rate by the cell.
- fast oxygen consumption rate.
- What parameters should be changed to increase the growth rate?
- Using any appropriate analogies with slurry reactors, derive an equation for cell concentration as a function of time. Assume that the cell clumps remain all the same size (i.e., only generation of new clumps occurs).
- Repeat Part C assuming that no new clumps are produced, so that clumps only grow in size.
- Obtain numerical values for your model parameters in Part C and plot cell concentration as a function of time [see S. J. Pirt Principles of Microbe and Cell Cultivation, New York: Halsted press (1975)]. Carry out a parameter sensitivity study on your model.