![]()
|
|
Type 5 Home Problem -- Solution
"What if …" problems that promote discussion.
There are a few variations in this solution, as compared to the Homogeneous Example 4 Solution. We remove the diffusion term (RB) from the differential equation for the hydrogen (B) flow rate.
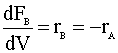
We will need to solve the differential equations for each of the species in our PFR simultaneously. The list of equations can be seen in the Polymath screen-shot below:
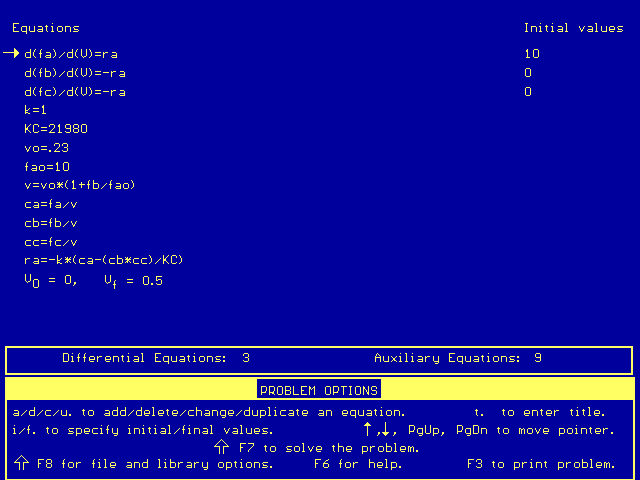
The conversion can be found by using information from this Polymath output:
Conversion, X = (FA0 - FA)/FA0. So with our data, X = (10 - 2.3)/10 = 0.77, or 77 percent.
Next we plot the flow rates versus the reactor volume:
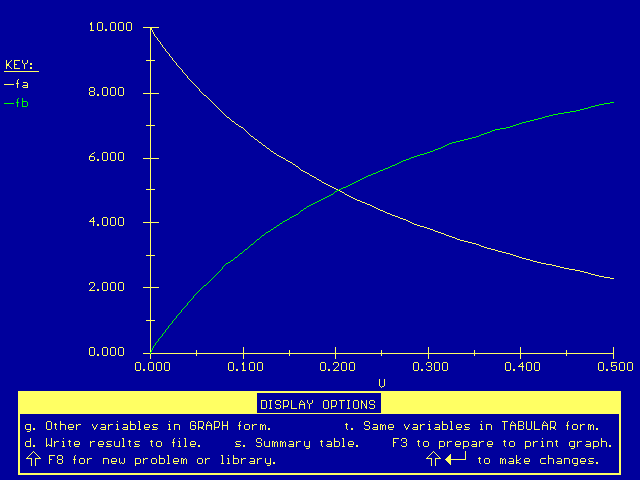
The flow of hydrogen (B) at the end of the reactor is much higher in this plot than in the membrane reactor plot. The extra presence of hydrogen (B) has lowered the reaction rate and the conversion. As with any reversible (equilibrium) reaction, we expect that as the concentration of products increases, the driving force for creating more products will decrease, according to Le Chatelier's Principle. This will prevent the reaction from going to completion, resulting in a drop in conversion.
In order to achieve an 86% conversion, we will need to have an outlet flow rate of product (B) that is roughly 8.6 moles/min. [Recall that X = (FA0 - FA)/FA0, so FA = FA0(1 - X).] The following Polymath table tells us how much reactor volume we need to achieve 86% conversion:
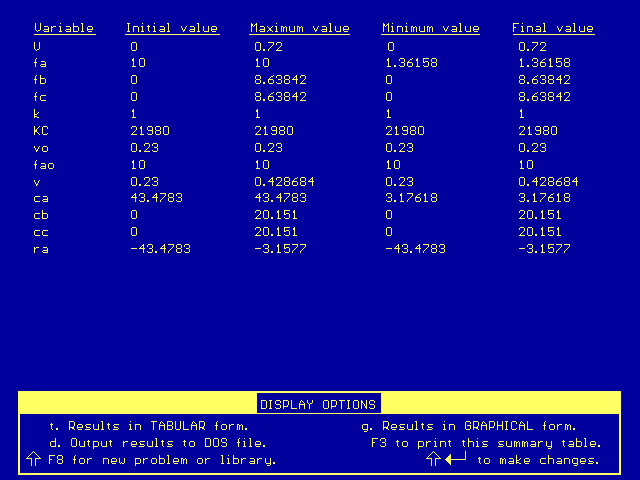
Compared to the membrane reactor (V = 0.5 dm3), this reactor (V = 0.72 dm3) requires 44% more volume to achieve the same conversion.
![]() Back to Homogeneous Example 5
Back to Homogeneous Example 5
CRE Thoughts Ten Types Homogeneous Example 5