9. Unsteady State Non-Isothermal Reactor Design*

Topics

  1. Batch Reactors with Heat Effects Example Index entry from CRE book.
  2. Control of Chemical Reactors Index entry from CRE book.
  3. Linearized Stability Theory
  4. Predicting the Behavior of a CSTR using LST

1. Batch Systems with Heat Effects Example top

Balance on a system volume that is well-mixed:

Adiabatic batch reactor with no work:



Polymath

The following reaction occurs in a batch reactor:

1)

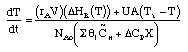2)

3)

4)

5)

6)

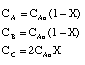7)

Parameter Values

Adiabatic Reaction

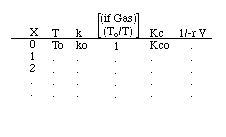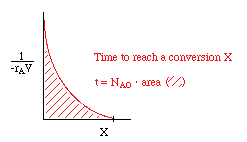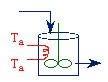
or use one of the integration formulas, e.g.: , to find the reaction time, t. Even better, use Polymath.

Cooling:

 
9.1 Batch Reactor with External Heating
9.2 Text Example 9-2
9.1 Questions related to Problem 9-2


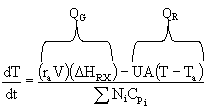
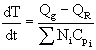
2. Control of Chemical Reactors top

Unsteady State CSTR:

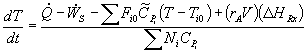For a batch reactor, FAO = 0

Integral Control

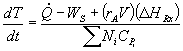For the reaction     in a CSTR:
Embedded Windows Media Player (opens a new window) Embedded Apple QuickTime Player (opens a new window) Direct link to file

Proportional Control

Embedded Windows Media Player (opens a new window) Embedded Apple QuickTime Player (opens a new window) Direct link to file


Integral Controller

Embedded Windows Media Player (opens a new window) Embedded Apple QuickTime Player (opens a new window) Direct link to file


Proportional-Integral Control

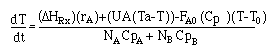3. Linearized Stability Theory top
Energy Balance (Applied to a CSTR)

1.

2.

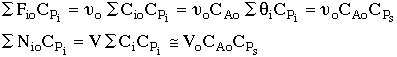3.

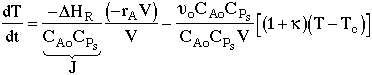4.

 
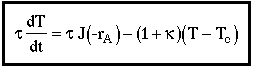CSTR Mole Balance

5.

6.

 
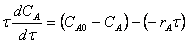Manipulating the Energy and Mole Balances

Let andsignify steady state values. Then at steady state:

7.

Adding equations (6) and (7):

8.

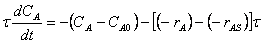Linearizingby expanding it in a Taylor Series:

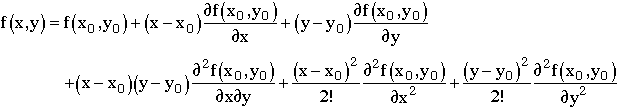To obtain:

9.

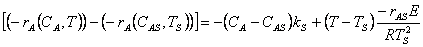10.

Let   

      
      

11.

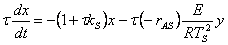12.

13.

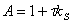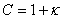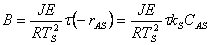Using these substitutions, we can arrive at the following equations that describe the behavior of temperature and concentration, when the steady state conditions are perturbed in a CSTR:

14.

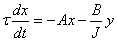15.

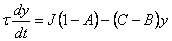16.

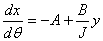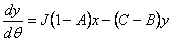17.

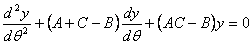18.

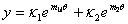19.

20.

21.

22.



4. Predicting the Behavior of a CSTR using LST top

At time t = 0, y1 = y10, where:

Making use of Equation 20

we notice that for the case of b2 = 4c:

if b < 0 ,   then the amplitude (i.e., T - TS) will increase

if b > 0 ,   then the amplitude (i.e., T - TS) will decrease

and that:

if b2 > 4c ,   thenis real (i.e., non-oscillatory behavior)

if b2 < 4c ,   thenis imaginary (i.e., oscillatory behavior)


 

  1. Critically damped: b is positive andis real
  2. Unstable growth: b is negative andis real
  3. Oscillatory and damped: b is positive andis imaginary
  4. Oscillatory: b is zero andis imaginary
  5. Unstable growth oscillation: b is negative andis imaginary

Reference:
R. Aris, Elementary Chemical Reactor Analysis, Prentice Hall, New Jersey, (1969).


Object Assessment of Chapter 9
 

* All chapter references are for the 4th Edition of the text Elements of Chemical Reaction Engineering .

top

Fogler & Gurmen
© 2008 University of Michigan