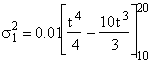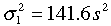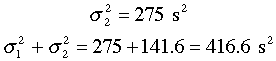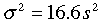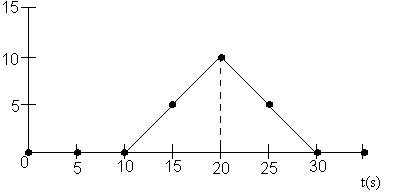
The following data were obtained from a tracer test to a reactor.
|
t(s): |
0 |
5 |
10 |
15 |
20 |
25 |
30 |
35 |
|
Ct(mg/dm3): |
0 |
0 |
0 |
5 |
10 |
5 |
0 |
0 |
1) Plot Ct(t).
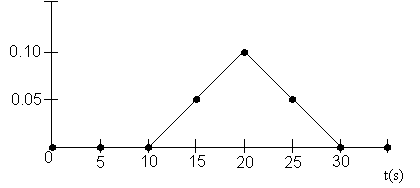
2) Find E(t).
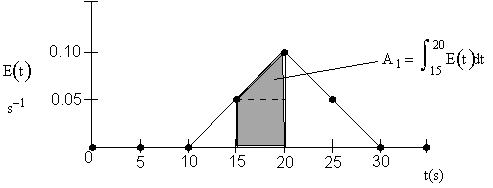
3) Find the fraction of material that spends between 15 and 20 seconds in the reactor.
4) Find F(t) and, the fraction of material that spends 25 seconds or less in the reactor.
5) Evaluate mean residence time.
6) Evaluate the variance.
Hint 1. Plot Ct
Hint 2. Determine E(t)
Hint 3. Find fraction of material spending time between 15 and 20 seconds
Hint 4. Find F(t)
Hint 5. Determining the mean residence time (tm = t)
Hint 6. Calculate the variance" s2
 |
 |
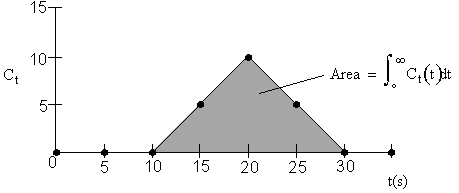

|
t(s): |
0 |
5 |
10 |
15 |
20 |
25 |
30 |
|
Ct(mg/dm3): |
0 |
0 |
0 |
5 |
10 |
5 |
0 |
|
E(t) (s1) |
0 |
0 |
0 |
0.05 |
0.1 |
0.05 |
0 |
 |
 |

Hint 3. Find fraction of material spending time between 15 and 20 seconds
a) Graphical
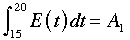 |
= Fractional material spending between time 15 and 20 seconds in reactor |

37.5% of the material spends between 15 and 20 seconds in the reactor
a) Graphical Solution


12.5% of the material spends 15 seconds or less in the reactor.


50% of the material spends 20 seconds or less in the reactor.
What fraction of material spends 25 seconds or less.

87.5% of the material spends less than 25 minutes in the reactor.
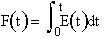
|
t |
0 |
0 |
0 |
0.05 |
0.1 |
0.05 |
0 |
|
F(t) |
0 |
0 |
0 |
0.125 |
0.5 |
0.875 |
1.0 |
b) Analytical Solution
For 0 < 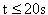
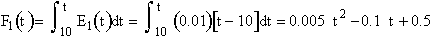
For 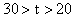
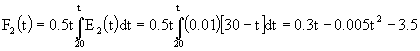

This plot can be obtained from the graphical procedure giving F(t) as a function of time or from the analytical procedure.
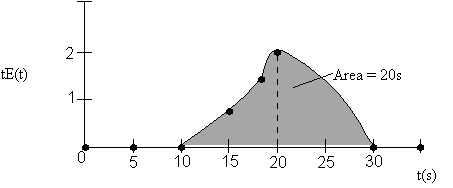
Hint 5. Determining the mean residence time (tm = t)
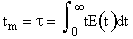
|
t(s) |
1 |
0 |
0 |
0 |
0.025 |
0.05 |
0.075 |
0.1 |
0.075 |
0.05 |
0.025 |
0 |
|
tE(t) |
0 |
0 |
0 |
0.313 |
0.75 |
1.313 |
2 |
1.69 |
1.25 |
0.68 |
0 |
Graphical Solution
b) Analytical Solution
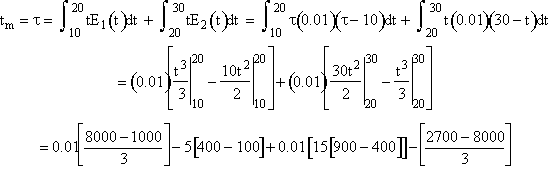
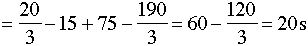
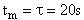
Hint 6. Calculate the variance s2
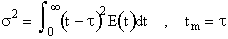
|
t |
E(t) |
(t - t) |
(t - t)2 |
(t - tm2)*E(t) |
|
0 |
0 |
-- |
-- |
0 |
|
10 |
0 |
-- |
-- |
0 |
|
12.5 |
0.025 |
-7.5 |
56.2 |
1.4 |
|
15 |
0.05 |
5 |
25 |
1.25 |
|
17.5 |
0.75 |
2.5 |
5.25 |
.394 |
|
20 |
0.10 |
0 |
100 |
0 |
|
22.5 |
0.075 |
25 |
5.25 |
.394 |
|
25 |
0.05 |
5 |
25 |
1.25 |
|
27.5 |
0.025 |
7.5 |
56.2 |
1.4 |
|
30 |
0.0 |
0 |
a) Graphical Solution

b) Analytical Solution