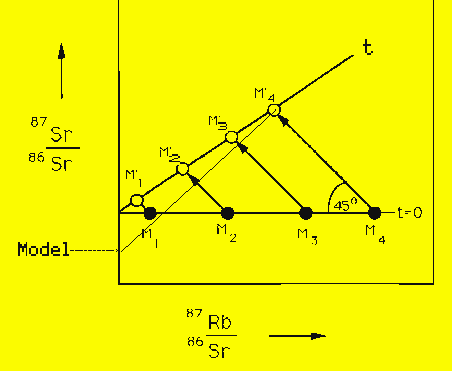
Schematic diagram illustrating dating by the Rubidium-Strontium method. The time t=0 is chosen to be just after the rock initially freezes. We suppose that it contains a number of minerals, designated by M1 through M4. These minerals contain successively more rubidium ions. M4 might be the mineral K-spar, where rubidium can substitute for potassium because of its similar ion size. All rocks are capable of acommodating some amount of rubidium, even when there isn't an obvious substitution. The four minerals M1 through M4 are chosen to reflect this. They don't correspond to any real mineral.
At the moment of freezing, the ratios of 87Sr/86Sr are the same in all four minerals. Then the Rb-87 decreases and the Sr-87 increases. The increase and decrease are by exactly the same amount in each mineral, but the increase-decrease is bigger in the minerals with more Rb-87. At a future time, t, the ratios for each mineral will be on the straight line outlined by the open circles. The slope of this line is a measure of time since the rock froze. If you extrapolate the line back down to the point where it intersects the y-axis, you will get the initial Sr-87 to Sr-86 ratio.
The age obtained in this way, using several minerals, is called
the sample age. The figure also illustrates how the model
age is obtained. In this case only one
87Rb/86Sr value is available, for example, that
for M4. The only way to get an age is to assume an
initial 87Sr/86Sr ratio. In the figure, this
is indicated by the line labeled "Model." The slope of light line
drawn from this point to M4 gives the model age.
Typically, model ages are greater than sample ages because low values
of the initial 87Sr/86Sr ratio are assumed.
In the case of the lunar rocks these initial ratios were taken from
old meteorites.
Click here for other figures.