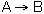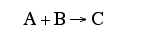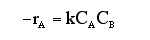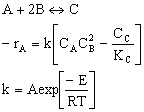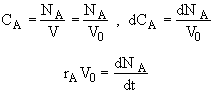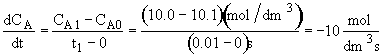Chapter 1 Self Test

What You Have Learned?

1. The rate law

(a.) is a differential equation    T     F

(b.) relates reaction rate and concentration of reacting species     T     F

(c.) is only valid in the U.S.    T     F

(d.) is a function of temperature;   T     F

2. The rate of formation of species A is per unit volume is

(a.) rA    T     F

(b.) -rA    T     F

(c.) the rate of generation of species A per unit volume    T     F

(d.) r'A    T     F

(e.) none of the above    T     F

(f.) not used in Canada    T     F

3.

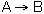
At a particular time t, the rate of formation of B in the reaction, rB, is 10 mole/dm3*min. Which of the following are true?

  1. The rate of disappearance of B is -10 moles/dm3*min.

  2. The rate of formation of A is -10 mole/dm3*min.

  3. The rate of disappearance of A is 10 moles/dm3*min.

  4.  rA = -10 moles/dm3*min

  5. -rA =  10 moles/dm3*min

  6. -rB = -10 moles/dm3*min

  7. Some of the above

  8. All of the above

  9. None of the above

  10. A will disappear faster if a magician is present

Solution  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Solutions

1 (a.) FALSE
F. The rate law is an algebraic equation relating the rate of reaction with the concentration of the reacting species. Example, for the elementary reaction

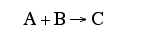
the rate law is:

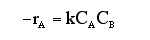
Return to Problem 1
 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

1 (b.) TRUE

-rA = function (temperature and reacting species concentration), e.g.

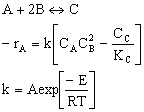
IfDCP = 0, then

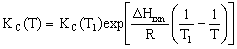
otherwise, see p. 930 of text.

Return to Problem 1  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

1 (c.) FALSE It's also valid in Canada and the northern Provinces

Return to Problem 1  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

1 (d.) TRUE (Page 5 of Text)

Return to Problem 1  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

2 (a.) TRUE (Page 8 of Text)

rA=moles of A formed, per unit time, per unit volume

[rA]~[mol/dm3 s]

Return to Problem 2  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

2 (b.) FALSE (Pages 3 and 5 of Text)

-rA is the rate of disappearance of A, [mol/dm3 s]

Return to Problem 2  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

2 (c.) TRUE

rate of formation=rate of generation

Return to Problem 2  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

2 (d.) FALSE (Page 6 of Text)

r'A is the rate of disappearance of A per mass of catalyst, i.e. grams of catalyst [mol/kg cat s]

Return to Problem 2  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

2 (e.) FALSE (a & c are true)

Return to Problem 2  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

2 (f.) TRUE

The Toronto Maple Leafs Hockey Team has little use for rate of formation of species A.

Return to Problem 2  

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

At a particular time t, the rate of formation of B in the reaction, rB, is 10 mole/dm3*min. Which of the following are true?

  1. The rate of disappearance of B is -10 moles/dm3*min.

  2. The rate of formation of A is -10 mole/dm3*min.

  3. The rate of disappearance of A is 10 moles/dm3*min.

  4.  rA = -10 moles/dm3*min

  5. -rA =  10 moles/dm3*min

  6. -rB = -10 moles/dm3*min

  7. Some of the above

  8. All of the above

  9. None of the above

  10. A will disappear faster if a magician is present

Solution

h. (all are true)
Explanation

Consider a constant volume (V = V0) batch system

Equivalently                

                                                  

                                                                

  :

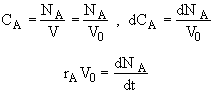
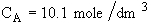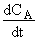At t = 0 then. A moment later at t = 0.01 sec then. If were to be positive, the concentration of A would increase with time. However, A is being consumed so the concentration of A is decreasing with time. For small times we use a different formula to find.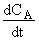

                             

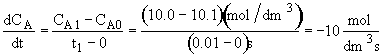
The rate of formation of A is

                                                         

                                                               

                                                               

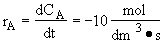
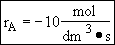
“B” is being formed

                                                                


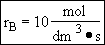
Back to top



Back to Chapter 1