Solution
Gas Phase
Equil Molar Feed
(1)
(2)
Gas, but 
(3)
(4)
(5)
(6)

(7)
(8)
(9)
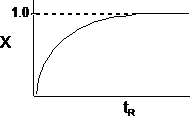
n = number of batches in 300 days
n = 300 days x no. of batch per day
n 
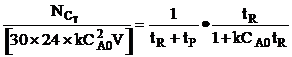
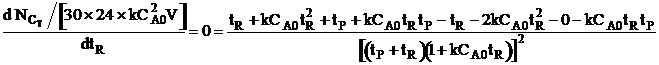
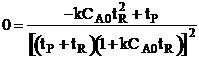

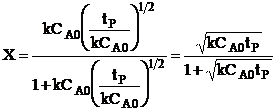
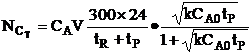


| The following irreversible liquid phase reaction follows an elementary rate law | ||
| A+B → C | ||
| Determine the minimum number of batch reactors 1.0 cubic meter in size to produce 10,000 moles of C in a 300 day period. The processing time to fill, empty and clean the reactor between batches is 4.5 hours. The feed is equal molar in A and B and the initial concentration of A is 1.0 moles per cubic meter. In a trial run it was found that 50% conversion was achieved in 2 hours. | ||
Solution |
||
| Part 1. Find X as a function of t and then find k. | ||
| Batch | ||
| A + B → C | Gas Phase |
Equil Molar Feed |
| Mole Balance | (1) |
|
| Rate Law | (2) |
|
| Stoichiometry | Gas, but |
|
(3) |
||
(4) |
||
| The number of moles C formed in a batch is | ||
(5) |
||
| Combine | (6) |
|
 |
(7) |
|
(8) |
||
| Integrating | ||
(9) |
||
| Evaluate k from information given | ||
| Evaluate | ||
| At t = 2 hr then X = 0.5 | ||
| The conversion for a reaction time tR | ||
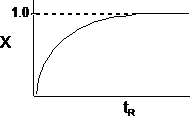 |
||
| Part 2. Find the minimum number of reactors | ||
| The total time for carry out one batch t is the reaction time tR plus the processing time tP which is the sum of the times to empty, te, to clean, tclean, and to fill, tf | ||
| The total processing time, tP, between reactions is tP, = 4.5 hrs. How many 1 m3 reactors do you need to make 10,000 moles of C in 300 days? Consider a single reactor. The number of moles of C formed in one batch | ||
| Total number of C moles made in one reactor for a 24 hour work day over 300 days is | ||
n = number of batches in 300 days |
||
n = 300 days x no. of batch per day |
||
n |
||
| Rearranging | ||
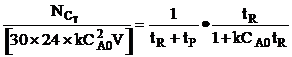 |
||
| For max production rate | ||
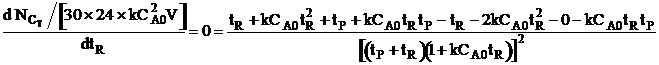 |
||
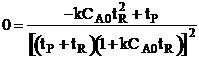 |
||
| Solving for tR | ||
 |
||
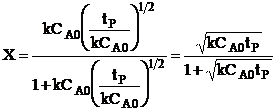 |
||
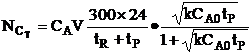 |
||
 |
||
 |
||
| The number of moles 1 reactor can make in 300 days is | ||
| The number of reactors, m, to make 10,000 moles of C in 300 days is | ||