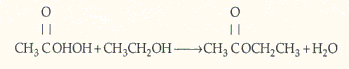
Ethyl acetate is an extensively used solvent and can be formed by the vapor-phase etherification of acetic acid and ethanol.
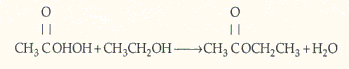
The reaction was studied using a microporous resin as a catalyst in a packed bed reactor [Ind. Eng. Chem. Res., 26(2), 198 (1987)]. The reaction is first order
in ethanol and pseudo zero order in acetic acid. For an equal molar feed rate of acetic acid and ethanol, the specific reaction rate is 1.2 dm3/g cat � min. The total volumetric feed rate is 25 dm3/min, the initial pressure is 10 atm, the temperature is 118�C, and the pressure drop parameter, 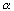 , is 0.01 g�1.
, is 0.01 g�1.
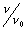 as a function of distance down the packed bed reactor.
as a function of distance down the packed bed reactor.Solutions
A + B → C + D
-rA = kCACB0 = kCA
Isothermal, ε = 0 and P = P0/10
therefore, P = P0(1-αW)1/2 1 = 10(1-0.01 g-1W)1/2 W = 99 g
| M.B | dX/dW = -rA/FA0 | |
| Rate Law | -rA = kCA | |
| Stoich. | CA = CA0(1-X)*P/P0 | |
| P/P0 = (1-αW)1/2 | ||
| CA = CA0(1-X)(1-αW)1/2 | ||
| Integrate from X = 0 to X = 0.9 | ||
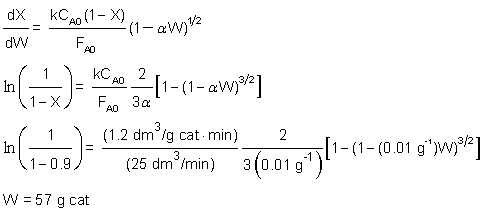
| ||