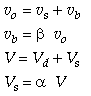Chapter 18: Models for Nonideal Reactors
Topics
| One Parameter Models | top |
A. Tanks-In-Series
A real reactor will be modeled as a number of equally sized tanks-in-series. Each tank behaves as an ideal CSTR. The number of tanks necessary, n (our one parameter), is determined from the E(t) curve.
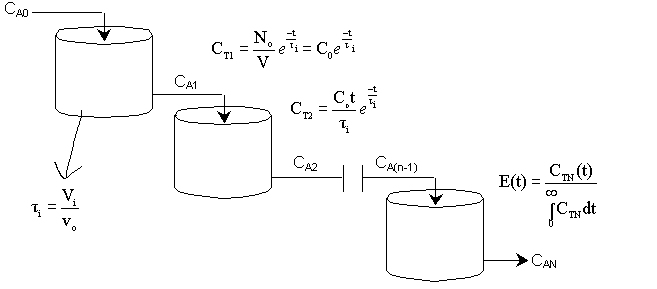
For n tanks in series, E(t) is

It can be shown that

In dimensionless form 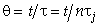

Carrying out the integration for the n tanks-in-series E(t)
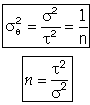
For a first order reaction
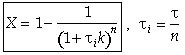
For reactions other than first order and for multiple reactions the sequential equations must be solved
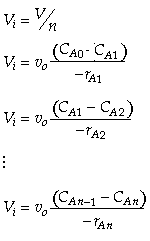
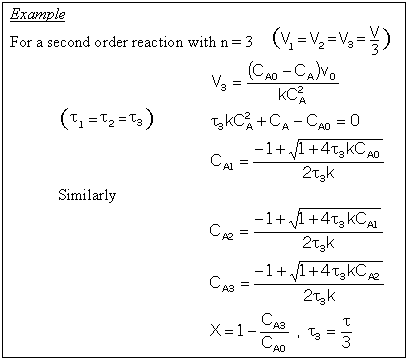
B. Dispersion Model
The one parameter to be determined in the dispersion model is the dispersion coefficient, Da. The dispersion model is used most often for non-ideal tubular reactors. The dispersion coefficient can be found by a pulse tracer experiment.
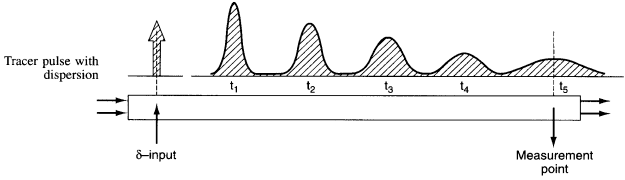
After a very, very narrow pulse of tracer is injected, molecular diffusion (and eddy diffusion in turbulent flow) cause to pulse to widen as the tracer molecules diffuse randomly in all directions. The convective transport equation is:
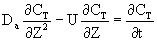
Finding the Dispersion Coefficient
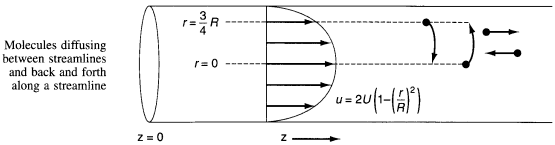
1) For laminar flow Taylor-Aris Dispersion, the molecules diffuse across radial
streamlines as well as axially to disperse the fluid.
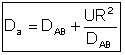
2) To find Da for pipes in turbulent flow see Figure 14-6
3) To find Da for packed bed reactors see Figure 14-7
Using the RTD to find the Dispersion Coefficient.
Results of the tracer test can be used to determine Per from E(t). We need to consider two sets of boundary conditions:
1. Closed-Closed Vessels
2. Open-Open Vessels
For a Closed-Closed Vessel
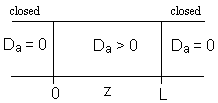
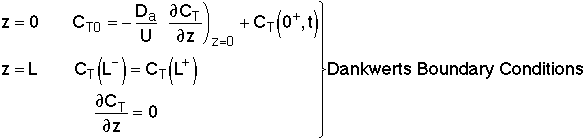
We note there is a discontinuity at the entering boundary in the tracer concentration.
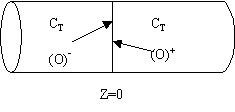
Due to the discontinuity at boundary due to forward diffusion, Ct(O-)=Ct0>Ct(O+)
However, at the end of the reactor, Ct(L-)=Ct(L+)
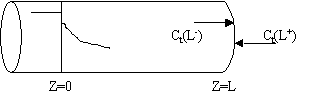
Returning to the unsteady tracer balance
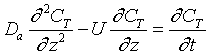 |
(1) |
Let λ = z/L, 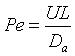 and
and 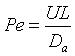
Then in dimensionless form

For the closed-closed boundary condition the solution to the tracer balance at the exit (λ = 1) , at any time Θ, i.e., Ψ(1,Θ) gives
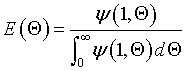
where
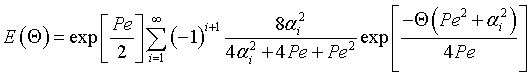 |
(2) |
From page 530 of Froment and Bischoff Chemical Reactor Analysis, 2 nd Edition, John Wiley & Sons, 1966.
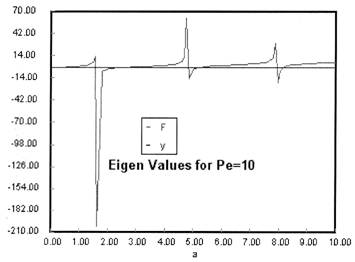
Eigen Values αi are found from the equation
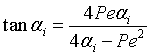
|
F |
|
0 ≤ λ ≤ 1
![]()
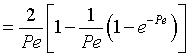 |
(3) |
Integrating Equation (3) using Equation (2) gives
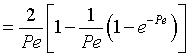 |
(3) |
We see from the equation for E(theta) that the exponential term dies out as
the values of alpha i become large.
then tm and Per is found from the relationship:
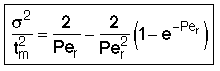

Use RTD data to calculate tm,  2, and then Per (i.e. Da)
and then use Da in calculating conversion.
2, and then Per (i.e. Da)
and then use Da in calculating conversion.
For the solution to 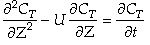 with the above boundary conditions we find
with the above boundary conditions we find

For an Open-Open Vessel
Dispersion occurs upstream, downstream and within the reactor.
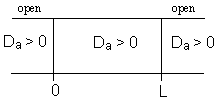
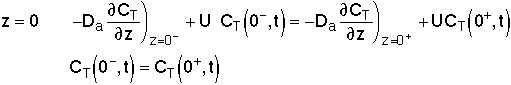

Per>100, for long tubes, the solution at the exit is
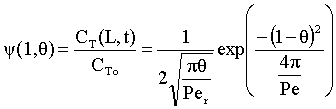
Because of the dispersion the mean residence time is greater than the space time. The molecules can flow out of the reactor and then diffuse back in.
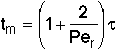

Use RTD data to calculate tm,  2, and then Per (i.e. Da)
and then use Da in calculating conversion. It can be shown that at
steady state, the open-open boundary conditions reduce to the Dankwerts Boundary
Conditions.
2, and then Per (i.e. Da)
and then use Da in calculating conversion. It can be shown that at
steady state, the open-open boundary conditions reduce to the Dankwerts Boundary
Conditions.
Dispersion with Reaction
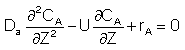
For a First Order Reaction
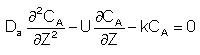
Let Ψ = CA/CA0, λ = z/L , Pe = UL/Da , and Da = kτ. The dimensionless balance on the concentration of A in the reaction zone is
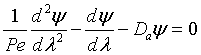
Danckwerts Boundary conditions
At λ = 0 then 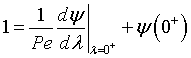
At λ = 1, then 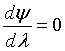
the solution is [See John B. Butt, Reaction Kinetics and Reactor Design, 2nd Edition, page 378, Marcel Dekker, 2000.]
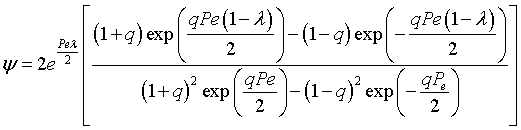
where |
The Polymath program used to plot Ψ versus λ is given below
Polymath Program  |
Sketches of the dimensionless concentration profiles for different values of Peclet and Damköhler numbers are shown below
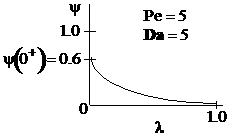


Note how Ψ(0+) changes as Pe and Da change.
The exit conversion is

![]()
The following figures given the Polymath solutions for y versus l for different values of Pe and Da

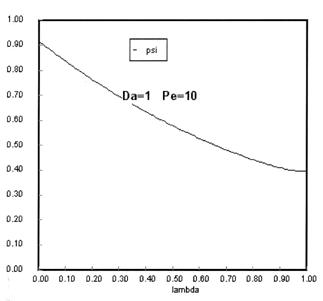

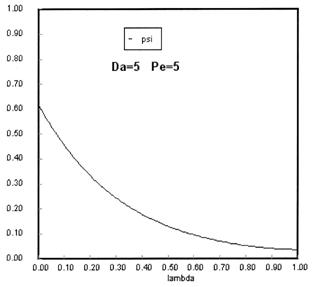
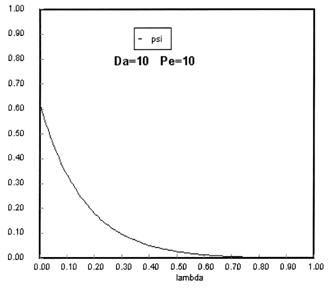
Open Open System
Upstream of the reaction zone the balance on A in dimensionless form is
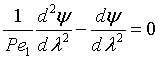
For these boundary conditions
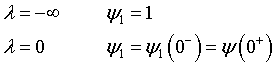
the solution is

Rearranging
A typical profile is
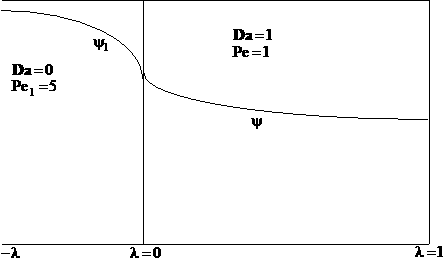
The following profiles were obtained from the Polymath Program given above. Here Ψ1 is the dimensionless concentration of A upstream of the reaction section where Da is greater than zero and Ψ is the dimensionless concentration of A in the reaction section.
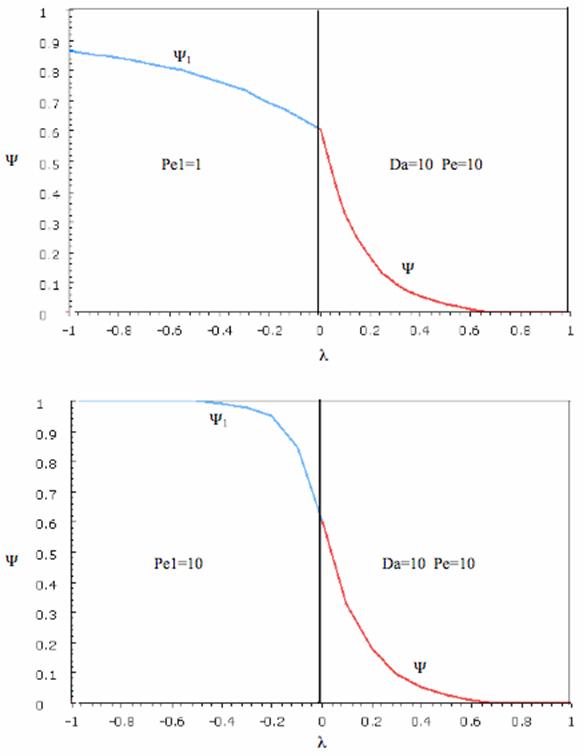
Use RTD data to calculate tm,  2, and then Per (i.e. Da)
and then use Da in calculating conversion.
2, and then Per (i.e. Da)
and then use Da in calculating conversion.
| Two Parameter Models | top |
The goal is to model the real reactor with combinations of ideal reactors.
CSTR with Bypass and Dead Volume
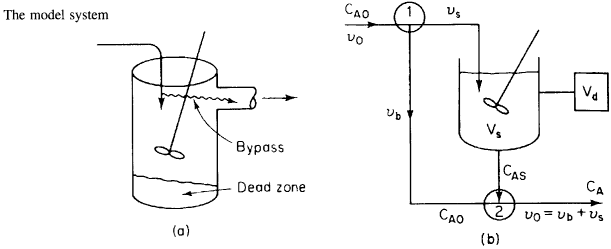
Two parameters ![]() and
and ![]() , the fraction of volume that is well-mixed (alpha),
and the fraction of the stream that is bypassed (beta).
, the fraction of volume that is well-mixed (alpha),
and the fraction of the stream that is bypassed (beta).
Reactor Balance
We now find the parameters a and b from a tracer experiment. We will choose a step tracer input
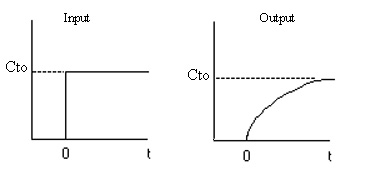
The balance equations are:
OTHER SCHEME INCLUDED
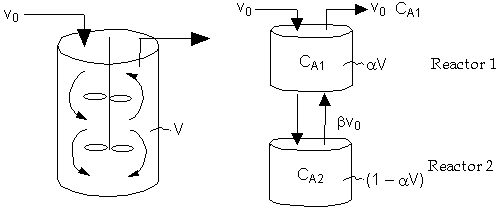
Real Reactor Model of Reactor
Two parameters, ![]() and
and ![]() :
:
- Use the tracer data to find
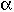 and
and  .
.
- Then use the mole balances and the rate law to solve for CA1
and CA2.
Reactor 1: 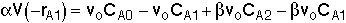
(Eqn. A) Rate Law:  for a first order reaction or
for a first order reaction or
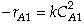 for a second order reaction
for a second order reaction Reactor 2: 
(Eqn. B) Rate Law: 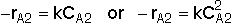
Solve Equations (A) and (B) to obtain CA1 as a function of 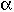 ,
,
 , k,
, k,
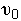 , and CA0.
, and CA0.
Overall Conversion

* All chapter references are for the 4th Edition of the text Elements of Chemical Reaction Engineering .