4. Stoichiometry^*

Rationale For Chapter 4

In chapter 2 we saw that if we had �r_A as a function of X, [�r_A= f(X)] we could size many reactors and reactor sequences and systems.

Topics

1. Batch System Stoichiometric Table
2. Flow System Stoichiometric Table

Stoichiometry

We shall set up Stoichiometric Tables using A as our basis of calculation in the following reaction. We will use the stoichiometric tables to express the concentration as a function of conversion. We will combine C_i = f(X) with the appropriate rate law to obtain -r_A = f(X).

Species	Symbol	Initial	Change	Remaining
A	A
B	B
C	C
D	D
Inert	I	________	-------	____________

Where:

and

Constant Volume Batch:

Note:              if the reaction occurs in the liquid phase                                                or           if a gas phase reaction occurs in a rigid (e.g., steel) batch reactor
Then
	etc.

            if       then

            and we have -ra=f(x)
 

4.1 Writing -r_A soley as a function of X

Species	Symbol	Reactor Feed	Change	Reactor Effluent
A	A
B	B
C	C
D	D
Inert	I	________	-------	____________

Where:

and

etc.

 

If the rate of reaction were -r_A = kC_AC_B then we would have

This gives us -r_A = f(X). Consequently, we can use the methods discussed in Chapter 2 to size a large number of reactors, either alone or in series.

etc. Again, these equations give us information about -rA = f(X), which we can use to size reactors. For example if the gas phase reaction has the rate law  then

with

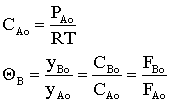
4.1 Rate Law in terms of Partial Pressures

4.2 Stoichiometry Table for Conversion

4.3 Oxidation of Naphthalene to Phtahalic Anhydride

Critical Thinking Questions for the Oxidation of Naphthalene

4.4 Production of Nitric Acid

4.5 Expressing a Catalytic Rate Law in Terms of Conversion

 

Calculating the equilibrium conversion for gas phase reaction

Consider the following elementary reaction with K_C and = 20 dm³/mol and C_A0 = 0.2 mol/dm³. Pure A fed. Calculate the equilibrium conversion, X_e, for both a batch reactor and a flow reactor.

Solution

At equilibrium

Stoichiometry

Batch

Species	Initial	Change	Remaining
A	NA0	-NA0X	NA = NA0(1-X)
B	0	+NA0X/2	NB = NA0X/2
	NT0 = NA0		NT = NA0 - NA0X/2

Constant Volume V = V₀

Solving

Flow

Species	Fed	Change	Remaining
A	FA0	-FA0X	FA = F A0(1-X)
B	0	+FA0X/2	FB = F A0X/2
	FT0 = F A0		FT = F A0 - F A0X/2

  Recall

The following humorous video on limiting reactants was made by Professor Lane's 2008 Chemical Reaction Engineering class at the University of Alabama, Tuscaloosa.

You may access the video by connecting to the internet and clicking the image below

4.2 Old Exam Questions

4.3 Matching C_A versus X curves

4.4 What is wrong with this solution?

Objective Assessment of Chapter 4

^* All chapter references are for the 1st Edition of the text Essentials of Chemical Reaction Engineering .

top