Elements of
Chemical Reaction Engineering
6th Edition



Home

Essentials of
Chemical Reaction Engineering
Second Edition

  Select  Chapter  >>     TOC     Preface     1     2     3     4     5     6     7     8     9     10     11     12     13     14     15     16     17     18     Appendices  
›

By Chapter Hide

  • Objectives
  • Living Example Problems
  • Extra Help
  • - Summary Notes
  • - LearnChemE Screencasts
  • - FAQs
  • - Interactive Computer Modules
  • Additional Material
  • Self Test
  • i>Clicker Questions
  • Professional Reference Shelf

By Concept Hide

  • Interactive Modules
  • -Web Modules
  • -Interactive Computer Games
  • Living Example Problems

U of M Hide

  • Asynchronous Learning
  • ChE 344
  • ChE 528

Chapter 2: Conversion and Reactor Sizing

Topics

  1. Conversion
  2. Design Equations
  3. Reactor Sizing
  4. Numerical Evaluation of Integrals
  5. Reactors in Series
  6. Space Time
  7. Useful Links
Conversion top

Consider the general equation

The basis of calculation is always the limiting reactant. We will choose A as our basis of calculation and divide through by the stoichiometric coefficient to put everything on the basis of "per mole of A".

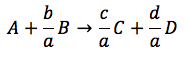
The conversion X of species A in a reaction is equal to the number of moles of A reacted per mole of A fed.

Batch Flow
Sorry, your browser does not support the audio tag. Please try using a different browser or updating your current browser.

What is the maximum value of conversion?

    For irreversible reactions, the maximum value of conversion, X, is that for complete conversion, i.e. X=1.0.

    For reversible reactions, the maximum value of conversion, X, is the equilibrium conversion, i.e. X=Xe.


Batch Flow
Moles A remaining = NA = Moles A initially - Moles A reacted Rate of Moles of A leaving FA = Rate of Moles of A fed - Rate of Moles of A reacted
NA= NA0 - moles A initially * (moles A reacted)/(moles A fed) FA= FA0 - Rate of moles A fed * (moles A reacted)/(moles A fed)

Design Equations top

The design equations presented in Chapter 1 can also be written in terms of conversion. The following design equations are for single reactions only. Design equations for multiple reactions will be discussed later.

Reactor Mole Balances in Terms of Conversion (Click on Reactor to see picture)

Sorry, your browser does not support the audio tag. Please try using a different browser or updating your current browser.
Reactor Differential Algebraic Integral    
Batch  
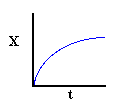
CSTR      
PFR    
PBR  

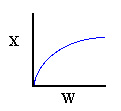
Reactor Sizing top

By sizing a chemical reactor we mean we're either detering the reactor volume to achieve a given conversion or determine the conversion that can be achieved in a given reactor type and size. Here we will assume that we will be given -rA= f(X) and FA0. In chapter 3 we show how to find -rA= f(X).

Given -rA as a function of conversion,-rA=f(X), one can size any type of reactor. We do this by constructing a Levenspiel plot. Here we plot either or as a function of X. For vs. X, the volume of a CSTR and the volume of a PFR can be represented as the shaded areas in the Levenspiel Plots shown below:




Using the Ideal Gas Law to Calculate CA0 and FA0
Levenspiel plots in terms of concentrations

Numerical Evaluation of Integrals top

The integral to calculate the PFR volume can be evaluated using a method such as Simpson's One-Third Rule:

NOTE: The intervals ( ) shown in the sketch are not drawn to scale. They should be equal.

Simpson's One-Third Rule (above) is one of the more common numerical methods. It uses three data points. Other numerical methods (see Appendix A) for evaluating integrals are:

  1. Trapezoidal Rule (uses two data points)
  2. Simpson's Three-Eighth's Rule (uses four data points)
  3. Five-Point Quadrature Formula
Reactors in Series top

Sorry, your browser does not support the audio tag. Please try using a different browser or updating your current browser.

Given -rA as a function of conversion, one can also design any sequence of reactors by defining the total conversion up to a point "i":

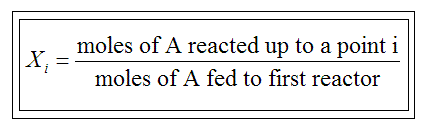
Only valid if there are no side streams

Consider a PFR between two CSTRs

Sorry, your browser does not support the audio tag. Please try using a different browser or updating your current browser.

Use Levenspiel plots to calculate conversion from known reactor volumes
One of the goals of the course is to have the readers further develop their critical thinking skills. One way to achieve this goal is through Socratic questioning. Throughout the course students will be asked to write questions on critical thinking drawing from information the Preface section B2. Below are some examples of critical thinking questions (CTQ) that are either superficial or don't use R. W. Paul's Six Types of Socratic Questioning
Reactors in Series CSTR-PFR-CSTR
What if ...?

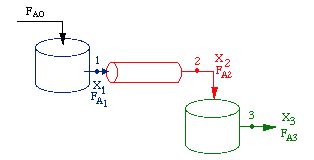
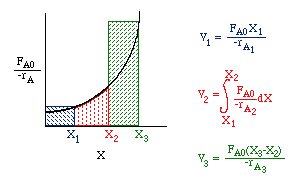
Space Time top
The Space time, tau, is obtained by dividing the reactor volume by the volumetric flow rate entering the reactor:

Space time is the time necessary to process one volume of reactor fluid at the entrance conditions. This is the time it takes for the amount of fluid that takes up the entire volume of the reactor to either completely enter or completely exit the reactor.

Sample Industrial Space Times
  Reaction  
  Reactor    Temperature  Pressure atm    Space Time  
(1) C2H6 → C2H4 + H2
 PFR 860°C 2 1 s
(2) CH3CH2OH + HCH3COOH → CH3CH2COOCH3 + H2O
 CSTR 100°C 1 2 h
(3) Catalytic cracking PBR 490°C 20 1 s < τ < 400 s
(4) C6H5CH2CH3 → C6H5CH = CH2 + H2 PBR 600°C 1 0.2 s
(5) CO + H2O → CO2 + H2 PBR 300°C 26 4.5 s
(6) C6H6 + HNO3 → C6H5NO2 + H2O CSTR 50°C 1 20 min
Useful Links top
Numerical Evaluation of an Integral
Old exam questions
What's wrong with this solution?
What is more efficient?
Hippo Web Module
Access your learning style.
How the website can help your learning style.
More on learning styles.
Objective Assessment of Chapter 2
 

* All chapter references are for the 1st Edition of the text Essentials of Chemical Reaction Engineering.

top