 for frequency, and
for frequency, and 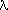 for wavelength. The symbol `c' is used for the velocity of
light, thus:
for wavelength. The symbol `c' is used for the velocity of
light, thus:
Until the development of the space program, virtually all of the astronomers' information came in the form of light from distant objects. It is natural that they would have played an important role in the investigations of the nature of light, just as they did in the development of Newton's mechanics. Many of the pioneers in the development of the modern theory of light were keenly interested in astronomical problems.
The true nature of light wasn't really understood until the 20th century. Experiments done in the 19th century indicated that light was a form of wave motion. For many purposes, it is sufficient to describe light as a combined electrical and magnetic wave, or an electromagnetic wave.
In the decade following 1964, the great English physicist James Clerk Maxwell was able to describe all electrical and magnetic phenomena with the help of four differential equations. They are now called Maxwell's equations, and every student of physics must learn to work with them.
It's quite amazing that phenomena as varied as starlight, and children's magnets could be described by four relatively short equations, but this is the case.
Perhaps the simplest way to think of these waves is to first picture ``lines of force'' about an electrical charge. Nearly everyone has seen the lines of force about the poles of a magnet demonstrated with the help of iron filings and a glass plate. The concept of lines of force arose as a way of eliminating the problem that arose with the attraction of two bodies separated by some distance.
It's easy enough to understand how something will move if you grab it and push or pull. On the other hand, how can two bodies separated by "nothing" attract or repel one another? This difficulty is known as the problem of action at a distance. It can be solved, after a fashion, with the notion of lines of force. Think of electrical charges or magnetic poles as being surrounded by lines of force, like those demonstrated for a bar magnetic. Then the lines of force fill in the void between the bodies. They will grab the other body, and there is no more action at a distance.
Maxwell's equations described these lines of electrical or magnetic force. They showed that if you accelerated a charge, for example, if you wiggled it up and down, a wave would run out the electrical line of force.
The same equations showed that the electrical wave would have to be accompanied by a magnetic wave. That's a little hard to see, and we won't go into it here. Take our word for it. But the electrical wave can be pictured as something similar to the wave that would travel down a rope. Stretch a rope out horizontally, and wiggle one end of it, and a wave will run down the rope away from your hand. Pretend that you fasten the far end way away, so you don't have to worry about what happens when the wave gets to that end.
The electromagnetic wave is a form of light. It turns out that light is most conveniently described as a wave when the wavelength is relatively long, say of the order of a centimeter or more. Radio waves can be tens or even hundreds of meters in wavelength.
All wave motion travels with a velocity equal to it's frequency
(units are per second, or sec-1) multiplied by its
wavelength. Astronomers traditionally use the Greek letter
 for frequency, and
for frequency, and 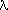 for wavelength. The symbol `c' is used for the velocity of
light, thus:
for wavelength. The symbol `c' is used for the velocity of
light, thus:

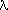 = c
= c
When the wavelength of light is shorter, a millimeter or less, say, a rather different picture is better--the photon picture.
According to the quantum theory, the energy in electromagnetic waves comes in little bundles that are called photons. The shorter the wavelength, the better it is to think of the photon as a kind of particle--rather than a wave. It isn't that the wave picture becomes invalid, it's just that for many purposes, it's better to think in terms of photons.
Light, X-rays, 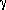 -rays, and radio waves are thus all forms of the
same phenomena--electromagnetic radiation.
-rays, and radio waves are thus all forms of the
same phenomena--electromagnetic radiation.
In 1900, the German physicist Max Planck was trying to understand the nature of radiation that filled an enclosure that had come to equilibrium. When temperature within the enclosure was the same everywhere, people measured the amount of energy in the radiation as a function of wavelength. Their results could be displayed as a kind of histogram, with energy plotted against small wavelength intervals. What they found was quite surprising. The shapes of the curves depended only on the temperature of the enclosure, and not on the material inside. Planck himself made many of these measurements, and the energy distribution became known as the universal Planck radiation law.
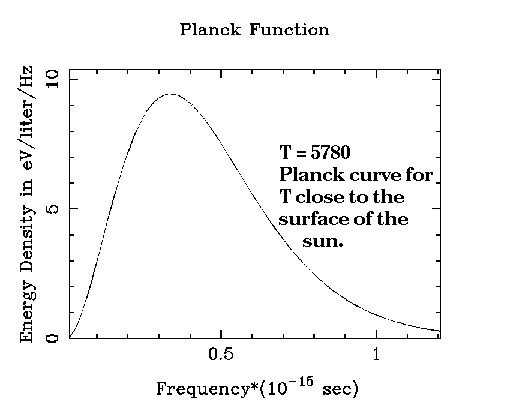
The German word for this kind of a distribution of electromagnetic radiation literally means "radiation from a cavity." The equivalent English term is black body radiation. One can blacken a surface with paint, or better, with soot, and it will emit radiation that is (very nearly) in the form of Planck's universal law.
Planck found that he could account for this law with the help of
Maxwell theory provided he assumed that the energy in the radiation
came in multiples of the frequency: E = h .
The constant quantity `h' is now known as Planck's constant, and it is one
of the three fundamental constants of nature. The other two are the
constant of gravitation G, and the velocity of light, c.
.
The constant quantity `h' is now known as Planck's constant, and it is one
of the three fundamental constants of nature. The other two are the
constant of gravitation G, and the velocity of light, c.
The most energetic forms of electromagnetic radiation are called
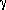 -rays. These photons are characteristic of the emissions of
atomic nuclei, and have energies of millions of electron volts
(MeV). X-rays are slightly less energetic, with energies of
thousands of electron volts, kilo-electron volts, or KeV. The
photons of visible light have energies of the order of a few electron
volts (eV).
-rays. These photons are characteristic of the emissions of
atomic nuclei, and have energies of millions of electron volts
(MeV). X-rays are slightly less energetic, with energies of
thousands of electron volts, kilo-electron volts, or KeV. The
photons of visible light have energies of the order of a few electron
volts (eV).
Name Energy A Characteristic Wavelength ---- ------ ---------------------------- Gamma rays Mev 0.01 Angstroms X-rays KeV 1 Angstrom visible light 0.2 eV 5000 Angstroms infrared light 0.003 cm microwaves 1 cm radio waves 100 meter
There are other forms of "radiation" that are not electromagnetic.
The famous "rays" of radioactive material were called  ,
,
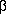 ,
and
,
and 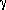 . Of these, only
. Of these, only  rays are electromagnetic. The
rays are electromagnetic. The
 and
and  rays are helium nuclei
and electrons, respectively.
rays are helium nuclei
and electrons, respectively.
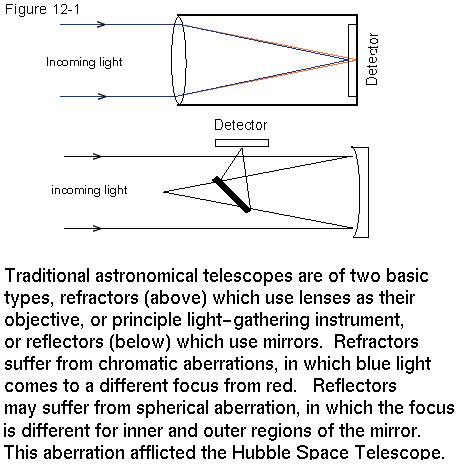
The first astronomical telescopes were made by Galileo. They used a lens and an eyepiece. Such telescopes are called refractors because the light is bent by these lenses. The bending of light is called refraction. Galileo's eyepieces allowed him to see the images right side up, just as binoculars do. It turns out to be a little more efficient if one can live with an upside down image. The traditional astronomical telescope inverts the image. This is not a disadvantage when viewing celestial objects, for which we may have no preconceived notion of how they should be oriented.
For many years, astronomers made upside down maps of the Moon, because that was the way the Moon appeared in their telescopes. Even in recent textbooks on astronomy, one can see photographs of the Moon that are upside down. Planetary astronomers prefer to have the north at the top of a map, however, and gradually most lunar maps have appeared in that orientation.
The largest refracting telescope is the 40" at the Yerkes Observatory of the University of Chicago. It, and the Lick 36" were built at the end of the 19th century. Early in the 20th century these instruments were surpassed by large reflecting telescopes. The 60" reflector Mount Wilson went into operation on Mount Wilson, California in 1908, followed by the 100" reflector in 1917. The latter was the world's largest telescope until the 200" reflector was completed at mid-century, and placed on Palomar Mountain. As our century ends, numerous larger telescopes have gone into operation. Currently the largest are the twin Keck telescopes in Hawaii, which have the light-gathering equivalent of 10 meter telescopes.
Telescopes have 3 roles or functions
 is the ratio
is the ratio
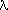 /D, where D
is the diameter of the primary. This ratio comes out in radians, and is
often converted to seconds of arc. There are 206265 seconds of arc in a
radian. Higher resolving power traditionally means the ability to
see objects separated by a smaller angle.
/D, where D
is the diameter of the primary. This ratio comes out in radians, and is
often converted to seconds of arc. There are 206265 seconds of arc in a
radian. Higher resolving power traditionally means the ability to
see objects separated by a smaller angle.
Telescopes may be characterized by 2 numbers, the diameter of the primary (mirror or lens) and the focal length of the primary. The focal length is the distance from the primary at which an image is formed by light rays coming from infinity.
All of the newer major telescopes are reflectors, because it is possible to make them bigger than lenses. The current new telescopes are of the order of 8 meters in diameter. By putting many mirrors together, the effective aperture has been increased to 10 meters. Reflectors are free from chromatic aberration, which distorts images formed by lenses. If a telescope used a simple lens, the blue light would come to a focus before (closer to the lens) than the red.
Mirrors have distortions too. In particular, light rays near the axis of the mirror may not come to the same focus as rays near the edge. This effect is called spherical aberration. It was the problem that affected the Hubble Space telescope.
Our Earth's atmosphere is transparent to light only from about 3200 to 10,000 Angstrom units. One Angstrom is 10-8 cm. Shorter and longer wavelengths are blocked by various absorbers. At much longer wavelengths, there is an important radio window, from about a centimeter to perhaps 10 meters.
The atmosphere not only absorbs radiation at wavelengths astronomers would like to observe at, it also distorts images--the twinkle of stars results in a distortion. So space observations are important, and the Hubble Space Telescope has been making many important discoveries.
The outer rim of the Hubble primary is too low, giving rise to spherical aberration. After three years of sub-standard performance, a servicing mission installed corrective optics, and the instrument is now performing to expectations.
Photography came into its own in the latter half of the 19th century. All of the early astronomers before this time were visual observers. They had to record their observations in the form of sketches, and some of them got to be pretty fanciful. The Earth's atmosphere distorts the images of stars and planets, so in a very real sense, observers couldn't really see the same things.
The problem came to a head in the case of observations of Mars, where the American astronomer Percival Lowell claimed to see elaborate markings, which he interpreted as artifacts from an advanced civilization. Other astronomers saw nothing like the amount of detail in Lowell's sketches.
Photographic plates of Mars showed even less detail than visual observers could see. This is because the plates were essentially long exposures; the flickering images of the planet were blurred. Visual observers could wait for moments of exceptional "seeing," and it was at those times that Lowell claimed to see things no one else could.
For most of the 20th century, the traditional astronomical detectors were photographic plates. They were widely used in astronomy toward the end of the last century, continuing to well past the middle of the current one. Quite wide fields may be photographed, and this is still one of the major strengths of astronomical photography. However, only about 1 to 2% of the light falling on a photographic plate is detected. This drawback not present with modern electronic detectors; the most common is called the CCD, or charge coupled device.
CCD's were invented at Bell Labs in the mid 1970's. Unlike photographic plates, they detect 90% or more of the light that falls on them. However, they are still small in size, the largest ones being about 2 inches on a side. They are also expensive.
CCD's operate on the basis of the interaction of light photons and silicon crystals. Photons in a certain wavelength interval are capable of freeing electrons within the crystal so they can be made to move as in ordinary electrical current. The freed electrons are maintained in the original locations by electric fields generated as a part of the detector. These fields are said to generate potential wells, which we may conceptually think of as little bowls in which the electrons collect. The more electrons, the more light photons that hit the silicon. It is possible to localize these potential wells in to very small areas, so that the resolution of modern CCD's approaches that of photographic plates--10 to 20 microns (1 micron is 10-4cm).
After a time, the charge in the wells represents a digitized version of the image. It is then possible to manipulate the wells in such a way that the charge in each of them may be measured. In essence, the charge (or number of electrons) in each well is read one by one, in such a way that the location of the charges is remembered. The image may then be reconstructed by a computer. To do this by hand would take quite a while. A modern CCD might contain 20 Megabytes of data!
With a photographic plate, or with a CCD, the most elementary technique is called imaging--just a fancy way to say "taking a picture." Images of star fields were traditionally measured carefully for the positions of stars, to reveal their proper motions or parallaxes. The images of extended objects, such as bright or dark gas clouds, or of external galaxies would simply be examined and their characteristics noted. Photographic images of galaxies led Edwin Hubble to his famous tuning-fork classification scheme. Extensive imaging from space probes have been used to advance our knowledge of the solar system. Even before the lunar missions, planetary geologists had begun to map the surface of the Moon using techniques that had been developed by terrestrial geologists. We had to wait for space probes before the same methods could be applied to the planets. Telescopic images of Mars, both visual and photographic, had led to more confusion than enlightenment. Mariner and Viking images of Mars in the 1970's, revealed a surface intermediate in complexity between those of the Earth and Moon.
It was known to Isaac Newton that if sunlight was passed through a polished glass prism, it would be dispersed into a rainbow of colors. This is illustrated in Figure 13-2
During the 19th century, physicists learned how to use this technique to identify chemical elements in a laboratory sample. At the same time, similar methods were employed as a means to analyze the atmospheres of the sun and the stars. If the dispersed light is examined with the eye, the instrument is called a spectroscope. If that light is first recorded with a photographic plate, or electronic detector, the term spectrograph is used. The light itself, after dispersion, is called the spectrum of the object that emitted it. The plural of spectrum is spectra.
It was already recognized that the nature of the spectrum of an object depended on physical conditions. Gustav Kirchhoff's laws of spectroscopy are valid today:
It was soon realized that one could identify the chemical element by its bright or dark spectral lines. There is a story that Kirchhoff once told his banker that he had identified the chemical element gold in the spectrum of the sun. The banker was unimpressed, because there was no way to mine that gold. Later, Kirchhoff was awarded a gold medal from the British Royal society, along with some gold coins. He is said to have returned to his banker, saying "this is gold from the sun."
Modern spectrographs generally employ gratings rather than prisms as dispersing elements. Gratings can be used either in transmission or reflection. In the latter case, a mirror is closely ruled with parallel scratches. The light that bounces off such a surface is dispersed into little rainbows on either side of a central image, called orders. It has become possible to make these scratches so that most of the light is thrown into one of these orders. Such a grating is said to be blazed, and can be a very efficient dispersing element.
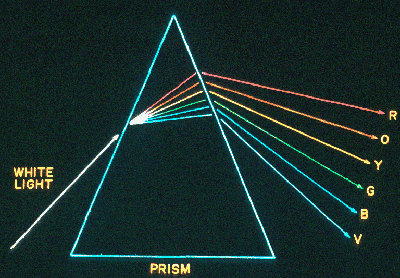
Atoms, electrons, and nuclei no longer obey Newton's "classical" laws. The modifications are not intuitive. You have to learn the rules, and that takes some effort. For our purposes, we can examine the case of a very simple system, a hydrogen atom consisting of an electron and a proton. The two relevant charges, are the same in this case, and the attractive force is is an inverse square one, just as for gravitation. The potential energy curve is proportional to 1/r, and looks just like the one for the two-body problem [Figure 11-2(b)].
Quantum rules prevent the electron from being at any (old) distance from the proton. If we drop the electron straight at the proton, then there are a series of distances where the electron will "hang" on its way down the well. It is the wave nature of the electron that makes it "hang" at certain depths in the potential well. It turns out that when you examine the wave nature of the electron, its wave has just one loop at the lowest possible energy level, 2 loops at the next one up, and so on. The picture is a little more complicated if we add angular momentum, but we don't need that here. There must be an integral number of waves in the well or the corresponding energy isn't allowed. This is why the energies are said to be quantized.
On the way to higher energies, the allowed levels crowd closer and closer together.
Electrons can get from one level to another by emitting or
absorbing a photon of just the right energy. This is an example
of the famous quantum jump. For a jump down, a photon
must be emitted, and for a jump up, one must be absorbed. These
photons are created when the electron jumps down, and are destroyed
when it jumps up. So the energy of the photon, h must be
just equal to the difference in the energies of the two levels
involved in the jumping. If we have two allowed energies,
En and Em > En, then the frequency of a photon,
must be
just equal to the difference in the energies of the two levels
involved in the jumping. If we have two allowed energies,
En and Em > En, then the frequency of a photon,
 that will be emitted will be given by the relation
that will be emitted will be given by the relation

If a photon of frequency  =
Em-En/h strikes
an atom in the lower level n, the atom may absorb the photon, and jump
to the level m.
=
Em-En/h strikes
an atom in the lower level n, the atom may absorb the photon, and jump
to the level m.
We can understand the Kirchhoff laws with the help of this quantum picture. First [see K1 above], we get a continuum when the energy levels get very close together. This can happen in solids (like a tungsten filament) and liquids. When the atoms are very near one another, the energy levels get smeared out and their average values can also get close together. This will also happen to a gas under high pressure, such as the visible photosphere of the sun.
When [K3] a continuous spectrum shines through a cool, low pressure gas, the atoms in the gas selectively absorb out the discrete wavelengths determined by their special energy levels: E1, E2, E3, etc.
If a hot gas is observed without a continuous source behind it, some fraction of the atoms in this gas will always be in higher energy levels. They can get into these upper levels in a variety of ways. They can bump into one another, and get boosted to higher internal energy states. What happens in that some of the kinetic energy of motion in transformed into internal energy. Also the hot gas may absorb occasional photons from some other source. These photons may boost the electrons to upper levels, or even ionize the atoms. Eventually, the electrons will recombine and/or just jump to lower energy levels, with the emission of a photon. When these photons are observed, they show a bright-line, or emission spectrum [K2].
Radio telescopes function much like optical ones. Their primaries
are often parabolic reflectors, but much larger. The same formula,
 /D holds for their resolving power, but it is
much lower than for
optical telescopes. This is because the wavelength of
radio waves is many orders of magnitude greater than optical light, and
the large primaries do not compensate. So
/D holds for their resolving power, but it is
much lower than for
optical telescopes. This is because the wavelength of
radio waves is many orders of magnitude greater than optical light, and
the large primaries do not compensate. So 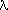 /D
is generally much
bigger for a radio telescope than an optical one. Radio astronomers
compensate for this by combining observations from telescopes separated
by large distances--sometimes thousands of miles. The technique is
called interferometry, and by using it, radio instruments can
resolve down to 0.0001 seconds of arc. This is now much smaller than
the resolution of optical telescopes!
/D
is generally much
bigger for a radio telescope than an optical one. Radio astronomers
compensate for this by combining observations from telescopes separated
by large distances--sometimes thousands of miles. The technique is
called interferometry, and by using it, radio instruments can
resolve down to 0.0001 seconds of arc. This is now much smaller than
the resolution of optical telescopes!
The large, often parabolic receivers that one sees in pictures of radio telescopes function rather like the antennae of ordinary radios. The electromagnetic waves from space are made to drive electrical currents which are then subject to ingeneius and powerful amplification. You can often read about radio astronomers "listening" to sounds from space. The signals received are electromagnetic, and not acoustical. The popular notion of listening comes by analogy to "listening" to an ordinary radio. The electrical currents can be made audible with the help of speakers. Often, radio astronomers employ speakers, and do listen to their sounds. Unlike the sounds from an ordinary radio, the sounds from speakers at a radio telescope mostly resemble static.
Astronomers are keenly interested in signals from deep space that
are carried by high energy photons, X-rays and 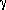 -rays.
They have built a variety of special instruments to detect these
photons, which we shall only mention briefly. X-ray telescopes
can resemble optical telescopes in that the light may be brought
to a focus and analyzed. Depending on the energy of the
-rays.
They have built a variety of special instruments to detect these
photons, which we shall only mention briefly. X-ray telescopes
can resemble optical telescopes in that the light may be brought
to a focus and analyzed. Depending on the energy of the
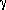 -rays,
these telescopes make use of the interaction between the
-rays,
these telescopes make use of the interaction between the
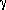 -rays
and atoms or nuclei. The most energetic
-rays
and atoms or nuclei. The most energetic 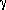 -rays
cause showers
of secondary particles to form in the atmosphere. These may be detected
at ground level with specialized instrumentation that need not concern
us in this course.
-rays
cause showers
of secondary particles to form in the atmosphere. These may be detected
at ground level with specialized instrumentation that need not concern
us in this course.
In planetary astronomy, this region of the electromagnetic spectrum is chiefly employed in the overall technique called remote sensing, which we will discuss in detail later in the course.
Electromagnetic radiation comes in many forms. In order of increasing
wavelength, and decreasing energy and frequency, we have:
 -rays, X-rays, visible light, infrared radiation,
and radio waves. Astronomers traditionally used optical telescopes,
first refractors, and then reflectors to catch visible starlight. With
the help of satellites and radio telescopes they now investigate the
entire electromagnetic spectrum. The light-gathering and resolving
power of a telescope depends on the aperture (size) and in the latter
case also the wavelength of the light received. Radio telescopes
receive and amplify cosmic radiation at long wavelengths. The
light-gathering and resolving power of these instruments are the
same as for optical telescopes.
-rays, X-rays, visible light, infrared radiation,
and radio waves. Astronomers traditionally used optical telescopes,
first refractors, and then reflectors to catch visible starlight. With
the help of satellites and radio telescopes they now investigate the
entire electromagnetic spectrum. The light-gathering and resolving
power of a telescope depends on the aperture (size) and in the latter
case also the wavelength of the light received. Radio telescopes
receive and amplify cosmic radiation at long wavelengths. The
light-gathering and resolving power of these instruments are the
same as for optical telescopes.
Light is analyzed with the help of spectrographs. Astronomers have used both prisms and gratings to disperse light. Each atom and ion has a set of unique energy levels. Transitions or quantum jumps among these levels give rise to the emission or absorption of photons. Kirchhoff's laws describe the conditions for continuous, absorption, or emission spectra. Chemical elements can be identified by measuring the wavelengths of light that are emitted or absorbed by some source.

What does it mean to analyze cosmic materials? What information would we have after such an analysis that we didn't have before?
There are a variety of ways cosmic materials may be analyzed. The simplest kind of question we might ask is for the number of atoms of each chemical element in the sample. So we can think of the periodic table, and having a number for each element. A chemist would think in terms of the number of "moles" rather than numbers of atoms, but if we know Avogadro's number (Na) we can give the information either way.
If we take a sample and divide it into several parts, the number of atoms in each part will be different, in general. If the sample is uniform--not different in one place than another--the relative numbers of atoms in each of the divided sample will be the same. Cosmochemists almost always work with relative numbers of atoms, rather than absolute numbers.
In the analysis of terrestrial and moon rocks, or meteorites the element silicon is usually taken as a standard, and numbers of atoms of other elements are given relative to the number of silicon atoms. A standard ploy is to quote the number of atoms of elements relative to a million silicon atoms.
Geochemists or cosmochemists will commonly speak of abundances of the elements in some sample. They almost always mean by `abundances' the relative number of atoms or moles of some element to the number of atoms or moles of silicon. Since we are taking ratios we are free to use either atoms or moles, since a possible Avogadro's number would cancel from numerator and denominator of the fraction.
Analyses by number of atoms are very useful when it comes to investigating isotopes. Isotopic abundances are essential for radioactive dating of cosmic materials as well as for determining their history. It has been found that the relative abundances of the three stable oxygen isotopes, 16O, 17O, and 18O are characteristic of different portions of the solar system. Since lunar rocks show the same isotopic abundances as terrestrial, we must conclude that the lunar impactor (Big Whack) must have originated from very nearly the same regions of the primitive solar system as the Earth.
An analysis by number of atoms alone obscures much of the history of cosmic materials. All of the chemistry, all of the processes that caused the atoms to fall into the potential wells we call chemical bonds, is lost. It is therefore also necessary to analyze samples in such a way that as much of this chemistry as possible is revealed. In the case of moon rocks and meteorites, much of the relevant chemistry is essentially mineralogy. We will get to mineralogy in detail in the next lecture. Here, we will discuss methods used to determine relative amounts of minerals in a sample.
For more than a hundred years the typical technique for the chemical analysis of a sample involved dissolution in acids. The solutions might then be treated with reagents (chemicals) to produce an insoluble precipitate which would then be separated and weighed. Today's techniques often involve dissolution in acids, but usually as a first step to a variety of methods quite different in nature from those that ended with measurements being made on a chemical balance.
We will discuss some of these methods in turn, starting with an instrument called a mass spectrograph.
Mass spectrometry may be the most powerful technique of modern cosmochemical analysis. The basic instrument was invented in 1919 by the British chemist and physicist F. W. Aston.
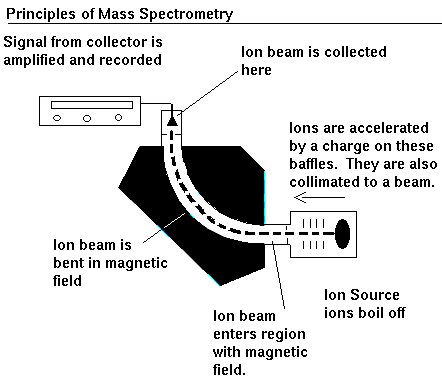
A standard mass spectrograph would work in the following way. First, the sample would be vaporized. This might be done by simple heating, or the sample might be dissolved in acid first, and then vaporized. The chemical treatment would have to be carefully chosen not to interfere with the analysis. The vaporized atoms or molecules would then be ionized. There are a variety of ways to do this, both chemical and physical. A simple method is to bombard the sample with electrons, perhaps from a hot filament.
The ions in the sample are then accelerated in an electric field, and collimated into a beam, often with simple mechanical baffles or other means, called ``ion optics.'' The beam then passes into a region with a magnetic field.
It is one of the fundamental laws of electromagnetism, that a moving, charged particle is acted upon by a force. The force depends on the velocity and the electrical charge, which we shall call q. The direction of the force is perpendicular to both the direction of the field and the velocity. This means if the velocity is in the x-direction, and the field in the y-direction, the force is in the z-direction. Complicated, but that's the way it is.
By Newton's second law, the acceleration (vector), which determines the trajectory, then depends on the ratio of the charge to the mass:
The ion beam is therefore bent in a curved path. The ions are eventually collected in a detector, and made to generate an electrical current that can be measured. Ions with different m are accelerated differently and so follow slightly different paths. If multiple collectors are available, the relative numbers of ions with different paths is immediately determined by the difference in electrical currents generated in the collectors.
With one collector, only one kind of ion can be measured at a time. However, it is possible to change the strength of the magnetic field in such a way that a new ion, with a different m, will enter the detector. The relative numbers of ions can be determined as before, by the different electrical currents generated.
It is also possible to tell the differences in the masses of particles in the beam by the magnetic field strength change that was necessary to make the new ions enter the detector.
Since F is directly proportional to the charge, q, the mass spectrograph strictly measures the ratio, q/m. If we can be sure that the charges are the same on all of the ions, one may determine the mass of particles entering a detector from the geometry of the beam.
One of the major uses of mass spectrographs is to measure the relative abundances of different isotopes of the same or other chemical elements. The age determinations of rock samples rely on such determinations. We will discuss this technique in Lecture 16.
Mass spectrographs can measure ratios of species with such accuracy that they can also be used to get absolute amounts of elements using a trick called isotope dilution. Here, isotopic ratios, say 16O to 17O are measured in an unknown sample. Then that sample is mixed thoroughly with a known amount of material with a different 16O to 17O ratio, but for which the percentage of all oxygen is completely known. When the isotopic ratio of the mixture is again measured, one can tell how much total oxygen was in the unknown from the amount that the isotopic ratio changed.
Chromatography gets its name from a technique used to separate different substances in mixtures used in dying. If a cloth were dipped into such a solution, the liquid would begin to wet the cloth, but different constituents of the dye would climb the cloth at different rates. The result would be a cloth with stripes of different colors.
Chromatography today is used in a variety of ways having little to do with the color of anything. The basic mechanism upon which it is based is the mobility of atoms, molecules, or ions as a function of their physical and chemical properties. Chromatographs commonly employ either the gas or liquid phases of matter. In either cases there are similar basic constituents:
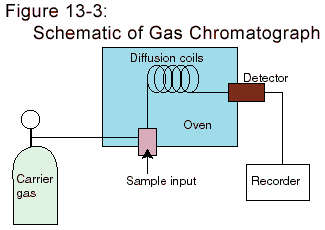
Figure 14-2 illustrates these components for a gas chromatograph. The supply for the carrier gas is shown on the left. This is often an inert gas, such as helium or nitrogen. The stationary phase is chosen so that the unknown species will be temporarily trapped by it. This trapping may take place by a variety of interactions, but they generally involve weak chemical bonding. A simple kind of trapping is by a process known as adsorption, in which a solid surface attracts a layer of gas molecules. If the stationary phase is liquid, the unknown samples can dissolve in the liquid, ultimately to evaporate from it.
Different "unknown" species will interact with the stationary phase in different ways and so reach the detectors at different times. A variety of detectors can be used to detect the presence of the (unknown) foreign species in the carrier gas. A common detector measures the thermal conductivity of the gas--the rate at which heat will flow across it. This depends on the composition of the gas. It is these times that are used to analyze an unknown mixture.
In space probes, a common detector is a mass spectrograph. The combination, mass spectrograph-gas chromatograph, is common enough to be known by the abbreviation GCMS. This combination is an integral part of the experiment to investigate the large moon of Saturn known as Titan.
One very powerful technique for the analysis of cosmic materials in the laboratory is irradiate an unknown sample with neutrons. The neutrons strike the nuclei within the sample, which then react in characteristic ways. The method is called neutron activation analysis, or NAA.
The neutrons used in this technique are typically obtained from a nuclear reactor. Many such reactors are available are available at national laboratories and universities. Neutron activation analyses are performed at the U of M with the help of the facilities of the Michigan Memorial Phoenix Project.
Each atomic nucleus is unique. It has its own energy levels, typically of the order of MeV apart. Since neutrons are uncharged, they are readily absorbed by most nuclei, to produce an isotope of the same element with one additional unit of mass. Not all chemical elements are easily analyzed by neutron activation methods, but many are. Let us take the lanthanide rare Earth cerium as a typical example.

Cerium has 4 stable isotopes: 136Ce, 138Ce, 140Ce, and 142Ce. The most common of which is 140Ce. When a thermal neutron is absorbed by a 140Ce nucleus, the isotope 141Ce is created. This isotope is unstable, and eventually decays to the stable 141Pr (praseodymium), with the emission of electron, a process we have seen before, called beta decay. The half-life for this transition is 32.5 days.
The 141Pr nucleus that is created by the beta decay of 141Ce is not in the ground state for that nucleus. It is in an excited state, 0.145 MeV above the ground state. The 141Pr nucleus quickly gets rid of this extra energy by the emission of a photon with 0.145 Mev of energy. It is this photon that is detected and measured in neutron activation analysis.
The gamma ray photon from the decay of 141Pr may be measured with a gamma-ray detector that operates on the principle of the photoelectric effect. One detector uses a germanium crystal which absorbs the gamma rays. Free electrons are then produced within the crystal, and they can generate a current, that can be made proportional to the energy of the incident gamma rays. In this way, the gamma-ray spectrum from the sample can be measured.
Ultimately the interactions that take place in an experiment of this kind are sufficiently complicated that it is necessary to calibrate the instrument with the help of a standard sample whose composition is already known. Such a standard is exposed to the neutrons of the reactor in a way that is as close as possible to that of the unknown material. Then signals from the known and unknown are compared.
When we may assume the responses of the instrument are linear, we can say the following. If the detector gives twice the current from 0.145MeV gamma rays from the unknown sample as from the standard, then there are twice as many 140Ce atoms in the sample as in the standard.
This is the essence of the neutron activation method. It is well suited to the analysis of whole-rock samples, as opposed to the analysis of some portion of a rock that might be isolated by physical or chemical means. Some 60 or so elements may be analyzed in this way, but one does not obtain direct information on the chemical or mineralogical composition of the sample.
In practice, in this as in most instrumental methods, there are many refinements and complications. We must leave them to those who actually perform the analyses.
Spectroscopy came into use in astronomy as well as analytical chemistry in the late 1900's. The technique is basically the same although it is more common in the laboratory to use emission spectroscopy for quantitative work than in stellar work. Astronomers have also analyzed many emission sources, from hot, diffuse gases. These gases may be excited by the ultraviolet photons from hot stars, or by shock waves from exploding stars.
Both in laboratory and stellar spectroscopy, there is a light source, and a spectrometer which produces a spectrum. The spectrum may consist of either bright or dark lines. The essence of the technique of spectroscopic analysis is that the strength of the lines is related to the number of atoms that produce them.
Laboratory analysts have a definite advantage over astronomers. They can compare the spectra of an unknown material with those of one or more samples whose compositions are completely known. If the strength of spectral lines of an element in some unknown sample are the same as those in a standard, then it may be safely assumed the number of atoms in both sources are similar.
Astronomers must know the detailed conditions of the stars or nebulae emitting the light they must analyze. They must know the temperature and density of the relevant gases. By contrast, the laboratory spectroscopist only needs to be sure that the unknowns and standards have been analyzed in the same way. This is not to say that the job of the laboratory analyst is a cinch. It may give some insight into why laboratory results are generally more accurate than astronomical ones, and why it is so essential for us to have "returned samples" from the planets.
When atoms combine chemically to form molecules, the relevant energy levels change in fundamental ways. Molecules have their own unique spectral lines. Moreover, these lines may be produced in ways not available to atoms.
As in atoms, the electrons in a molecule may jump from one energy level to another, with either the emission or absorption of a photon. Unlike atoms, molecules may store energy either in the form of rotation or vibration. The energies associated with molecular rotation or vibration are typically lower than those associated with electronic levels. The latter are, as in atoms, of the order of electron volts (eV).
Molecular vibration and rotation energies are one to many orders of magnitude smaller than electronic energies. Characteristic vibrational energies lead to spectra typically in the range of one to tens of microns (10-4 cm or 10-6 meter). Rotational spectra lie typically in the microwave region of the electromagnetic spectrum, with wavelengths of a millimeter to a meter.
Molecular spectra may be observed in emission or absorption from free molecules in the interplanetary medium or planetary atmospheres. In the Earth's atmosphere, there is extensive absorption due to water vapor. Because of that, much of the infrared spectra of stars and planets cannot be observed from the ground.
Very important advances have been made over the last several decades in the realm of reflectance spectroscopy. In this case, one examines the spectra of light reflected from a sample. This sample might be a planetary surface, in which case the source of light would be the sun. We will return to this method in Lecture 27, when we discuss asteroids and techniques of remote sensing.
One of the most useful analytical methods of the geologist is called optical mineralogy. The method goes back to Henry C. Sorby who examined the first thin section with a microscope in the mid 1800's. The technique requires grinding and polishing a sample of a rock or mineral to a thickness of 0.003 cm (or 30 microns micro-meters). These are mounted on slides, and examined with a petrographic microscope. The light from the source is made to pass through polarizing filters before it goes through the thin sections. The results are typically beautiful kaleidoscopic images generated because the light interacts differently with minerals in different ways.
People trained in optical mineralogy not only recognize the different minerals--a kind of quantitative analysis--but can also tell quantitatively how much of each mineral is in the sample. The relative fractions of different minerals is a primary factor that distinguishes rock types. Rough estimates may often be made from hand specimens, but the ultimate arbiter is the thin section. It is interesting that this general method was one of the most enlightening used in the study of the lunar samples.
In this course, we do not need to become experts in optical mineralogy. We only need to know that thin sections can be made, minerals identified from them, and their relative amounts evaluated.
The information from optical mineralogy is quite different in nature from that obtained from the techniques discussed earlier. While one may deduce some information on mineralogy from these methods, one gets it directly from the geologist's microscope. As we will see in Lecture 14, the mineral content of a rock is an important clue to its history.
Several methods of laboratory analysis involve firing beams of particles at samples and observing the X-ray photons that are then emitted. The particles may be electrons, protons, or even ions, for example, O- (oxygen with an extra electron attached). It is also possible to use X-rays photons in the exciting beam.
In all these instances a small volume of the sample is affected, typically, a micron or two in diameter. This means that it is possible to examine individual minerals in a rock sample, so these probes can give important information about the mineralogy as well as the elemental composition of a sample. Often, when a crystal forms by solidification within a melt, the composition of that melt will change while the crystal is forming. This can make the composition of the crystal change from the inside to the outside, a phenomenon known as zoning. Probes are ideal for investigating such changes in the composition of a zoned crystal.
Typically, when fast electrons from a probes strikes the atoms in a source, an electron is ejected from an inner shell. This creates a hole in the inner shell, into which an electron from an outer shell may drop. The jump of the outer electron into the hole is deeper into its potential well, and the excess energy is emitted as a photon. Inner shell electrons have energies in the X-ray region, so X-rays are emitted.
It is possible to distinguish among chemical elements by the wavelengths of these X-rays. Generally speaking, the lightest chemical elements are not easily studied from their X-rays.
Ion beams are used in a rather different way. They strike very small areas of a specimen, and deposit sufficient energy to create a small plasma (ionized gas) atmosphere over the point of impact. This plasma may then be analyzed using mass spectrographic methods. In this way one can get isotopic information, often lacking in other methods.
An important technique that has been used for the analysis of
lunar and Martian rocks in situ has been to fire
 particles at a small region, and examine the
results. Many of the
particles at a small region, and examine the
results. Many of the  's will orbit the nuclei
of atoms in the sample, and return in nearly the direction they
came from. This is called
's will orbit the nuclei
of atoms in the sample, and return in nearly the direction they
came from. This is called  backscattering.
The energies of the backscattered
backscattering.
The energies of the backscattered  's depend
on the mass and charges of the nuclei they interact with. One can
use this technique to determine the composition of the lighter
elements in the sample. This method was used to analyze martian
rocks.
's depend
on the mass and charges of the nuclei they interact with. One can
use this technique to determine the composition of the lighter
elements in the sample. This method was used to analyze martian
rocks.
The common analytic techniques that are used in the analysis of cosmic materials are (1) optical and mass spectroscopy, (2) neutron activation analysis, (3) electron and ion microprobes, and (4) optical mineralogy with a polarizing microscope. Remote sensing by reflectance spectroscopy will be covered in Lecture 27.
Optical
spectroscopy, in the laboratory or at astronomical observatories,
is based on the unique pattern of absorption or emission lines
from the chemical elements. In neutron activation, one observes
the varied reactions of atomic nuclei that have absorbed neutrons.
These nuclei emit gamma rays which are characteristic of the individual
nuclei. Microprobes fire beans of particles (or photons) at a
source. The analyst either examines X-rays, or in the case of
ion probes, uses a mass spectrograph to analyze the microplasma
created over the focus of the beam.  backscattering has recently been used to analyze Mars rocks.
backscattering has recently been used to analyze Mars rocks.
The thin section and polarizing microscope is a classical tool in geology that allows the skilled observer to recognize minerals and determine their relative proportions.

NASA Pathfinder Image
Rocks, Rocks, look at those rocks... We had gone to Mars to look at rocks... Why did we want rocks? Every rock carries the history of its formation locked in its minerals, so we hoped the rocks would tell us about the early Martian environment. The two-part Pathfinder payload, consisting of a main lander with a multispectral camera and a mobile rover with a chemical analyzer, was suited to looking at rocks. Although it could not identify the minerals directly--its analyzer could measure only their constituent chemical elements--our plan was to identify them indirectly based on the elemental composition and the shapes, textures, and colors of the rocks. By landing Pathfinder at the mouth of a giant channel, where a huge volume of water once flowed briefly, we sought rocks that had washed down from the ancient, heavily cratered highlands. Such rocks could offer clues to the early climate of Mars and to whether the conditions were once conducive to the development of life.Matthew P. Golombeck
Project Scientist, Mars Pathfinder
In Lecture 4 we pointed out that Earth's crust is only about 0.4% of its total mass. The oceans and the atmosphere are only about 6% of the mass of the crust. Most of the Earth is rock and metal. Apart from the liquid outer core, these materials are minerals.
There is little reason to believe that the bulk structure of Venus is significantly different from that of the Earth. Mercury appears to have a somewhat larger portion of metal than rock, while with Mars, there is more rock and less metal. As far out as the asteroids, we believe the denizens of the solar system are primarily mineralic in nature.
If we are to understand the current structure and history of these objects, we must learn something about the special chemicals known as minerals. This is the purpose of the present lecture.
We shall define minerals as naturally occurring materials that are usually solids with a definite crystalline structure and chemical composition. The classical definition of minerals omits the qualification "usually" and must draw a distinction between ice and liquid water. It must also make some provision for mercury, which occurs rarely in its elemental, liquid form. Biological processes are capable of producing crystalline solids that would not qualify as minerals under some definitions.
Books on mineralogy usually provide an description of calcite (CaCO3), whether or not the atoms were ever a part of a living creature. On the other hand, they do not describe crystallized protein, which surely has a regular spatial structure and chemical composition. The latter is clearly organic in origin, while the biological origins of the former are often shrouded by time, physical, and chemical processes.
There are problems with most definitions, as we pointed out in connection with the undefined terms of physics. However, these difficulties are rarely a bother to anyone other than pedants.
References on mineralogy list several thousand mineral names. Most of these minerals are rare, and of little relevance to the non specialist. There are two kinds of mineral names in common use, specific and general, and it is important to realize the difference. Mineral family names are often used. "The feldspars" provide a common example. The family name, "feldspar," includes the common pink potassium feldspar, and a sodium (albite) and calcium (anorthite) feldspar, as well as additional varieties that we shall not be concerned with.
Only a small number of minerals dominate the bulk chemistry of the Earth and probably all cosmic solids. We therefore set out a highly simplified classification of minerals, as follows.
The refractory oxides did not all vaporize when the solar system formed. Some of them were formed out in interstellar space, perhaps in the atmosphere of a red giant star. Their composition therefore is not the same as that of the solar system as a whole, the SAD. Here, we speak of the isotopic composition, the relative proportions of isotopes in the minerals. Since corundum is always Al2O3, its elemental composition is always the same. But there may be a different mixture of the three isotopes of oxygen: O-16, O-17, and O-18.
Mineral fragments that never vaporized when the solar system formed are called pre solar grains. Their investigation is one of the most exciting areas in the chemistry of cosmic materials. We will return to the topic of pre solar grains in Lecture 35.
Silicon forms many compounds for much the same reason that carbon does. Recall that the entire domain of organic chemistry is the chemistry of carbon compounds. Both carbon and silicon have 4 electrons in the outermost shell, 2 s-electrons and 2 p-electrons. These different subshells form a mixture in such a way that all 4 electrons are similar in nature. Chemists call such a mixture a hybrid. The flat formula for methane, CH4 is
H
|
H--C--H
|
H
This is supposed to show the 4 bonds are similar (the | and -- are as close as I could get them to look with the font available). Actually, methane is a 3 dimensional structure, with the bonds directed to the corners of a regular tetrahedron. This geometrical figure has four faces made of equilateral triangles. Each bond angle is equidistant from the other bond angles.
Silicon also typically forms bonds directed to the vertices of a regular tetrahedron. Silicon tetrahedra are SiO4, and this complex ion requires four additional positive charges for electrical neutrality.
A good example of a mineral where the silicon bonds to 4 oxygens with tetrahedral bonding is the family of olivines. These are Mg2SiO4 and Fe2SiO4, and mixtures with intermediate compositions, which we might write (FeMg)2SiO4. The mixture can form a solid solution of the two "end members." It is a miscible solution, that is, the solution is like alcohol and water rather than oil and water.
Mg_2SiO_4 Fe_2SiO_4
Forsterite(Fo) Fayalite(Fa)
| |
-------------------------------------------
100%Fo 100%Fa
0%Fa <-- Olivine--> 0%Fo
The next major family is the pyroxenes. This time there are four end members, but we shall only need the names of two of them, enstatite and diopside. The most common pyroxene of terrestrial rocks, augite, is a mixture of all four end members, but closest in composition to diopside.
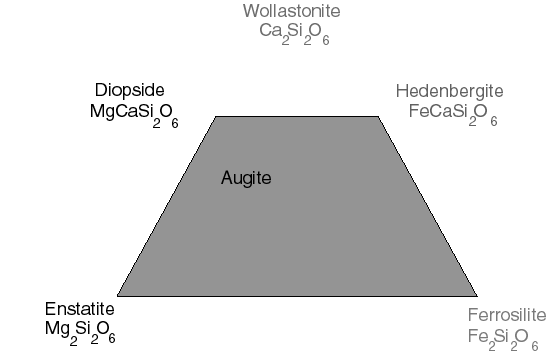
This can be thought of as the bottom part of a triangular diagram with Ca2Si2O6 at the top. However, this calcium silicate is not called a pyroxene because of the structure of its crystals.
The feldspars can be described with the help of a full triangular (sometimes called a ternary) diagram:
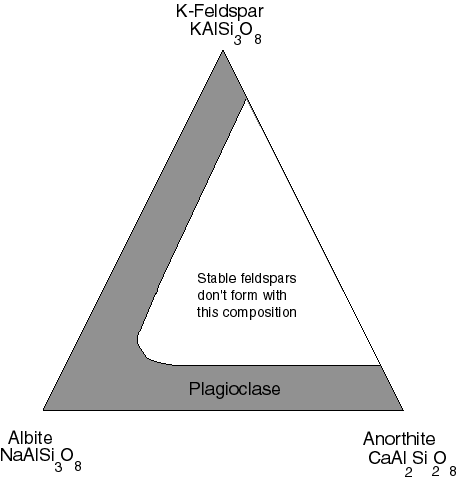
The two feldspars on the bottom form a miscible solid solution the general term for which is plagioclase. Anorthite is very common in lunar rocks. Rocks dominated by plagioclase feldspar are called anorthosite. The suffix "site" usually indicates a rock rather than a mineral--"ite" is a common ending for a mineral. Lunar anorthosites are dominated by the calcic feldspar. Much of the ternary field between K-feldspar and anorthite is not filled by natural minerals. The x's in the above diagram illustrate this area, but very crudely. Between albite and K-feldspar one gets an "unhappy" solid solution. Given enough time, the two feldspars will try to separate out from one another in the solid state. This phenomenon does not happen for an albite-anorthite mixture (plagioclase).
A mnemonic for the three common silicate families is to add an SiO2. Thus, enstatite Mg2Si2O6 has one more SiO2 than forsterite, Mg2SiO4 and albite NaAlSi3O8 has one more SiO2 than enstatite. The mnemonic isn't perfect. You'll sometimes see enstatite written MgSiO3, you still must remember that the feldspars have aluminum, and that the Al's and Si's change from albite to anorthite. Use the mnemonic if it helps.
The olivines, pyroxenes, and feldspars are the major minerals of the Earth's crust. They explain to a large extent why the crust is dominated by 8 elements: O (62%), Si (21.2%), Al (6.5%), Na (2.6%), Fe (1.9%), Ca (1.9%), Mg (1.8%), and K (1.4%). The percentages here are by numbers of atoms. You can see that these are the elements in the dominant minerals.
Note also that the elements Na, Al, and K, which all have odd Z are more abundant in the crust than you would expect them to be from their abundances in the SAD. In particular, compare these abundances with that of the even-Z element, sulfur, which is not a major element of the Earth's crust.
Clearly Na, Al, and K are abundant in the crust because they occur in the feldspar minerals. Next question: why are the feldspars so abundant in the crust of the Earth. They are not the major minerals of the far more massive mantle.
When a heterogeneous solid, such as a rock is heated, the first liquid that appears will not have the same chemical composition as the rock itself. Indeed, the melt is enriched in those minerals with the lowest melting temperature. If this melt is separated from its parent material, and then refrozen, the new rock will be enriched in those more easily melted minerals. If the new rock is again subject to partial melting, the new melt will be still richer in minerals that melt easily.
The rocks that make up the terrestrial crust have a complicated history of partial melting, freezing, melting and refreezing. Petrologists often speak of the "distillation" of the material. As any student of booze knows, if you distill a mixture of alcohol and water, the vapor is first more enriched in alcohol than the parent liquid. This same situation holds for mineral mixtures, except that in this case the relevant phases are solid and liquid, rather than liquid and vapor.

There are phase diagrams that are used to describe this process in detail. The upper phase diagram describes the behavior of a mixture of the feldspars albite and anorthite. Pure albite NaAlSi3O8 is at the left, and pure anorthite, CaAl2Si2O8 is at the right. A solid solution of these two minerals is called plagioclase. Note that the melting point of pure anorthite is considerably higher than that of pure albite. Anorthite is tough stuff, a fact which explains much of the chemistry of the lunar highlands. We'll come to that in Lecture 23.
If a solid plagioclase with the composition A' is melted, the first liquid to appear has the composition A. With further melting, the solid will move up the curve labeled solidus, toward the point C, and the liquid composition will move to the upper right, toward the composition A' again. When all of the solid has melted, the liquid will have the original composition, A', again, of course.
Partial melting by definition, means that not all of the parent material is melted. Then the liquid that may be removed will have a different composition--it will be chemically differentiated from its parent. In processes that take place on the planets and their moons, solids may be partially melted, and the liquids forced toward the surface through fissures or vents of various kinds.
The albite and anorthite are miscible in both liquid and solid solution. The lower phase diagram shows what happens when a solid containing diopside and anorthite crystals is partially melted. This time, there is no solidus. When the solid is heated, the first melt has the composition indicated by E. If the parent material has the composition A, that is, 80% diopside, and 20% anorthite, then some diopside will remain solid until all solid is melted. The liquid will begin with the composition E, and the melting will go on until all of the anorthite is melted. Then the liquid will gradually move up toward the composition A again.
It is not necessary for you to remember the details of the partial melting. For various mixtures, it can get very complicated. What you need to remember is that during partial melting, the most easily melted minerals will be the first to liquefy. This is the reason they work their way upwards--toward the surfaces of planetary bodies.
This is the situation for the sodic and potassium feldspars, as well as for quartz. They are more easily melted than the olivines and pyroxenes that form the bulk of the Earth's mantle. In addition, their densities are lower. These two factors insure that the feldspars and quartz will work their way upward as a result of melting and partial melting processes that go on in the Earth.
What processes cause partial melting? We know from seismology that most of the volume of the Earth is solid. But heat is generated within the Earth, probably mostly by radioactive decay, and it works its way non uniformly. Most of the melting in the present Earth is associated with plate tectonic activity, which we shall take up in Lecture 17.
Solid Earth materials are subject to two main processes, partial melting and weathering. While these can be very complicated processes, certain regularities do emerge, so that it is possible to say from the mineral content of a rock, if its constituents have had a complex history or not.
The American geochemist N. L. Bowen summarized the overall geochemical trends with a diagram that has come to be known as the "Bowen Series." There are two series, a continuous and a discontinuous one:
Olivines Anorthite
Pyroxenes Albite
Amphiboles K-spar
Micas
Quartz (SiO_2)
clay minerals
(soil)
The series on the left is called discontinuous because the olivines and pyroxenes are immiscible as solid solutions. The feldspars are all at least partially miscible, hence that branch of the series is called continuous.
Natural processes cause minerals at the top of the series to be transformed by a variety of chemical reactions to ones lower down. These processes may take place at low temperatures, as in the case of the weathering of feldspars to clay minerals. Important geochemical reactions also take place at the higher temperatures of magmas. In this course we will call the minerals at the top "early" and the ones at the bottom "late." A rock with mostly "late" minerals may be assumed to have had a complex history of partial melting and weathering.
We shall be concerned primarily with how easily minerals are melted and how dense they are. Generally speaking, these properties are closely related to positions in the Bowen series. The early minerals, at the top of the series are both denser, and less easily melted than the late ones. The properties are not parallel in the continuous and discontinuous branches. This is shown in the following table.
Mineral Formula Melting T(K) Density
Water=1
Forsterite Mg SiO 2163 3.21
2 4
Enstatite Mg Si O 1830 3.19
2 2 6
Diopside CaMgSi O 1668 3.30
2 6
Anorthite CaAl Si O 1830 2.76
2 2 8
Albite NaAlSi O ~1313 2.63
3 8

We can see that forsterite has both a higher melting point and density than it's opposite number in the continuous series, anorthite. However, anorthite has a higher melting point but a lower density than the common pyroxene diopside. This has important consequences for the formation of the lunar highlands, as we have already mentioned.
We have introduced four categories of minerals: native elements and alloys, oxides, silicates, and a miscellaneous category containing only a few carbonates, phosphates, or sulfides. Of these, the silicates are the most complicated. We discussed the olivines, pyroxenes, and feldspars. There are also hydrated and complex silicates, such as the amphiboles, and micas. Chemical formulae for 14 minerals were written. You need not explicitly memorize all of these formulae and names, but you should be able to recognize them, and put them in the appropriate mineral families. Use the mnemonic trick for the olivines, pyroxenes, and feldspars.
The Bowen Series relates geochemical processes on the Earth to mineral content of rocks. Rocks with complicated geochemical histories contain minerals that are late in the series. Typically, minerals that are early in the series are denser and have higher melting temperatures than those that are late.
The preponderance of the mass of the terrestrial planets is either metal or rock. To a geologist, any mineral aggregate is a rock, and if there is only one mineral in the mass, it may be called a "monomineralic" rock. The study of rocks is called petrology, from the Greek word for rock, "petro."
The three main divisions of rocks are igneous, metamorphic, and sedimentary.
Igneous rocks formed from a melt, which might have cooled slowly within a planet, or rapidly on its surface.
Sedimentary rocks were deposited from a fluid. In the case of terrestrial rocks, the fluid is almost always liquid water. An interesting case occurs when volcanic ash is deposited in layers from the air. It can be compacted into rocks with the mineralogy of igneous material. Such rocks, called tuffs, often appear layered, are light in color, and can be mistaken for limestones. Large volumes of tuff may be seen in Yellowstone Park. The most common sedimentary rocks are limestones and sandstones. None of the lunar rocks are sedimentary.
Metamorphic rocks are either igneous or sedimentary rocks that have been modified by heat, pressure, or shock. To metamorphose is to change. Common examples are gneiss and schist. Gneisses are typically squeezed igneous rocks that exhibit foliation or layering. Schists come from shales subjected to pressure, and for them too, the layering is apparent. Marble is metamorphosed limestone.
In this course we shall omit most of the complex processes of metamorphic and sedimentary petrology. Thus far, these processes belong (almost) entirely to Earth scientists. On the other hand, Moon rocks have been formed (almost) exclusively by igneous processes. When samples are returned from Mars, this situation may change completely. There is good evidence that liquid water was once common on the surface of that planet, so the probability of finding sedimentary or metamorphosed Martian rocks is reasonably high.
There is one metamorphic process that is highly relevant for lunar rocks. It is called shock metamorphism. Almost all of the lunar samples show evidence of extensive fracturing due to impacts of meteoroids during the last phases of lunar formation. Rocks that are made up of broken fragments are called breccias. Most of the lunar samples are brecciated.
The other source of extraterrestrial rock samples available to us are meteorites. These may contain igneous materials as well as those that have been subjected to aqueous alteration, that is, metamorphosed by water and water solutions. Other meteorites contain materials that are not accurately described by any of the terminology developed for terrestrial or lunar petrology. We postpone a discussion of these materials until Lecture 35.
We must consider one category of sedimentary rocks, the limestones. They are of great importance in the history of the Earth's atmosphere, because they now hold the Earth's complement of CO2. In Lecture 26, we will explain why there is so much CO2 in the atmosphere of Venus, and so little on the Earth.
With the complexities of most metamorphic and sedimentary processes avoided, we now turn to a consideration of igneous rocks.
Igneous rocks are described in a variety of ways. There are words to describe:
The following figure gives a simplified, two-dimensional classification of igneous rock types. The horizontal coordinate is related to the rock chemistry or mineralogy. The vertical coordinate is related to texture.
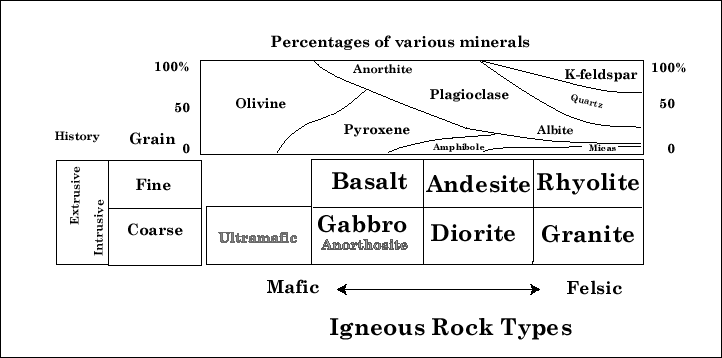
The names mafic and felsic are derived from chemistry. 'Mafic' derives from magnesium, and ferric. 'Felsic' comes from feldspar and silica.
Important chemical and mineralogical trends that take place from mafic to felsic rocks:
Some special rock categories that shown in small type in Figure 16-1 are:
Even though the mantle is mostly olivine and pyroxene, there is some extra SiO2 and feldspar. If mantle rocks are partially melted, the silica and feldspar can come squirting up through fissures and veins. We think of the crust as a kind of distillate. As we mentioned in Lecture 15, we use the notion of distillation, which commonly involves transformation of a liquid to a vapor. The recondensed vapor is called the distillate. In the present case, we are talking about solid and liquid material. Partially melting of mantle rock produces a liquid relatively rich in SiO2 and feldspar. When this freezes, it is a sort of distillate, and this "distillation" process is one reason why the crust of the Earth is chemically very different from the mantle. The other main reason is "weathering."
Sea floor spreading, typified by the mid-Atlantic ridge, builds an oceanic crust rich in mafic minerals that have been only slightly modified from mantle materials. Similar mafic rocks are thought to form the lower parts of the thicker, continental crust, which are often referred to as the sima, for silicon and magnesium. The upper continental crust, called the sial, is also enriched in aluminum, primarily from the feldspars. The oceanic crust, mostly sima, is some 10 to 12 km in thickness. The continental crust, sima + sial may be 25 to 35 km thick.
We now have the background to understand the results of the analysis of Martian rocks by the Pathfinder mission. In July of 1997, the lander deployed on the surface of the planet, and a roving laboratory and scout called the Sojouner began to analyze some of the nearby rocks. At the height of the mission, some of the names given to the rocks by the mission scientists, such as Barnacle Bill, and Yogi, became household words.
The principle instrument to probe the mineralogy was called an Alpha Proton X-ray Spectrometer. It is a kind of ion probe, as discussed in Lecture 13, but in this case, the ions were alpha particles, or helium nuclei. The particles came from radioactive nuclei that emit alphas, such as plutonium. This was a part of the instrumental package. When these alphas hit the rock, they interact with the atoms and nuclei of the rock to produce X-rays, protons, and simply backscattered alphas. From the energy spectra of these particles and photons, it is possible to determine relative atomic abundances in the sample.
Unfortunately, an analysis for the relative proportions of the chemical elements leaves the mineralogical composition of the rocks open. Geologists have a way of getting from the atomic percentages to plausible mineralogical compositions. Efforts of this kind are shown in Figure 16-2
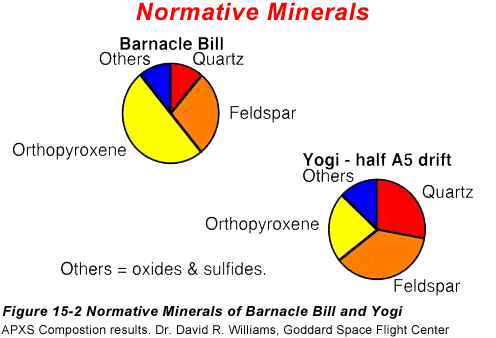
What we see from these pie charts is that the geologists believe there is a lot of quartz and feldspar in these rocks in addition to the mafic mineral pyroxene.
A plot that does not involve assumptions about the mineralogy is shown in the next figure.
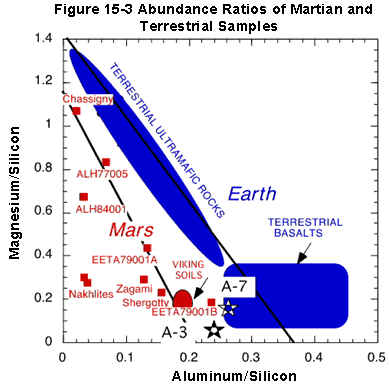
The two Mars rocks are indicated by the large stars, near the bottom-center of the plot. Barnacle Bill is A-7, and Yogi is A-3. What we need to notice about this plot is that the composition of these rocks does not resemble that of the terrestrial ultramafics. Indeed, our best guess at the bulk composition of the Earth's mantle would plot rather high and to the left in the broad area labeled "Terrestrial Ultramafic Rocks."
Putative meteorites from Mars, including the notorious AH 84001 with possible evidence of past microbial life, plot to the left of the terrestrial samples.
Press releases have described the "surprising results" of these analyses as indicating an overall composition for the rocks at the landing site as resembling the bulk composition of the Earth's crust--andesitic. We now understand what this term means, from Table 16-1. Moreover, we can also make some inferences about the maturity of the Martian rocks from the point of view of the Bowen series. The rocks have been subject to some distillation and perhaps weathering.
The overall analyses of these rocks have been a little confused by a dust coating. Attempts have been made to correct some analyses for contamination by this dust. The overall conclusion is that all of the rocks in the vicinity of the Rover had compositions resembling an `average' for the continental crust. Therefore, not so felsic as the sial, but definitely more differentiated than mantle ultramafics.
Two common words that are used to describe rock types are 'volcanic' and 'plutonic'. These words can mean very nearly the same thing as 'fine grained' and 'coarse grained'. Rocks from volcanoes are extruded on the surface of the Earth, and cool rapidly. Their grain size is therefore small. Anyone who has tried to grow crystals of sugar or salt knows it takes time to form large ones.
By this time, we have three terms to describe rocks that cooled rapidly: fine grained, volcanic, and extrusive. There's at least one more that the reader will be spared.
Some rocks cool slowly, because the magma from which they form did not erupt on the Earth's surface, but was intruded into a layer beneath it. These rocks are also called intrusive, and because large aggregates of rock formed in this way are also called plutons, such rocks are also called plutonic. Slow cooling allows for grains to grow in size, hence we have coarse grained, plutonic, or intrusive rocks. Again, we spare the reader additional names.
The professional geologist may use these words with shades of meaning beyond those of grain size. Usually, volcanic rocks are mafic, and often plutons are granitic or andesitic in composition. Thus chemistry as well as history might be implied.
Some rocks cool so rapidly no grains form at all, and the frozen material is said to be glass rather than crystalline. One may define a glass to be a solid lacking crystalline structure. Physical chemists describe glasses as supercooled liquids.
Glassy rocks are called obsidian if they are granitic (= rhyolitic) in composition. Obsidians are common roadside finds in Oregon, where volcanoes of the Cascade Mountains have ejected felsic materials.
Pegmatites fall at the opposite extreme. These are rocks with large grains, of the order of a centimeter or more. The most common pegmatites occur along with minerals late in the Bowen series, and are therefore granitic in composition. This means that pegmatites have had a complex chemical history as well as an extended cooling time.
Many of the trace chemical elements, the rare Earths, and the radioactive elements uranium and thorium have no major minerals of their own. They also have rather large ions, and have to force their way into rock crystals. This means that they tend to be preferentially retained in a melt, and that they are among the first materials liquefied upon partial melting. Thus, they tend to work their way upward, along with the typical felsic materials. Thus, granites tend to have more radioactivity than basalts, and pegmatities are even richer in these trace elements.
Will any pegmatites be returned from Mars or Venus? If so, we will know some of the chemical and physical processes that may be inferred from such a find.
An important trend in the properties of cosmic materials as the chemistry changes from mafic to felsic is the viscosity of the melt. Viscosity is the property of a fluid that makes it sticky. Tar is a viscous fluid that becomes less viscous as it is heated.
There are two properties of mafic lavas that make them less viscous than felsic lavas. First, they melt at characteristically higher temperatures, and most fluids become less viscous at higher temperatures. (Modern multi-weight engine oils are an important exception to this rule.) Second, the presence of the SiO2 in the magma is known to be an important factor in the viscosity, the more SiO2, the higher the viscosity. The nature of the interactions are rather complicated, and we must be satisfied with the general notion that liquid SiO2 makes for a sticky magma.
There are many volcanoes known in the solar system. Impressive volcanoes are known on Mars, and there may be more volcanoes on Venus than on any other planet in the solar system. As far as we know, none of these volcanoes are active, but on the Galilean satellite, Io, there is active volcanism.
A very simple division of volcanoes uses only two types:
The main difference in these extreme volcanic types is due to the viscosity of the lavas. If the lavas are mafic, shield volcanoes form. Felsic lavas tend to stick in vents, often forming plugs. When the pressure builds to the point that the plugs are ejected, an explosion often follows driven by the release of steam.
Volcanic explosions occur for reasons similar to explosions of chemical bombs--there is a rapid transformation in the phase of material from solid or liquid to vapor. In the case of the volcanos, water is dissolved in the magma. Because of the high pressures under the Earth, much more water can be dissolved in the liquid than would be possible at atmospheric pressures. The sticky felsic lavas seal the vents of the volcanos, and allow pressure to build. When cracks develop so the magma is open to the lower pressures above ground, the water comes rapidly out of solution, and because of the high temperatures, it comes out as a vapor rather than a liquid. This water vapor requires a much larger volume than when it was dissolved. The expansion is the source of the explosion.
The mechanism resembles what happens when a carbonated drink is shaken in the bottle and the cap suddenly taken off. Here, CO2 dissolved under high pressure comes out of solution when the pressure is released. The volume of the CO2 is much greater than the volume of the bottle, once the pressure is released.
Volcanologists have many tales of destruction by explosive volcanoes. In a notorious case, the town of St. Pierre on Martinique island was destroyed along with some 20000 inhabitants by the eruption of Mt. Pele in 1902.
Rocks may be igneous, metamorphic, or sedimentary. The former are most important for astronomy. Rocks names may describe the chemistry, texture, history of a rock or a mixture of these. Mafic rocks contain ferromagnesian minerals while felsic are rich in feldspars and silica. From mafic to felsic, the fine-grain types are basalt, andesite, and rhyolite. The corresponding coarse-grained types are gabbro, diorite, and granite. Anorthosites, dominated by plagioclase feldspar, are common on the Moon.
Explosive volcanoes are associated with viscous, felsic lavas. Mafic lavas flow more readily, and tend to form shield volcanoes.
Geology is the study of a planet.
We have discussed how waves can travel down a rope when we described a model for electromagnetic waves. That kind of a wave is called a shear wave.

When the material a wave is running through is moving perpendicular to the direction of wave motion, the wave is said to be a shear wave.
Shear waves are unable to travel through gases and liquids. All three phases are capable of transmitting pressure waves.
When pressure waves run through matter, the particles oscillate in the direction of propagation of the wave itself.
In both pressure and shear waves, there is no net motion of particles of the medium. The waves travel, but the particles only oscillate over a limited distance.
The velocity of a wave through matter depends on two main factors. Both pressure and shear waves travel more slowly through a denser medium than a less dense one. The velocity also depends on the strength with which the medium resists deformation. Most solids are a little more resistive to a compression, which reduces the volume, than a shear, in which the volume is distorted in shape, but not changed in size. This latter property of wave motion makes pressure waves travel faster than shear waves through the same medium, and gives rise to the geophysicist's designation of the pressure waves as `P', and the shear waves as `S'.
It is a useful mnemonic that pressure waves are designated with a P, and shear waves with an S. The origin of these letters came about in an entirely different way.
Geologists use instruments called seismographs to detect wave motions in the Earth that are generated by earthquakes. The first waves to reach the instrument are called primary, or P. These are characteristically followed by slower waves, the secondary, or S waves.
The instrument in Figure 17-3 illustrates the general principle on which all seismometers work. Part of the apparatus will move along with the earth. In the figure, this is the base. A second component is constrained by inertia so that it will not respond immediately to short-timescale Earth movements. This is the arm, weighted in the figure with a brick! The motion of the Earth may be recorded with a pen on moving paper, or in modern instruments, an electrical signal is generated, amplified, and eventually displayed.
The speed of the two kinds of seismic waves depends on the composition and state of the material through which they travel. It is possible to use them to gather information about the unseen interior of the Earth. The simplest example of this is the location of the Earth's core. It is obvious that the Earth's composition must be a mixture of both metal and rock from its mean density. Even if we allow for compression, the decompressed density of the Earth is higher than any plausible rocky composition it might have.
The Earth's core was discovered in 1906 by the Irish geologist Richard Oldham. It is interesting that he did not get a clue to the presence of the core from the S waves, which are actually incapable of being transmitted through the liquid of the outer core. Rather he noted the existence of a shadow zone in which P waves from an quake in the opposite hemisphere of the Earth failed to appear.
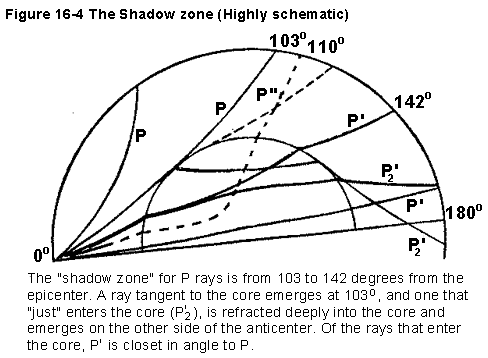
Waves traveling through the body of the Earth are bent outward, as shown in the figure because of refraction. This is the same phenomena that causes light to be brought to a focus by a lens. The difference is that when light enters glass from the air, it moves into a medium where its velocity is lower. The ray is therefore bent toward the normal the the surface.
The velocity of seismic waves, both S and P, increase as they move into the interior of the Earth, and therefore their trajectories are bent away from normal, or outward, as shown. The reason for the increase in the wave speed is related to the difficulty of compression (or shearing) of the material as it is subjected to ever increasing pressures of the overlying layers of the Earth. There is, actually, a competition between the increase in density and the increase in the forces that resist deformation. The former would make the waves travel more slowly, the latter more rapidly. In this case, the latter forces win out, and the wave velocities increase with depth in the Earth.
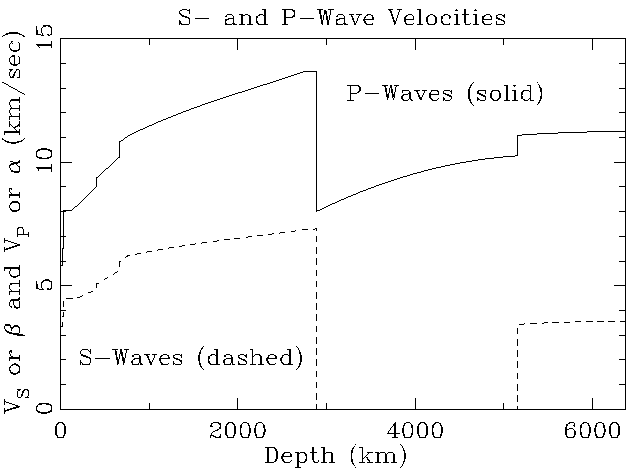
At the boundary to the outer core, the phase of the material changes from solid to liquid, and the resistance to deformation changes accordingly. The S waves are not transmitted through the outer core at all, and the velocity of the P waves drop significantly.
Whenever waves encounter a medium in which the velocity changes, they may be reflected as well as refracted. In general, this happens at every surface. Astronomers coat the glass surfaces of lenses with a special material that reduces the amount of light that is reflected from them. This increases the efficiency of their instruments since the reflected light is typically lost. The geologist cannot do this with seismic waves, of course. Therefore every P wave that strikes the core is partially reflected from it, and partially transmitted into it.
S waves cannot travel into the core, but their energy can be transformed into a P wave at the surface of the core, and the resultant wave can propagate through the core, to emerge as partially P and partially S.
The zone of rapid rise of seismic velocities for waves descending from the crust of the Earth is called the Mohorovicic discontinuity or Moho. It marks the transition from the Earth's mantle to the crust.
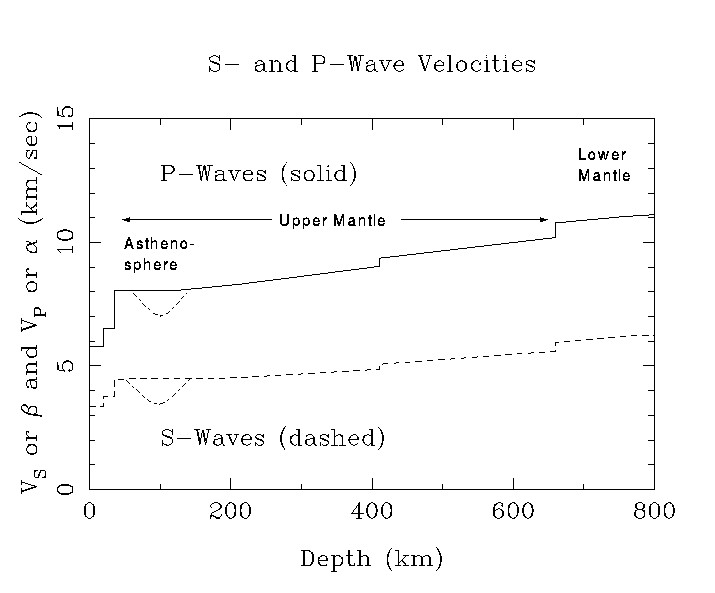
The Moho is the step to the first long shelf of the upper mantle. The asthenosphere is indicated by the dashed lines, indicating (in a highly schematic way) lowered velocities in the mantle due to softening of the material.
Figure 17-6 is an expansion of Figure 17-5, with some annotations. It shows the basis for divisions of the mantle into inner and outer zones. The jumps in wave velocity correspond to changes in the crystalline structure of the olivines that make up the bulk of the mantle. Two transitions occur, one very near 400 km depth, and another at a depth of about 650 km. As the pressures of the Earth's layers increase, the olivines first change the arrangements of their ions into a structure that resembles that of another family of minerals known as spinels. This change is a physical and not a chemical change. At greater depth, both a physical and chemical change takes place:
The olivine changes into a silicate with the composition of a pyroxene, plus an oxide. The structure of the MgSiO3, the arrangement of the ions, is not the same as that of the common pyroxenes, but resembles the oxide perovskite, CaTiO3. This mineral form has only recently been understood as a result of experiments that have been carried out with diamond anvil presses, capable of reaching the necessary pressures to bring about this phase change. We must leave the fascinating technology and experimental results with diamond anvils to web surfers. Just enter "diamond anvil", and away you go!
The new geometrical arrangements of the ions as a result of pressure changes both the density and the resistance of the materials to deformation. Generally speaking, the resistance to deformation has a larger influence on the velocities of the seismic waves, so these velocities increase, causing the upward steps to the right (toward the center of the Earth) seen in Figures 17-5 and 17-6.
Modern geophysicists analyze a broad network of seismometers with the help of sophisticated analytical programs. The results are three-dimensional images with far more detail than the classical picture of shells. The basic technique is not unlike that employed in medical cat scans of the human body. This technical term for building higher dimensional images is called tomography. The seismic waves, for example, travel along a one-dimensional path from an earthquake to a given seismometer. However, information from enough of them gives two and three dimensional pictures of the Earth's interior.
With the help of these methods it has become possible to study in detail the modes of transfer of heat from the center of the Earth to its surface.
The planets all have both internal and external heat sources. The most important external heat source now is the sun, but in the past meteoroid bombardment supplied a good deal of heat. Internal heat sources derive from radioactive decay, chemical energy, and gravitation.
The average heat coming from the Earth's interior is estimated to be about 4 x 1013 Joules/sec, or 4 x 1013 watts. Radioactive elements within the Earth are expected to provide just about this amount. We shall make more quantitative estimates of this heat in Lecture 29.
Heat may be transported by three classical mechanisms: conduction, radiation, and convection.
Heat is transported by conduction when there is no net motion of the molecules through which the heat is flowing. Molecules in regions where the temperature is high pass their energy to regions where the temperature is lower by collisions or by oscillations. If you stick a poker in a fire, eventually the end you are holding gets hot. There is no transfer of iron atoms down the poker. The ones in or near the fire oscillate more rapidly than those where it is cooler, and pass their energy down the rod of the poker.
In radiation, photons transport energy from regions where it is hot to those where it is cooler. Energy from the sun comes to us by radiation.
In convection, there is a physical transport of hot material to regions where it is cooler. This transport often comes about because hotter materials are less dense than their surroundings, and they tend to rise. In the interior of the Earth, it is thought that very slow convection currents move hotter rocky material from deep regions toward the surface.
In classical laboratory experiments done at the end of the 1800's, convection cells were observed in laboratory fluids that were heated from below. These cells lasted for long periods of time relative to the turnover time for the fluid, and had a regular, honeycomb-like appearance when viewed from above. If the heating from below was increased, the cells became irregular in shape, and individual cells would persist only for one or several turnover times for the fluid.
Convection cells are common in the atmospheres of planets. The Earth is no exception. Such cells can often be seen in cloud layers from an airplane flying above them. We have mentioned this in connection with the sun's atmosphere. In the Earth, the motions are very slow indeed. They must take place in a medium that transmits seismic shear waves. However the distortions in these waves are very rapid compared to the motions thought to occur in mantle convection. Here the motion is comparable to that of the continental plates. Indeed, their motion is thought to derive from the circulatory pattern of mantle convection.
It is not certain how deep the convective cells really go. Figure 17 - 7 shows a cell that involves both the upper and lower mantle. Some geophysicists believe only the upper mantle is involved in convection.
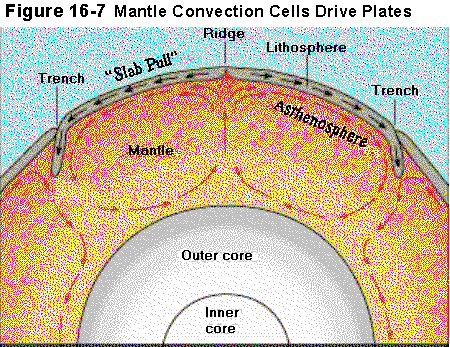
The crustal plates are thought to be driven from below by convection on a time scale of about 100 million years. The rate of motion of the plates is about an adult's height in one lifetime. In round numbers, say about 2 meters in 100 years. That makes 2000 km in 100 Million years--roughly correct. Continental drift, an older term with much the same meaning as plate tectonics, was strongly advocated by Alfred Wegener in the early decades of the 20th century. It was not accepted until convincing observations were made of sea-floor spreading in the mid-Atlantic ridge.
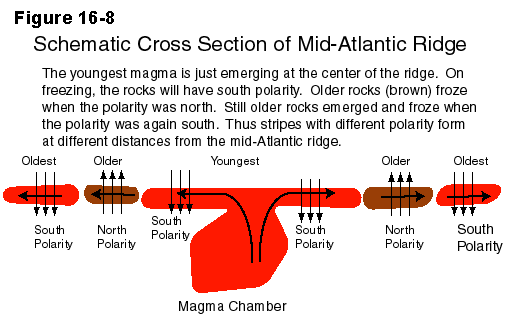
The plate boundaries on the Earth are well delineated by earthquakes and active volcanoes. Many of the Earth's gross features may be accounted for in terms of the motions and interactions of these plates.
When the plates move toward one another, one of the plates may sink beneath the other. In this way, crust is destroyed, and we have the opposite of what occurs in sea floor spreading, where crust is created. The region where one plate is moving beneath another is called a subduction zone. There are several possibilities when plates collide, because the plates may be made of either (thin) oceanic or (thicker) continental crust. Here are some classical processes that take place at plate boundaries.
Until the spring of 1999, there was little evidence for plate tectonic activity on any body other than the Earth. But in April of that year, NASA announced results from the Mars Global Surveyor, of a pattern of magnetic "stripes" running from east to west in Mars's southern hemisphere. These stripes appeared to be similar to the ones found on either side of the mid-Atlantic ridge, which played a key role in the final acceptance of the theory of plate tetonics. The consequences for the overall history of Mars could be extensive, but as of this writing, the information is too new for definitive statements.
Thus far, we know of no evidence for plate tectonics on Venus. The adjective ``tectonic'' may apply to a variety of processes, including the building of mountains or volcanoes, or localized (not global) motions of broken crustal blocks. There has been extensive "tectonic" activity, on all of the solid surfaces of planets and satellites in the solar system. We shall explore some of them in detail.
When rocks freeze, any radioactive elements slowly transform themselves, from parent to daughter atoms. Some of the daughter element may have been present at the time the rock froze. If we can determine the amounts of the parent and daughter both now and when the rock froze, we can tell how long ago that freezing took place. This is because the parent decays to the daughter at a rate that can be measured in a laboratory experiment.
Let PF be the amount of the parent present when the rock freezes, and Pt be the amount of the parent at the time t. Then the law of radioactive decay gives
 t)
t)
The constant  in this formula
is simply related to the
half-life. Put Pt/PF = 0.5 in the above
formula, and solve for t = t1/2, the half-life. We
readily find t1/2 = 0.693/
in this formula
is simply related to the
half-life. Put Pt/PF = 0.5 in the above
formula, and solve for t = t1/2, the half-life. We
readily find t1/2 = 0.693/
Of the 92 elements between hydrogen and uranium, all after bismuth Z=83 have only radioactive isotopes. Moreover, two elements much lighter than bismuth have no stable isotopes. These two elements are technetium (Z = 43), and promethium (Z = 61). Several other elements have radioactive isotopes that are useful for dating materials. These elements can also supply energy to the interiors of planets and satellites.
A few examples of important radioactive isotopes are:
Isotope half-life (years) C-14 5730 K-40 1.28 x 10^9 Rb-87 4.8 x 10^10 U-235 7.04 x 10^8 U-238 4.47 x 10^9
C-14 or 14C is useful for dating materials that are no older than some 30 to 50,000 years. Here is what happens.
Cosmic rays--mostly fast protons--smash into atoms in the upper atmosphere, and split their nuclei. In some cases, free neutrons are produced, and these bump into nitrogen atoms. The most common isotope of nitrogen, N-14, absorbs a neutron, and emits a proton, becoming C-14. The C-14 then decays with a half-life of 5730 years.
If the production rate of C-14 remains constant over several half-lives, then it is straightforward to show that an equilibrium ratio is set up of radioactive C-14 to the other atmospheric constituents.
Plants take in all isotopes of carbon when they process CO2, and therefore, a fixed proportion of this carbon is C-14. After the plant dies, the C-14 begins to decay. We can tell how old the plant is by how much of its carbon is C-14. The same situation holds for animals, since they must eat plants, or maybe they eat other animals that eat plants. Ultimately, all energy to run most life forms comes from plants or bacteria via photosynthesis, which is the conversion of CO2 + H2O to carbon-containing molecules.
The C-14 method is not good for deep geological time, that is, times of the order of hundreds of millions of years or more. The 50,000 years mentioned above is only a small fraction of the way, for example, to the KT (Cretaceous-Tertiary) boundary, corresponding to the extinction of the dinosaurs.
A method that is much more satisfactory for deep time uses Rb-87, which decays to Sr-87. We would expect all rocks to have some Sr-87 initially, that is, before the Rb-87 started to decay. Some of the Sr-87 therefore comes from the Rb-87 and some was present to begin with. How do we tell the difference? When several minerals are present, as in most rocks, it is possible to determine both the age of the rock and the initial Sr-87 present in it. The resulting age is called a "sample age." In practice, geologists use mass spectrographs (Lecture 13), and they measure ratios of the parent and daughter isotopes to an isotope that is not involved in the decay. Sr-86 is usually chosen.
At the time a rock freezes from a liquid, the ratio of Sr-87 to Sr-86 will be the same for all minerals, because these isotopes have the same chemical properties. What is important in this instance is the radii of the isotopes, because this determines how they will fit into the crystals of the minerals making up the rock. While the two strontium isotopes will have the same ratios in the rocks, the ratio of Rb-87 to Sr-86 will be different from one mineral to the next because the radii of Rb and Sr ions are very different. For example, Rb and K have about the same ion sizes. So the Rb-87/Sr-86 ratio will tend to be high in potassium feldspar (KAlSi3O8). But in an olivine, (Mg2SiO4) there wouldn't be much room for the large Rb-87 ions, and the Rb-87/Sr-86 ratio would be low.
Consider a plot of Sr-87/Sr-86 (y-axis) vs Rb-87/Sr-86 (x-axis). When the rock first freezes, the y-points will be the same because of the identical chemical properties of the strontium isotopes. The x-points will be different for each mineral.
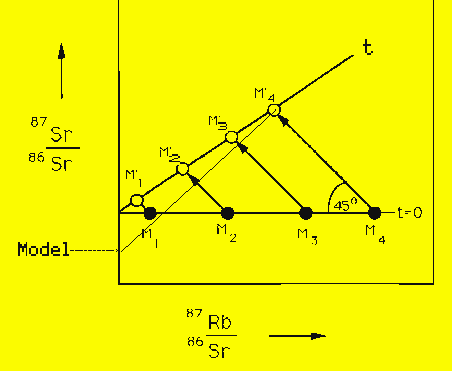
As time goes on, the x-values of points along the x-axis will decrease as the Rb-87 turns to Sr-87. The points on the y-axis will increase by exactly the same amount, since the Sr-87 comes from the Rb-87. In the ideal case, the points for each mineral will continue to fall on a single line, but the slope of that line will increase with time. Therefore, the slope gives the sample age of the rock, that is, the time since it froze.
We can get the initial Sr-87/Sr-86 ratio by looking at the intercept of the points, that is, the y-value for x=0. This is essentially the y-value for a hypothetical mineral with no Rb-87 to decay to Sr-87, so its initial Sr-87/Sr-86 ratio is its ratio for all times.
As time goes on, the total amount of Rb-87 decreases, and the total amount of Sr-87 increases in the rock. If the rock is softened by heat, the radioactive "clock may be reset." This can happen if the ions in the rock can move freely--essentially, the rock becomes a solution again. The next time the rock freezes, the minerals will again have the same Sr-87 to Sr-86 ratio, but that ratio will be greater than when the rock last froze.
Crustal rocks with complicated histories typically have higher values of the initial Sr-87 to Sr-86 ratios than mantle rocks. And they also have shorter sample ages.
Geologists also give another kind of age called a model age. In this kind of age, the initial Sr-87/Sr-86 ratio is assumed. You may read about the method from the link. The USGS has a great page on radioactive dating and the history of the Earth.
The Earth is a large body, and it certainly seems to be flat. It's only when there is some way to examine enough of it that becomes clear it's round (spherical). Many aspects of the physical universe become fundamentally different when it is possible to view them from a broad perspective. Our ideas about the nature of living things changed fundamentally with the use of the microscope. Similarly, powerful telescopes have enabled us to see that our universe extends well beyond our solar system and Galaxy.
There is an interesting chapter in the history of geology that illustrates this. It concerns speculations on the age of the Earth. During the Middle Ages, most educated people tried to discern information about the age of the Earth from the scriptures. The famous Irish cleric James Ussher concluded in the mid 1600's that the world began in 4004BC. Some 200 years later geological wisdom was that there was no beginning to the world at all!
The exorcism of the idea of a beginning of the world is often attributed to two British geologists, James Hutton (1726--1797) and Charles Lyell (1797--1875). Lyell's Principles of Geology was the definitive work for many years, and echoes of it remain in geology texts today. Those scholars whose outlook was based largely on notions of the ``creation'' and the ``flood'' came to be known as catastrophists. They thought there was a time when the world was different in most ways from what it was in their time. At one point, for example, it was ``without form and void.''
Today, relatively few who call themselves scientists take the this view, but those who do are not difficult to find on the internet.
Hutton and Lyell were uniformatarianists. The uniformitarian point of view is probably best expressed in Hutton's poetic words. He presented a summary of his geological ideas in papers read to the Royal Society of Edinburgh in 1785, which concluded as follows: The result, therefore, of our present enquiry is, that we find no vestige of a beginning,--no prospect of an end. Lyell was somewhat more cautious. He wrote that any time when the Earth was fundamentally different from it's present state was outside the bounds of what he considered to be legitimate science.
There is an irony in this. Hutton and Lyell may be considered true scientists, who laid the foundations of modern geology. Nevertheless, from the modern point of view, they were very wrong about beginnings and endings. Why?
When we review the kinds of information available to geologists in the eighteenth and nineteenth century, we find it severely limited. Radioactive dating of rocks did not occur until the early 1900's. Tectonic activity and erosion erased most of the evidence of the early Earth. What Hutton and Lyell saw, then, resembled a manuscript, with erasures superimposed upon erasures. They had no basis to conclude that the manuscript had once been empty.
Most of Lyell's geology deals with the most recent 0.6 billion years of the Earth's history. That time represents the interval in which bones and shells could be found in the form of fossils. Prior to this period, soft-bodied life left few traces. Prior to the use of radioactive dating methods, fossils were the most common tools used to give relative sequences for layered structures of the Earth. It is therefore not surprising that geology concentrated on the rather short time interval for which this tool was available.
It is quite clear that the uniformitarians simply had too restricted a view to be able to see back to the birth of the Earth. They were in some ways like the people who looked around and concluded the Earth must be flat. This is what we mean by a flat-Earth view of Earth history.
The contemporary author John McPhee has written a number of popular books on geology, and has contrasted views of the past history of the Earth. Most who based their estimates of Earth history on religion have rarely thought the Earth was as old as 10,000 years! Hutton and Lyle--and Darwin--thought in terms of tens and hundreds of millions of years. McPhee has said that they grasped deep time. In fact, their perspective was limited. We think the Earth is some 4.5 thousand million years old.
The late Harvard professor of geology and zoology Stephen J. Gould wrote a rather severe criticism of the 19th century stalwarts Hutton and Lyell. The criticisms appear in one of Gould's lesser-known works, entitled Times Arrow, Time's Cycle.
The uniformitarian theory of Earth history had no ultimate beginnings or endings, but there were definitely processes at work. These processes built and eroded mountains, and created lakes and streams. With no beginning or end, there was nothing else for these processes to do but cycle.
The problem with this approach is that it was invalidated by evidence already known to 19th century geologists in the form of the fossil record. Indeed, William Smith, by the end of the 18th century, had made use of fossils to identify geological strata, and put them in time sequences relative to one another.
Smith used the principle of superposition which goes
back at least to Nicolas Steno (1638-1686). This principle
seems almost like common sense, but was a formidable intellectual
step centuries ago. It says, for example, that of two horizontal
geological strata, say one of sandstone and one of limestone,
the one on top is the youngest. Similarly, if a dike of quartz
passes through these layers, that dike must be younger than
either of them.
In the illustration to the right, the plaque reads: Pegmatite dikes of different ages which have invaded granite. Note that the horizontal dike must be younger then the two more nearly vertical dikes across which it cuts. Clearly all dikes must be younger than the host rock. The word "pegmatite" refers to the grain size rather than the chemistry of a rock, but pegmatites are typically granitic in composition. The pink color is surely due to potassium feldspar, which is probably more abundant in the dike than the host granite.
Steven J. Gould made the point that the fossil record, unlike that of rocky layers and dikes, was monotonic in time. It was easy enough for Hutton and Lyell to see that rocky layers could become inverted or melted in such a way that similar patterns could recur in time. But fossils in the oldest layers did not recur in the younger. Indeed, it was possible to use the fossils to estimate relative ages of geological strata. This provided an "arrow" for time--a unique direction. Hutton and Lyell thought the various processes taking place on the Earth went through endless cycles.
Hutton, and Lyell--until the end of his life--never accepted the fossil record as evidence that Earth's history did not go through cycles. For this Gould takes them to great task.
The story is interesting because Hutton and Lyell are generally seen as giants, standing at the foundation of modern geology. One can view their apotheosis in the following way. Check a modern geology text, and look up the principle of uniformitarianism. You will probably read that it means that the laws of physics (!) have not changed in time. This sometimes reminds me of the one-time adversary of Galileo, Cardinal Bellarmino, who said that if the scriptures seemed to be at odds with our interpretation, we need to modify our interpretation. We have pointed out earlier that belief in the constancy of physical laws is virtually essential to the scientific method. Undoubtedly Hutton and Lyell embraced it too. But they surely meant more than that by uniformitarianism, and Gould has a valid criticism.
It's one thing to be correct, and yet another to be fair. It is fair to say that the geological record was still very incomplete in the time of Hutton and Lyell. This incompleteness may well have left legitimate room to believe that eventually cycling might be found in the fossil record. On more than one occasion scientists have gone out on a limb, and made assumptions that were necessary to preserve some concept they felt too dear to relinquish. This happened when Wolfgang Pauli postulated the neutrino to save conservation of energy and momentum. It is happening today, when dark matter is postulated to save the law of gravitation. We know that Pauli was right. The jury is still out on dark matter.
Seismic waves probe the interior of the Earth. S and P waves propagate through the mantle, and reveal changes in the physical state with depth. With the help of tomographic techniques, seismic data has revealed three dimensional patterns consistent with convective motions that drive the surface plate tectonic motions. Plate interactions, including subduction, account for many of the gross surficial features of the Earth. Convection cells carry heat that is generated by radioactive decay. It is not yet clear whether these cells extend into the lower mantle, or are confined to the upper mantle.
The age of the Earth is now well established by radioactive dating of rocks. Sample and model ages of rocks are determined from well-understood principles of radioactive decay.
James Hutton and Charles Lyell established modern geological principles. They firmly grasped ``deep time'' even though their view was limited. Their principle of uniformatarianism--a kind of steady state picture of Earth history--requires some reinterpretation to make it consistent with modern views of the age of the Earth.
What happened during the formative phase of Earth history, the hundreds of millions of years missing from the geologic record? The Moon holds the answer. The Moon is too small to have plate tectonics or own an atmosphere. There is no mountain building, it never rains, and rocks don't weather like they do on Earth. Though churned by meteorites over the ages, the otherwise pristine lunar surface retains a record of its embryonic development. The scarred and cratered moonscape reveals what a horrendous time this was.
--J. William Schopf (Cradle of Life, 1999, Princeton Univ. Press)
Physiographic provinces are regions with similar geomorphology, that is, they are similar in form. A few of the physiographic provinces of the continental US are shown in Figure 18-1.
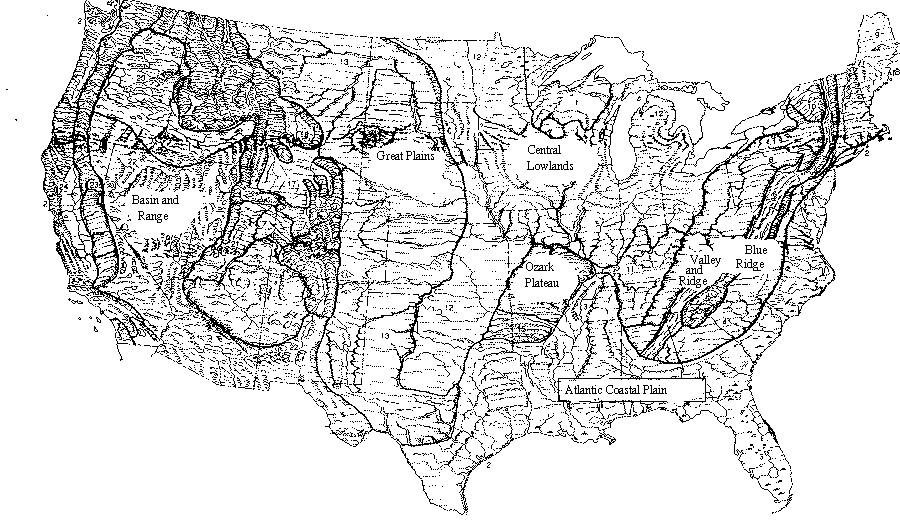
It is not necessary to remember all of the names of these provinces. There are at least 24 of them, but it will be necessary to remember enough so you get the idea. If you remember the names of the central lowlands, where we live, and the basin and range provinces, that should be enough. Basin and Range is the name of a popular book on geology by John McPhee, who introduced the colorful phrase ``deep time,'' that we used in the last lecture.
On the Moon, there are only two physiographic provinces, the (1)highlands and the (2)lowlands. These two provinces may be seen in a striking false-color lunar image made by the Galileo spacecraft on its way to Jupiter. As is explained in the caption to the figure the highlands show up pink, while the lowlands are either blue or blue-green. The colors are part of a remote sensing experiment, which uses techniques that we mentioned briefly in Lecture 13. We will have more on remote sensing in Lecture 27, when we discuss the asteroids.
The lunar physiographic provinces can be seen with the naked eye. They are what makes the features sometimes said to be the "man in the moon." With only mild optical aid, with binoculars, for example, the difference in the colors of the Moon's surface are easy to see. The dark areas are called maria or seas. Ironically, they are dryer than terrestrial deserts. The highlands are much whiter, and it is easy to see where the highlands start and the maria stop.
Here are the names of the major lunar basins, along with the locations of the Apollo landing sites. The Fall 1996 class had a contest for a mnemonic for the first 8 maria. The winner was, going clockwise from Imbrium: I'm Sure That Frogs Never Need Hair Pieces. To these, you need to add Mare Crisium, not included at that time. We shall have another contest, which includes Crisium, winner to receive the usual reward.
Numbers refer to x I - Mare Imbrium
Apollo landings x x S - Mare Serenitatis
x I15 S x T - Mare Tranquillitatis
x 17 x F - Mare Foecunditatis
x P 11T c x N - Mare Nectaris (to east)
x 12 14 16 x N - Mare Nubium (to west)
x N F x H - Mare Humorum
x H N x P - Oceanis Procellarum
x x c - Mare Crisium
x
The Fra Mauro crater, within the rectangular outline, marginally visible on this image, is an important site. We will make a figure of it below, but you should use your browser to get a better view.

Prior to the return of the samples from the Moon, no one was sure what the rocks would be like. There was some speculation that they would be pristine in their composition, that is, they would be like the SAD, but without the hydrogen and helium. Other speculations ran the gamut of possible rock types, from mafic to felsic, but with a slight preference for the latter, based on the properties of light reflected from the Moon's surface.
Astronomy books written in the pre-Apollo era described in exquisite and boring detail the various lunar features, the mountains, the rills, the ridges, the craters, mostly with no hints about how these features came to be. One school of thought was that the maria were low regions that had been filled with dust or chips. These people thought the first astronauts might sink out of sight into dust layers possibly up to a mile in thickness.
The returned samples from Apollo 11 (the first) told much of the tale. It landed in Mare Tranquillitatis, so the majority of their samples were characteristic of the lowlands, or mare. These rocks are basalts, similar in nature to flood basalts known on the Earth.
Terrestrial flood basalts are found in eastern Washington state, as well as at the Snake River plain in Idaho. The mafic lavas flowed over great distances because of their low viscosity. This is true for both the Earth and the Moon.
Among the Apollo samples were some fragments of nearly pure plagioclase feldspar. The American astronomer John Wood then predicted that this kind of rock, an anorthosite, would dominate the composition of the highlands. Subsequent missions confirmed his prediction. Some rock classifications group the anorthosites with the gabbros. Gabbros, generally speaking, are the coarse-grained counterparts of basalts, and thus much richer in olivines and pyroxenes than feldspars. However, there is some of the calcic feldspar in basalts and gabbros. Some subcategories of gabbros have olivine with no pyroxene, others have pyroxene with no olivine. If both olivine and pyroxene are missing, and the remaining dominant mineral is (calcic) plagioclase, then the anorthosite could be called a kind of gabbro. The lunar highlands owe their unusual chemistry to the properties of the calcic feldspar anorthite.
We have discussed the influence of plate tectonics on terrestrial surface features in Lecture 17. The plates move on a time scale of hundreds of millions of years. The Atlantic Ocean, for example, opened some 150 million years ago. At this time, the Earth was quite different from today, or even in the days of Hutton and Lyell. Dinosaurs roamed the land, and would do so for another 85 million years. None of the great mountain ranges existed: no Rockies, no Himalayas, no Alps. But the Appalachian mountains were probably several hundred million years old.
The interval of time from the present to the opening of the Atlantic represents only about 3% of the Earth's 4.5 billion-year history. Yet the appearance of the Earth changes at a much more rapid rate. A round figure given for the general rate of erosion of the Earth's surface is 10 cm per thousand years. This is not much, in a human lifetime, but in a million years, it would amount to 100 meters, and in 150 million years, it would be 15 kilometers.
The surface of the Earth, geologically speaking, is quite recent. The layers for which Charles Lyell had a rather thorough grasp went back only about 65 million years. Of course, there were older rocks and older layers. The erosion rate of 10 cm per 1000 years is not uniform over the land. Even today, in some regions, pre-Cambrian rocks are exposed. But prior to the use of radioactive dating, little could be made of these fragmentary outcroppings.
What a contrast it is, then, to look at the surface of the Moon. There is hardly anything to be seen on the Moon that is less than a billion years old. Most features are between 2 and 3.5 billion years old.
Prior to the Apollo program, geologists had begun to map the moon using the technique of geologists. Basically, this technique groups features into relative time sequences.
Some geological time units are listed below. Most of the words in this table refer to materials whose ages may be found with the help of radioactive dating. Eras, periods, and epochs are sometimes called geological time units; chronological ages may be assigned to them. Systems and series refer to features called chrono-stratigraphic units. The words mean almostthe same as periods and epochs, and in this course, we will not distinguish among them. We would not have to mention them at all, except that the corresponding lunar units are called "systems".
Era Period Epoch Began(Million years ago)
(System) (Series)
Cenozoic Quaternary Holocene Present
Pleistocene 1.6
Tertiary ...
...
Paleocene 65
Mesozoic Cretaceous 145
Jurassic 208
Triassic 250
Paleozoic Permian 290
...
...
Cambrian 570
PreCambrian 4560
Detailed relationships in the Tertiary were pretty well worked out relative to one another in the time of Charles Lyell in the mid 1800's.
Geological features are mapped into a third category, called rock stratigraphic or lithostratigraphic units. The largest division of these is called a group, which may be divided into formations. We will not need additional subdivisions in this course.
There is no better place on Earth to illustrate these terms than in the Grand Canyon. Click here for a view of the Grand Canyon from the south rim with several geological formations indicated. Be sure to read the text beneath the figure. A geological formation is a body of rock or material, typically a layer that is distinct from nearby material, in appearance, texture, or composition. Often one formation may differ from another in its content of fossils. Formations are subdivisions of geological groups. An example in the Grand Canyon is the Supai Group, which you can view in with the help of this button. Follow the links to the Grand Canyon sites.
There are two famous lunar formations that we will discuss momentarily, the Fra Mauro and the Cayley formations. First, let us consider the mapping of the Moon into periods or systems.
Lunar systems were mapped before the Apollo missions returned samples that enabled them to be put into absolute time intervals for which the word "periods" is now more appropriate. Starting with the oldest system, we shall use (for this course):
System (or Period) Began about
pre-Imbrian 4.6 billion years ago (birth of moon)
Imbrian 3.8 " " "
Eratosthenean 3.2 " " "
Copernican 1.1 " " "
Note that the time intervals corresponding to the lunar systems are much larger than those of terrestrial systems (or periods). There is not much we can do about this. The pundits have used this terminology for some time.
The lunar systems are well delineated in the region near the southeast rim of the Imbrian basin. Check this figure, and the computer sketch below. Just coming off the Apennine Mountains (Ap) is the crater Eratosthenes. It has a rough floor, but no pronounced rays. Copernicus, on the other hand has a well developed ray system. The crater is just outside of the ("this figure") image, but its rays may be seen in the lower left (cf. Figure 18-2). It is now well known that the ray system of craters darkens with age, therefore:
The crater Archimedes (A) was clearly formed after the Imbrian event, AND after the subsequent flooding of the basin by basalt. We can see this because Archimedes has a flooded floor. Two smaller craters near Archimedes (o) are mapped in the Copernican system because of their rough floors, and presumably evidence (that is not obvious in the photograph) that they are younger than Eratosthenes.
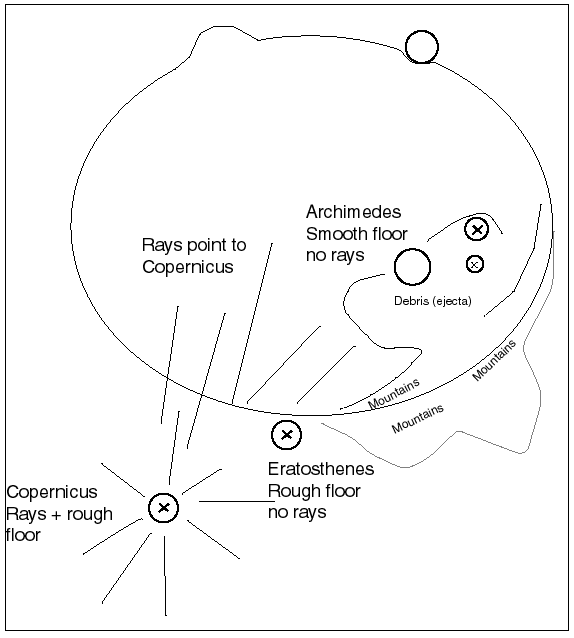
So it is easy to delineate the relative sequence of events near Imbrium. First, the event itself, which created a multi-ring basin. Archimedes was formed at some point after this event--otherwise, it would have been destroyed. The inner Imbrian rings are now covered by the subsequent basalt flooding of the basin, that also filled the floor of Archimedes. Eratosthenes (E) and still later, Copernicus (C) were formed after the flooding. You can see Copernicus and its rays on the false color moon.
A rather minor crater, Fra Mauro was used to name an important lunar formation. The link is to a NASA frame showing the site of the Apollo 14 landing (arrow) as well as Fra Mauro (centered). An instrument from the orbiting spacecraft extends from the left edge of the image.
Look also at an image of a region just to the northwest of Alphonsus. The crater Ptolemaus, which is the large crater in the highlands due north of Alphonsus is just visible in the lower right. On these shots one can see lineations that pointed to the center of the Imbrium basin. This material is believed to have been ejected in the Imbrian event, and it can be seen clearly in the region of the Fra Mauro crater. An critical part of the mission of the Apollo astronauts was to return rocks from this formation. It is in this way that the Imbrian event was dated (3.8 Billion years ago).
Another famous lunar formation is named for the quite insignificant crater Cayley. Cayley formation is best viewed in the bottom of some large craters in the highlands. Check out the crater Alphonsus, which is in the central highlands. Ptolemaus is just to its north (above), and Arzachel to its south.
At first glance, these craters seem to have been flooded similar to Archimedes. A closer look shows faint, underlying relief, rather than the sharp boundaries you would expect from filling by a liquid. The current theory is that this smooth material is debris that sifted down from above, often by material in ballistic trajectories ejected from other cratering events. You may imagine sifting sand over a child's sandbox. Eventually you would cover the caves, roads, and bridges, as well as the toy cars left in the box. But there would be a point when the surface would be lumpy from the objects beneath it. That's what the Cayley formation looks like. This material was first investigated near the crater Cayley, and is therefore called Cayley formation.
This implantation from above should be added to the two major kinds of processes that have altered the lunar surface, basaltic flooding, and cratering.
Planetary (and lunar) surfaces may be mapped in two general ways. One method simply groups areas with similar topography--areas that look alike. These areas are called physiographic provinces. On a smaller scale, one may consider the chemistry and petrology of natural materials and use the categories group or formation. In the case of terrestrial materials, groups or formations may not necessarily appear on the surface, but may be revealed by road or river cuts.
A second kind of mapping groups areas with similar ages. Again, if layers are revealed by road or river cuts, this same terminology may apply to them. The large time categories we use are eras, periods, and epochs. Lunar surface areas have been mapped into systems.
The surface of the Earth is much younger than that of the Moon. Terrestrial maps rarely show surfaces that date back more than a few tenths of a billion years. Plate tectonics and erosion rapidly renew the Earth's surface. We can tell the relatively younger surfaces by rays and crater densities. But virtually everything is a billion years or more old. When we view the lunar surface, we truly look deeply into past time.
Regions of a planetary surface that are similar in appearance (or chemistry) may be classified into groups and formations. Two important examples are the Fra Mauro and Cayley formations. Material from the Fra Mauro formation is associated with the Imbrian event, but the Cayley formation might have accumulated over a wide interval of time.
It is only on the Earth that we may travel to a geographical site, collect a rock specimen, and get it dated in a laboratory. Only a highly selected sample of lunar materials have been dated in this way, from materials collected some decades ago. For the many other fascinating planetary and satellite surfaces of the solar system we have only one other method of establishing dates, crater counts.
We must begin with the lunar surface. The Earth's surface is entirely too young to be of much use in this procedure. First, it is necessary to assign chronological ages to regions of the Moon. This has been crudely sketched out in Table 18-2, and we need not refine it here. The next step is to investigate the nature of the cratering process, and its record on a planetary surface.
Since the craters are made by infalling meteoroids, the size of the craters is related to the size distribution of the meteoroids. You might expect that there would be more small bombarding objects than large ones, and the surfaces of the planets and satellites certainly reflect this. Consider a plot of log(N(D>) vs log(D), where N(D> is the number of craters per square kilometer with diameters in a range about D. Such plots are typically straight lines (#):
x*
|# x*
log(N ) | # x*
D | # x*
| # x*
| # x*
--------------
log(D)
As the surface ages, the crater densities will increase (x). When new craters obliterate old ones, the surface saturates and the curve moves very slowly to the right (*), and eventually doesn't change at all.
If some phenomena such as flooding destroy the craters, the small ones tend to be destroyed first. Such a process could be basalt flooding, as in Imbrium. Many of the smaller craters were destroyed, but remember that Archimedes survived. On Mars, flooding by water removed many of the craters. This could reset the crater density clock for the smaller craters. The plot above might change to look like the one below.
|*
log(N ) | *
D | * *
| *
| *
--------------
log(D)
There are many examples of such distributions of crater sizes. They give the possibility of obtaining two dates, an older one from the larger craters, giving the pre-flooding age, and an age for the flooding, based on smaller craters.
With the help of the known dates of Moon rocks, it is possible to draw up a calibration curve for such craters. One example of such a curve is show in Figure 20-1.
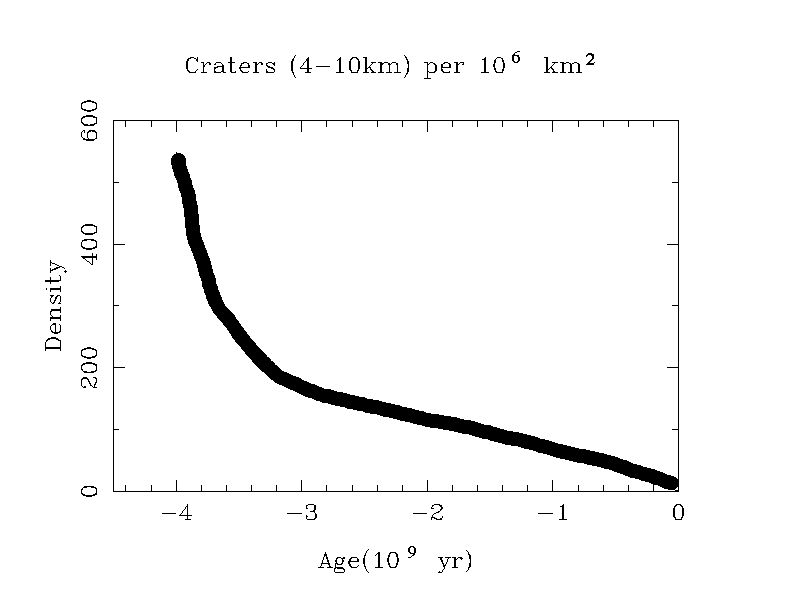
In order to make a plot like Figure 20-1, it is only necessary to count the number of craters within a 4 to 10 km diameter range within some area on the Moon's surface. The counted number then gets scaled up or down to what it would be if the area were a million square kilometers. If the age of the surface is known, say from Table 18-2 (or perhaps a more detailed version of it), we have one point on a plot like Figure 20-1. Other points come from counts of other regions of the Moon's surface.
This procedure may be repeated with craters in other size ranges. In the end we have a recipe for dating any cratered surface. It is only necessary for us to make an assumption about the relative cratering rates on other planets relative to the Moon. The simplest assumption we can make is that it is the same, and then we can use the date from the original plot.
The planetary scientists who have employed this technique have used various assumptions to refine the method. Some have assumed that cratering rates would be higher for objects nearer the asteroid belt. Others have applied corrections to surfaces they thought were near saturation, that is, where craters had begun to destroy those that had preceded them in time. We will not consider those details here.
One obvious conclusion to be drawn from Figure 20-1 is that the cratering rates at the Moon were much higher farther back in time. Note how the crater density increases much more between 3 and 4 billion years ago than between 2 and 3. This is what we would expect if the Moon were being formed by the accumulation of meteoroids or planetesimals. Toward the end of the buildup of these bodies, the supply of planetisimals starts to run out.
Much of the early history of the Earth has been erased by erosion, and the phenomena known as plate tectonics or continental drift. But modern work has added much to our understanding of the Precambrian, which is now commonly divided into the Hadean, Archean, and Proterozoic eons. We shall not need those subdivisions in this course.
It is well established that continental regions on the surface of the Earth have moved relative to one another, forming supercontinents, and then breaking up again. Oceanic crust has been destroyed by these motions. It has been driven down into the mantle in what are called subduction zones. Because of their lower density, some continental regions have survived for a considerable fraction of the Earth's history. The oldest terrestrial rocks that can be dated have ages of about four billion years.
If we try to push beyond the record that can be read from the oldest rocks, we must rely heavily on theory. The age of the solar system itself derives from dates of meteorites. The oldest values are about 4.6 billion years. We think that the sun, the Earth, and the other planets formed some tenths of a billion years after this.
The Earth formed by the accumulation of solid material. A few solid presolar (q.v.) grains were present in the solar nebula, and never melted. We will discuss them in Lecture 35. The majority of matter from which the planets formed was gaseous at the earliest times. It is likely that some solids condensed from the nebula on these presolar grains, in much the same way that rain droplets form on dust particles in the Earth's atmosphere today. As more solids formed, the condensation centers collided, and in some cases stuck together, eventually forming planetesimals. These continued to sweep up solids, forming the terrestrial planets.
The last solids swept up are responsible for the rapid increase in the density of craters on the oldest planetary surfaces. A similar rain fell on the Earth, but evidence of it has long since been erased.
A major feature of the Earth's structure is its nickel-iron core. We are not entirely sure how this formed. Possibly the core formed first, and the mantle accreted about it. This is not the favored theory today. Most experts think that the original material from which the Earth formed was a jumble of solid matter, not unlike that which occurs in many chondritic meteorites--rocky minerals interspersed with metallic, or reduced nickel-iron. If this is the case, the core had to form after the Earth had accumulated to the point that heating was important. One source of heating is radioactive decay.
We think, from the fact that there are iron meteorites, relatively small bodies could be heated sufficiently to form cores. The asteroids are often discussed as possible meteoritic parent bodies. The largest of these, Ceres, is only about 0.3 times the size of the Moon. Small bodies cool quickly, indeed, one can show that the cooling time goes as the square of the size. If these bodies generated sufficient heat to form a core, more powerful sources of radioactive heating would be required than the 40K, uranium, and thorium that are active today.
There is good evidence that the unstable nuclide 26Al was present in the early solar system, and it is often mentioned as a possible heat source for smaller bodies. Its half-life is 7 x 105 years, so it provides energy at a much higher rate than 40K, uranium, and thorium. Their relevant half-lives are of the order of a billion years.
If the Earth's core formed from little specks of iron that melted and then sank, this process itself is a source of considerable energy. One may readily calculate the difference in energy of a homogeneous body the size and density of the Earth. This energy may be compared with a Earth-like sphere with a metallic core and rocky mantle. This energy would be released during core formation, and should be more than sufficient to totally melt the Earth. However, since we do not know how the core formed, we cannot say how rapidly this energy was made available.
The heat released by the radioactivities or by core formation could have readily cooked out volatile materials within the body of the planet. We are not really sure how the Earth got its volatiles, the air and water so necessary for life. The Earth's crustal abundances (see also Figure 20-2 below) show a marked depletion of the noble gases, and the heavier of these could not have simply ``boiled away.'' It is most probable these and other volatiles were never present as a part of an early atmosphere.
There are two possible explanations of the current source of the Earth's volatiles. They were either cooked out of the interior, or they were brought in by volatile-rich comets. Both mechanisms must have acted to some degree, but we cannot be sure which dominated.
If volatiles were cooked from the interior, they had to be brought in by the planetesimals that formed the bulk of the planet. We can be relatively certain that water, CO2, and N2 were gaseous at the time of the accretion of the Earth. Some gaseous material could have been attached to the surfaces of the accreting solids by the weak chemical bonds. The process is called adsorption. Other gaseous molecules might have been enclosed inside porous solids.
Comets, on the other hand were probably formed beyond the snow line where CO2 and certainly water had condensed as solids. The main problem with using them to bring in the volatiles is that it is hard to predict their infall rate. One theory of comets supposes that most of them were originally in the inner solar system, and they were thrown out by perturbations mostly from Jupiter. If this is the case, most comets were headed away from the Earth, with a small residual left to bring in the volatiles. This residual is difficult to calculate.
The Moon's mean density is 3.3 times that of water. This is significantly less than the density of the Earth, 5.5, so it has been known for a century or so that the compositions of the Earth and Moon are significantly different. It is rather easy to account for the Moon's low density by assuming that it lacks an iron core. Why might this be?
A theory of the origin of the Moon that was popular in the early 20th century was that the Moon was torn from the mantle of the Earth after the core had formed. This might account for the density, but the mechanism by which tidal forces deformed the Earth, and separated it into two masses is no longer considered tenable. In addition, there are important differences in the chemistry of the Earth and Moon that were revealed by the Apollo program.
Most discussions of lunar rocks point out that they lack the hydrated minerals common in terrestrial crustal rocks. There is no liquid water on the Moon from which such minerals might have formed, and almost certainly never has been. Whatever processes brought the Earth its volatiles were not efficient for the Moon.
Another aspect of the lunar rocks that differentiates them from the Earth's crustal rocks, or even our estimates of the composition of its mantle rocks is the fraction of metallic iron in them. While native or metallic iron is rarely found in terrestrial rocks, it is a ubiquitous minor phase of lunar materials. If the Moon had been torn from the Earth's mantle, we would expect less metallic iron.
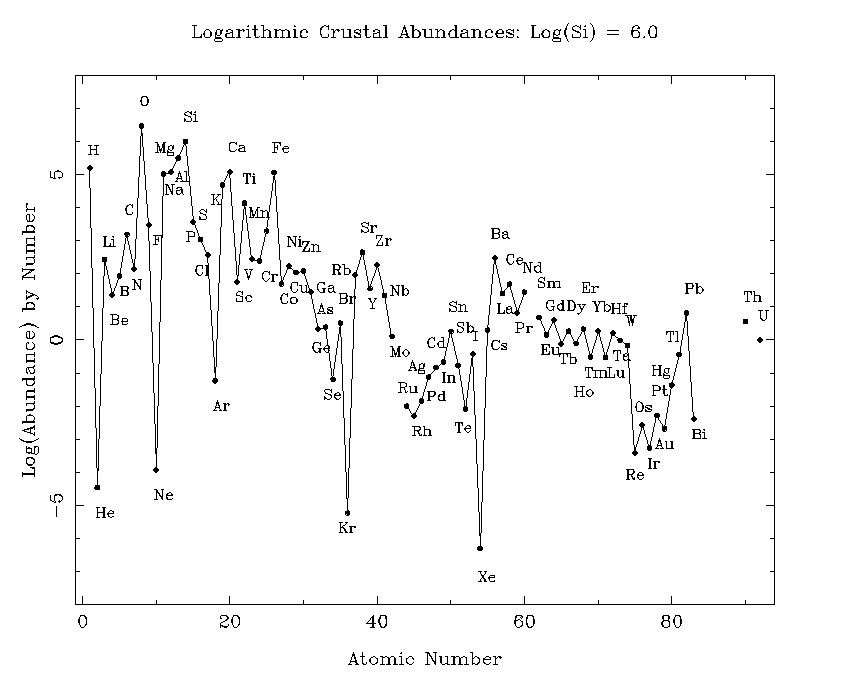
There is, however, an intriguing similarity in the lunar and terrestrial rocks, and that is a depletion of the so-called siderophile elements. These are the elements that may be seen in troughs in a plot of crustal abundances. The best defined of these troughs is that of rhenium (Re) through gold (Au). There is a comparable trough involving ruthenium (Ru) through silver (Ag). These elements fall in analogous positions in the periodic table. Siderophile means iron-loving. These elements had a strong tendency to follow iron into the core.
The Australian geochemist A. E. Ringwood described a model for the formation of the Moon that would account for its chemistry. In his picture late stages of bombardment of the Earth by meteoroids would actually vaporize some fraction of the mantle, boiling off a ring of material which could later recondense and form the Moon.
In Ringwood's picture, lunar material would have thus been subject to two condensation epochs, one when the Earth was formed, and a second epoch leading to the formation of the Moon itself. In the second epoch, additional volatiles could have been driven away by the solar wind. In addition, free oxygen is presumed less prevalent during the second condensation than when the bulk Earth accreted, which would account for the free iron in lunar rocks.
It is generally thought that mantle rocks are predominately olivine, mostly Mg2SiO4. In lunar rocks, the dominant minerals are pyroxenes, e.g. Mg2Si2O6, richer in SiO2. Ringwood's picture accounted for this by having SiO2 boil off preferentially when bombardment had raised the temperature of the Earth's surface to some 1500K.
This picture was designed to account for the lunar chemistry, not surprisingly, because Ringwood is a geochemist. He dealt less convincingly with a problem connected with the dynamics of the Moon's orbit.
The Moon's orbit does not lie in the equatorial plane of the Earth. In fact, it is nearer to the plane of the Earth's orbit, the ecliptic plane, although it is slightly inclined to that as well. If the Moon formed from recondensed materials that had boiled off the Earth, its orbit would almost surely have been in the Earth's equatorial plane.
Ringwood in fact suggested that one or more large impacting bodies, in the latest stages of the overall process of lunar formation might have knocked the accreting Moon from an equatorial orbit. Modern ideas take this one step further, and postulate that the entire process of lunar formation was dominated by a late impact on the Earth of a body with the approximate size of the planet Mars.
The theory of lunar formation by a giant impact was proposed about 1976 by A. G. W. Cameron and others. The giant impact theory is now widely accepted, and is described in considerable detail in elementary astronomy texts. There is a 1996 semipopular book about the Moon by Paul Spudis, of the Lunar and Planetary Institute in Houston, in which this theory is called the ``Big Whack.'' Perhaps this view of the formation of the Moon has been accepted without due caution.
The Big Whack theory of the Moon's origin can readily account for the fact that the orbital plane does not coincide with that of the Earth's equator. Presumably, the impactor had an orbit near to the plane of the ecliptic, and the present Moon's orbit still ``remembers'' this.
In the scenario often illustrated in textbooks, the impact took place very early in the Earth's history, but after both objects had formed cores. Most of the moon comes from the mantle of the impactor. The core of the impactor eventually merges with that of the Earth.
The Moon then forms from the mantles of the impactor and the Earth. Opinions differ about how much of the current Moon came from each possible source. Most of the Moon probably was once a part of the impactor. This helps to account for geochemical differences between the Moon and Earth. The mantle of the impactor, plus some fraction of the Earth's mantle become vaporized as a result of the collision, and recondense. During the condensation phase, volatiles may be driven off in a recapitulation of the mechanism of formation of the terrestrial planets. This picture is much the same as in the earlier theory discussed by Ringwood, and accounts for the dryness of the lunar rocks and soil.
While there are significant geochemical differences between the terrestrial and lunar rocks, there is one intriguing similarity. Ratios of the isotopes of oxygen show no differences. Oxygen has three stable isotopes, 18O, 17O, and the dominant isotope, 16O. For reasons that are not well understood, the relative abundances of these isotopes appear to be characteristic of different regions within the solar system. The atmospheres of Venus, Earth, and Mars, as well as different kinds of meteorites all have distinct ``signatures'' of oxygen isotopic abundances.
Lunar and terrestrial rocks have the same oxygen isotopic signature. How can this be if the Moon formed out of material from a foreign body? Advocates of the Big Whack hypothesis usually say that the impactor must have formed near the Earth. This is neither probable nor impossible. It is not probable because the Earth could have readily swept up materials that would have formed the other body. It is not impossible because we do not know the precise conditions of the accumulation of the Earth, and cannot say how improbable assembly of the putative impactor near one astronomical unit really was.
Does the Moon have a core? Shortly after the Apollo missions, there was much discussion of the Moon's core, as a result of a 1972 seismic event. Until results from the Lunar Prospector, a mission launched in early 1998, there was little to confirm the existence of a lunar core. But in March of 1999, mission scientists announced that their analysis of gravity and magnetic measurements indicated a core some 200 to 400 km in radius. According to a press release, the existence of the core supports the idea that the Moon was formed after the impact of a Mars-sized object. In this picture, the lunar core would derive mostly from the impactor. In earlier scenarios, the core of the impactor merged with that of the Earth.
For the present, the Big Whack hypothesis is the best idea to date for the origin of the Moon. We still have much to learn.
After the Moon had formed, kinetic energy from infall or short lived radioactive sources supplied enough heat to melt all or most of the body. Anorthite, because of its low density and high melting temperature, crystalized from the melt, and floated upward, to form the anorthositic highlands. The last few large impacts formed the major basins, ending with the Imbrian event. A few hundred million years later, longer lived radioactivities provided enough heat to melt and flood the major basins that we now see as maria.
Compared to the Moon, the surface of the Earth was created "yesterday." The Earth probably formed from the accumulation of planetesimals containing both metal and rock, but relatively few volatiles. The core probably formed after sufficient heat was released by radioactivities for the iron to sink through softened or melted rock. Elements that followed iron into the core are called siderophiles. Additional heat was released when the core formed. Heat from the Earth's interior drives surface plates. These and erosion ensure that features at the Earth's surface are rarely more than a few hundred million years old.
The Moon formed in orbit from the debris of a "Big Whack." The highlands formed when anorthite floated upward in an early, mostly melted body. After the crust solidified, the major impact basins were formed. They flooded, some hundred million years later.
Virtually all lunar features are older than a billion years. Lunar systems can be dated by the principle of superposition. A powerful application of superposition involves counting crater densities. The densities can be converted into ages using dates for rocks returned by the Apollo missions.
The Discipline of thermodynamics was one of the triumphs of nineteenth century science. Its name indicates that it has something to do with heat, or energy. One may take a broader view, and include such diverse matters as information, but we shall not do so here. Instead of attempting a general description of thermodynamics, we shall describe some specific situations that illustrate its principles. Ultimately, thermodynamics rests on laws, or postulates. We may justify them in various ways, but there is no rigorous, logical proof.
Suppose you had two large boxes of gas. We might suppose the gas is inert, like helium or argon. It is simplest if we think of an ideal gas, a material that does not exist, but is approximated for many purposes by inert gases. Let us suppose that both contain the same number of molecules but that one of the containers is hotter than the other. The pressure would then be higher in the hotter container too. We could put the boxes in contact with one another, and open a door between them. Molecules from the hot box would stream into the cold one. We could put a paddle wheel in the stream, and use it to generate electricity, or wind a weight, or do a variety of other things.
Eventually, the gas in both boxes would reach the same temperature, pressure, and number of molecules. At this point, there would be no way we could use a paddle wheel to do any of the things we mentioned above, nor any other useful work. Although there would still be lots of energy in the boxes that had reached an equilibrium, we couldn't do any work with them.
The system with the two boxes at different temperatures has the ability to do work, while after the temperature between them has equalized, this capacity is no longer present. The German physicist Rudolph Claussius (1822--1888) proposed that the inability of a system to do work could be considered a property of the system. If we picture our system as a box of gas, then familiar properties would be temperature, volume, and density. Claussius's new property was given the name entropy, according to the popular science writer Isaac Asimov, ``...for no clear etymological reason''!
Entropy is measured in a funny way, that makes sense only after some study. Since it measures the inability to do work, you might expect it to be zero for the two-box gaseous system, after it has come to temperature equilibrium. But it turns out that entropy is measured in a way that it reaches a maximum for that temperature equilibrium. So we measure the ability of a system to do work by considering how far the its entropy is from its maximum value.
Ludwig Boltzmann (1844--1906) clarified the notion of entropy by postulating that it was a measure of the probability of the system. Consider our two boxes at different temperatures just after we open the door between them. According to Boltzmann's ideas, the configuration with a high temperature (Th) in one box and a low one (Tl) in the other would not be very probable. Therefore, the initial entropy of the system would be relatively low. Then the system would adjust until the gas in both boxes had the same temperature. Boltzmann was able to show from the kinetic theory of gases that the state with the temperatures equal was much more probable than the original one. Then, the way entropy is defined, the entropy was higher.
We are now in a position to state the three laws of thermodynamics. Laws one and three turn out to be much simpler to state than the second law, sometimes known as the law of increase of entropy. Most of this section will be spent with it.
The first law is sometimes called the conservation of energy. We can state it in a useful way with the help of a specific example. Suppose we dump some energy inside a balloon that is filled with an ideal gas. The first law then states that the energy of the gas will increase, but some of the energy must go into expanding the balloon. Therefore, at the end of the energy transfer, the gas will not be as hot as it would be if, for example, the gas were in an insulated box with a fixed volume.
Whenever energy is converted from one form to another, such as from heat to work, the first law just says we must be careful to consider all of the possible forms.
The second law deals with entropy. It says that all naturally occurring processes cause the entropy of a (closed) system to increase. Consider our two boxes of gas. Just before we open the door, there is a value for the entropy of the box with temperature Th, and one for the box with temperature Tl. It turns out that the entropy of the hotter box is higher than that of the cooler box, but we don't need to worry about that here. The second law tells us that after we open the door, and the temperature equalizes, the entropy of the two boxes is greater than the sum of the initial entropies.
My introduction to thermodynamics was in 1953, when I took a course in physical chemistry at the University of Virginia. My lab partner said the second law was easy---it just said water ran downhill. While this is a considerable simplification, it is also very useful. We need to use the concept of potential energy, which we discussed in Section 4.8. Water that is uphill has a potential energy that is converted into kinetic energy as it flows downhill. Since this is a natural process, the second law implies that ``downhill'' water is more probable than ``uphill'' water. This is entirely in line with what we would expect.
Suppose we placed a droplet of water at the lip of a bowl. We would expect it to slide to the bottom, probably move uphill a little but end up right at the bottom. If there were no friction between the droplet and the bowl, the droplet would oscillate forever (assuming it didn't evaporate). We know that friction is a part of the real world, so the water would end up at the bottom of the bowl. The potential energy the drop had at the top of the bowl would be converted into heat, and the bowl would be just a little hotter than before the drop did its thing.
According to the second law, the entropy of drop + bowl would be higher at the end of this process than at the beginning. It shouldn't be too difficult to convince oneself that of all the possible things that might happen to a drop of water at the lip of a bowl, the most probable is that the water flow downhill---as my lab partner said.
As far as we know, there is no violation of any law of the elementary interaction of particles that says heat might be extracted from the molecules in the bowl and deposited in a small puddle of water in just such a way that the puddle would climb up the side of the bowl! Common sense tells us that this would not happen. Thermodynamics tells us something a little different. It says that it is over-, over-, over-, overwhelmingly more probable that the drop will settle at the bottom of the bowl than that it would spontaneously extract the kind of energy from the bowl to do the opposite thing.
Very simple physical systems behave in a way that doesn't depend on the direction of time. Consider the planets as just points, moving around a featureless, smooth sun. This is a simple system. We could make a movie of the system, and it would look natural whether we ran the film forward or backward. When there are many particles interacting in a complex way, we could tell immediately whether the film was being run forward or backward.
Consider the water drop and the bowl. The number of molecules involved in both the drop and the bowl are many powers of ten. There would be 2 x 1019 water molecules if the drop were one millimeter in diameter. For matter involving large numbers of particles like this, time does have a unique direction. Time goes forward in such a way that the entropy of an isolated system will increase. Technically, this direction for time has only statistical validity. Practically, it is so overwhelmingly more probable that the entropy of a complex isolated system will spontaneously increase. There is only one exception, when the entropy has reached its maximum value. We couldn't use an isolated system that had reached its maximum entropy to tell time. This does not pose a problem for the world we live in.
Given our definition of entropy as a measure of the probability, we now briefly state the third law. At absolute zero, the entropy of pure crystalline substances is zero. This postulate allows chemists to make calculations of the entropy of substances from thermochemical measurements. With the zero point defined by the third law, such entropies are called absolute entropies. Another way to calculate them for simple systems is with the help of Boltzmann's definition of entropy in terms of probability. In practice, absolute entropies are often not needed because we can tell the ``downhill'' direction from changes in entropy.
In astronomy, we often have a temperature and pressure that is fixed by our model, and we want to know the relative amounts of chemicals that could be present. Chemists often have a similar problem. They mix two chemicals at the ambient temperature of their laboratory, and they want to know if they will react. Under these circumstances, it turns out to be more convenient to look at another thermodynamic property of a system known as the Gibbs energy.
The Gibbs energy is related to the entropy, but there is a negative sign. Consider a system with energy (E), volume (V), and entropy (S). Let us suppose the pressure (P) and temperature (T) have fixed values. Then the Gibbs energy (G) may be defined as G = E + PV - TS. Since we are trying to avoid equations in this book, we shall only point out that the minus sign leads us to expect the Gibbs energy to decrease for spontaneous processes, since the entropy must increase by the second law.
The energy and PV-terms in the definition G make it simpler to use it than the entropy when a system might have to do work against a constant pressure. We could show this explicitly with a few equations. We will try to make this plausible below.
Consider a chemical reaction to form the simple diatomic radical CN. It is convenient to think of the two atoms as well as the molecule as being in the gas phase, (g).
In order to see if the reaction will proceed at a fixed temperature and pressure, we calculate the Gibbs energies for the substances on both sides of the arrow. We would do this for more complicated chemical equations too. If the Gibbs energy of the products (on the right) is lower than the Gibbs energy of the reactants (on the left), then the second law tells us the reaction will go from left to right as long as the temperature and pressure remain fixed.
On an atomic level, it is useful to look at the formation of this molecule with the help of a potential curve, similar to the ones we have used in looking at the behavior of one dimensional motion in the two-body problem.
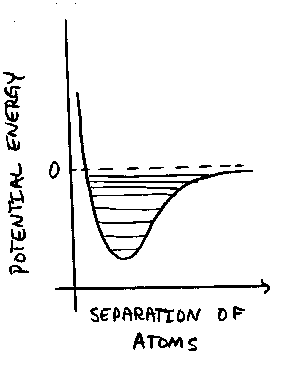
In this plot, the vertical axis gives the potential energy as a function of the relative separation of the two atoms. The minimum of the curve shows the most probable separation of the atoms in a bound molecule, at low temperatures.
If we think of the potential curve of Figure 21-1 as representing a classical system, then a marble placed on the curve would roll down the hill toward the minimum. We might think of the minimum as the most stable position, as we did for the water drop in the bowl. But the CN molecule is a very simple system, and as yet, we have no analogue of friction. So the ball would roll out of the minimum, toward even smaller separation, come to a halt at the same vertical position it started with, and roll back the way it came.
We have had very similar behavior in the discussion of another two-body problem, that of a planet and the sun.
For the CN molecule to form, something must remove the relative energy with which the two atoms approached one another. This energy could be removed by the emission of a photon, or by a collision with a third atom, which could take up the excess energy. Given that these possibilities exist, we ask whether for a given temperature and pressure the CN would form. The answer depends on the values of the temperature and pressure.
Intuition tells us that if the temperature is high, the molecule is more likely to dissociate than form. The pressure is also relevant. In elementary chemistry we learned a useful rule called the law of mass action. That law said that if you stressed a system, the system would try to remove the stress. For example, if you increased the pressure of either C or N, the system would try to form the CN molecule to decrease the pressure. Conversely, if the pressure of C and/or N decreased, it would be more likely that the CN would dissociate.
There is another way to look at the effect of pressure. Pressure depends on both the temperature and the number density or the number of particles per unit volume. So if we fix the temperature, the pressure will increase or decrease directly with the number density. Clearly at high number densities, the atoms of C and N will collide more frequently with one another, and have the opportunity to form CN.
If CN dissociates, it forms two atoms, so there are two particles where previously there was only one. At a fixed temperature, which we assume, the two atoms supply exactly twice the pressure of the molecule. This is not intuitively obvious, so we must make a mental note of it. In an ideal gas at a fixed temperature, the pressure depends only on the number density (particles per cm3) of particles, not on the mass of the particles.
Since we also assume a constant pressure, the increase due to the extra molecule must be removed in some way, and that is done by an expansion of the gas. This is equivalent to doing work against pressure. When CN dissociates, we need to consider this extra work in addition to the energy it takes to roll the ball up the hill in Figure 21-1 It is this extra work that is properly figured in the Gibbs energy for the constant temperature and pressure processes. Such conditions usually turn out to be of primary interest, both in laboratory chemistry, and astronomical applications.
Suppose the differences in the Gibbs energies of the two sides of a chemical equation is zero. The reaction can then proceed in either direction with equal probability. The influence of pressure that we discussed in the previous section now plays a key role. With the pressure fixed, it turns out to be possible to calculate the relative amounts of the reactants and products in a chemical equation.
Let us consider an especially simple reaction, where iron in the gas condenses, that is, becomes solid iron. We may write
where the `g' and `s' stand for gas and solid. The `reaction' is really only a phase change, but the laws of thermodynamics still apply. In this case, the pressure of gaseous iron is a function of the temperature only. Note that this is what the chemists call the partial pressure of iron, that is, the pressure that would hold if iron were the only gaseous species. The total gas pressure is the sum of all of the pressures of atoms and molecules in the gas phase.
There will always be some iron in the gaseous phase, no matter how low the temperature drops, but there is a relatively narrow temperature range where the pressure in the gas phase drops very rapidly, and for temperatures below this range, it is a good approximation to assume that all of the iron has passed into the solid phase. Workers in this field often take the temperature at which the partial pressure has dropped to half of its original value, and call it a 50% condensation temperature.
The vertical axis gives the partial pressure of gaseous iron. Temperature is plotted on the horizontal axis, increasing to the right. A straight line divides regions of the plot where gaseous and solid iron are the dominant phases.
We can make a plot of the vapor pressure of iron as a function of temperature. For each value of the vapor pressure, there will be a temperature where half of the original vapor has condensed. The plot of Figure 21-2 is made so that a straight line on the plot divides the region where the iron is primarily in the gas phase from the one where it is primarily solid. The figure shows that the condensation temperature depends on the partial pressure of gaseous iron. The law of mass action is a useful mnemonic here. If we consider a fixed temperature, then we would expect a high gas pressure would drive the ``reaction,'' Fe(g) ----> Fe(s), to the right. So the region where the solid phase dominates is above the dividing line of Figure 22-2.
Calculations of condensation temperatures have mostly been carried out for cooling gases with the composition of the SAD. They show that the first appreciable solids to form are oxides of aluminum, calcium, and titanium. Two of these oxide are the minerals corundum (Al2O3), and perovskite (CaTiO3). Materials that form solids at the highest temperatures are called refractory, while those that do not enter the solid phase until the temperature is low are said to be volatile.
Corundum and perovskite are thus said to be refractory oxides. Their properties of early condensation is why they are so important, and why we stressed them in our classification of minerals in Lecture 14.
The temperature at which a chemical element comes out of the gaseous phase depends critically on the compounds that it forms. Few elements condense as pure species, as we have indicated for iron. Detailed calculations show this is not a bad approximation for iron itself, but for an elements like aluminum or calcium, the assumption would be badly off. Both of these elements come out of the gas phase at high temperatures because they form refractory oxides.
It has nevertheless been the custom of workers in this field to assign condensation temperatures to elements with the understanding that these values depend on the overall composition assumed for the cooling gas.
Thermodynamics is the discipline that tells the direction of a chemical reaction. It is based on three laws. The first is the law of conservation of energy. The second is the law of increase of entropy. Entropy is a measure of the probability of a system, so the second law says any system will change naturally to reach its most probable state. For processes taking place at a fixed temperature and pressure, those that occur spontaneously do so with a decrease of the Gibbs energy.
With the help of thermodynamics, we can calculate the sequence of solids that condense from a cooling gas. Elements that condensed at high temperatures are called involatile or refractory. Those that remain in the vapor until cooler temperatures are reached are called volatiles.
In Lecture 4, we discussed briefly the formation of the sun and solar system. We are now in a position to discuss this in some detail. It is believed that stars form mostly in clusters. This is because most young stars are found either in clusters, or in looser associations, which could be the remnants of clusters. It is now possible to use infrared and microwave techniques to look into dense interstellar clouds, and see young stars in the process of formation. There is now strong evidence for violent winds, such as those invoked to dispel the complement of hydrogen and helium from the zone of formation of the terrestrial planets.
Astronomers have located several thousand giant molecular clouds in our Galaxy in which star formation is taking place. Enter "star formation" into a search engine on the web, and get more than you ever wanted to see on the topic! The infrared techniques pick up the stars in these regions, and the microwave observations allow observations of molecules. Much of the dust of the plane of our Galaxy must also be in these regions. The dust shields the molecules from ultraviolet photons from hot stars, which would otherwise dissociate the molecules.
Dust is thought to form originally in the atmospheres of cool stars. There is something of a chicken and egg problem with this dust. The GMC's require it, to shield the molecules, and assist in the formation of the most common molecule of all, H2. This molecule forms only very slowly in the gas phase, but if two H atoms can stick on a dust grain, they will hop around and find one another, and then hop off the grain. This is a very efficient way to make H2. But the dust itself can be destroyed by ultraviolet photons. So the picture is:
This means some dust must survive from one GMC to the next.
For many years, the basic mechanism for the formation of individual stars involved self gravitation. Essentially this worked as follows. Think of the protostar as a ball of gas. If the ball were diffuse enough, the pressure forces would prevent collapse. But if the ball were squeezed, perhaps by a pressure wave from an exploding star, then the temperature would have to go up to prevent a collapse. The collapse would occur because the law of gravitation is inverse square. One side of the star would attract the other with a strength four times greater if you squeezed the ball to half its size.
So the question was whether the temperature in the ball could rise fast enough to prevent the collapse. This is governed by mechanisms that heat and cool the gas. Astronomers computed what they knew of these processes and concluded that stars would collapse under their own self gravitation.
New observations have shown that young stars have violent winds and jets that are not a part of this simple picture. However, there is no good substitute, so we shall work with this old picture for the purposes of this course.
A collapsing cloud, like an ice skater who brings in outstretched arms, will rotate faster and faster. It is an illustration of the conservation of angular momentum. Collapse along the axis of rotation is not affected by the angular momentum, however, and the cloud collapses into a lenticular form, with the protosun at the center. The remainder of the cloud is called the solar nebula
It is entirely reasonable to assume that there would be a temperature gradient in the solar nebula--that it would be hotter closer to the sun than further away from it. The nebula would slowly cool, and as it did so, those chemical species that change from the vapor to the solid phase would do so.
Condensation schemes were calculated for the cooling solar nebula beginning with the remarkable work of Nobel Laureate Harold Clayton Urey (1893--1981). He simply applied the laws of thermodynamics that we have discussed in the previous sections to a cooling gas with the SAD composition.
We can simplify the outcome of his work as well as modern improvements of it with the help of three of our four ``elements'' from Lecture 4. Thermodynamics tells us which materials condense from a gas with the SAD composition as the temperature drops. The first solids are metallic, with a small admixture of refractory oxides. At lower temperatures, rocky materials solidify, followed by the ices.
Let us look at what happens during condensation. First, we need to recognize that solid material is essential in a potential well compared with the vapor phase. The more closely packed the material, the deeper the well. For just two atoms, A and B, we can think of the forces between them deriving from a potential curve that has the same general shape as that for the two-body problem. When the two atoms are close, there is a minimum, that corresponds to the bound atoms, that is, the molecule AB. You can't jam the atoms arbitrarily close, so there is a repulsion for very small interatomic distances. At very large distances, the potential goes to zero.
The forces between these particles are electrostatic and derive from inverse square forces, even though the net forces between neutral atoms are not inverse square. They are, in fact, derivable from the relevant potential curves. So the closer the atoms are packed, the deeper in the well the particles of matter are. This makes metal harder to break up than rock, which is harder to break up than ice. By and large, the deeper the potential curves, the closer together the atoms are, and the denser the chemical compound.
There is an important exception to the rule that the higher the density the tougher the material. The mineral anorthite is lighter than many rock-forming minerals, but it's constituent ions are very tightly bound. We discussed this in connection with the formation of the Moon's crust.
If two atoms approach one another from afar, there will generally be a positive total energy for the system. This means that they may approach one another, but then move apart. In order to stick in the well, the system must lose energy. It can do this by either emitting a photon, or bumping into another atom so that the third atom carries away the excess energy needed for the pair A+B to be caught in the well. Photons are the practical way for the atoms in the early solar system to lose energy, since that energy can escape to the dark sky. When a system A + B loses energy to another atom, say C, that atom gains energy. It then has sufficient energy to disrupt a system AB, so that overall, no progress has been made in forming molecules, and eventually solids.
Mostly, liquids are not important in astronomical bodies. We shall discuss some very significant exceptions, or course, but throughout the cosmos, matter is usually gaseous or solid. Much of it is a plasma, that is, an ionized gas.
Urey thought the condensation sequences provided a theoretical basis for understanding the densities of planets at various distances from the sun as shown in Table 4-1. In his original theory, there would be a different density for each distance from the sun. This density could be derived from the pressures and temperatures in the solar nebula. Urey pointed out that the decompressed densities should be used in this comparison, since the solids in the solar nebula formed first under low pressures.
There are two models for the formation of planets from solids that condense out of the gas. They are called homogeneous and heterogeneous accretion. By accretion, we just mean the coming together of the solids to form the terrestrial planets.
In heterogeneous accretion, the first material to form at the position of a planet will build up a central protoplanet. Then as the gas cools, material of different composition will accrete on top. In the case of the Earth, the iron core would form first, and then the rocky mantle would accrete on top of it. This is called heterogeneous accretion because the Earth would be heterogeneous in its composition, as it is, in fact, today.
Solid particles which form as a result of cooling of the gas in the solar nebula would be able to react chemically with the residual gas. Iron dust, for example, could oxidize to rust. If the iron quickly condenses into a sizable ball, then only the outside could rust, and most of the iron would be unaffected. The number of atoms on the surface of a large spherical ball are negligible compared to the total number of atoms. (Can you explain why?) This is the picture of heterogeneous accretion.
In homogeneous accretion it is assumed that the solids are in the form of a fine dust that can always react with the residual gas if the temperature is right. If this is the case, a planet can be formed from material that is initially chemically homogeneous. Actually, even in this case, we picture fine needles of iron mixed in with silicates, but so that a box taken from any part of the accumulating Earth would have the same overall mix of rock and iron. In homogeneous accretion, we picture the core forming well after the Earth has accumulated to its present size, after sources have heated it so the iron can melt, and sink.
It is unlikely that either pure homogeneous or heterogeneous accretion took place. Some mixture of the two is more likely. However, the densities predicted for the planets with either hypothesis are quite similar. The exact percentages to these two models of accretion represent a detail that remains to be worked out.
The basic condensation model dominated thinking about the early chemistry of the planets---especially the terrestrial ones--for several decades. It had long been granted that the Jovian planets may have formed largely as a result of their own gravitation. For Mercury through Mars, and perhaps even for some asteroids, condensation seemed the ideal tool to understand the bulk densities. There was a problem understanding what happened to the matter that did not condense, but it was assumed a vigorous wind from the young sun could remove the uncondensed material.
Even as the most detailed condensation calculations were being carried out, evidence began to accumulate that seemed to contradict the notion. Space probes were able to sample the atmospheres of Venus and Mars. Certain meteorites were identified with reasonable probability as being fragments of the planet Mars or the asteroid Vesta. Many of these observations showed that there was no firm relation between the volatility of material and its location in the solar system.
A pillar of the condensation theory has always been the relatively high bulk density of Mercury. If this is not due to condensation at a high temperature, how might it be explained? A popular idea that persists since the 1980's is that Mercury once had a structure and composition similar to that of the Earth and Venus. These twin planets have rocky mantles and metallic cores that start about halfway down toward their centers. In the case of Mercury, it is possible that much of the rocky mantle was blasted away by meteoroid impact.
We have known since the Mariner missions in the 1960's that meteoroid impacts have scarred the faces of Mars and other solid planetary surfaces. Geochemists realized that the earth must have grown by the accumulation of relatively small solid bodies because of the absence of heavy noble gases in its atmosphere. These gases, argon, krypton, and xenon are much too heavy to have simply boiled off into interplanetary space.
Throughout much of the twentieth century astronomical textbooks used this mechanism---boiling off---to explain why the Earth and terrestrial planets did not have their SAD complements of hydrogen and helium. It is rather easy to show that most of these two light gases would leave the present Earth's atmosphere. But it was never very clear how such demonstrations would apply to a hypothetical body that once had all of the hydrogen and helium that would complement the Earth's metal and rock. Recall that about 2% by mass of the SAD is in elements other than hydrogen and helium. Consequently, a proto-Earth might have been 50 times its present mass, not as massive as Jupiter or Saturn, but more massive than Uranus and Neptune. It is at least problematical whether such a body might have lost its hydrogen and helium.
The geochemists had it right all along. The Earth and terrestrial planets never did have their full complement of hydrogen, helium, and other volatiles from the SAD because they formed from small solid bodies mostly of metal and rock. In the heyday of condensation, it was thought the terrestrial planets had the composition of solids that could condense at various distances from the sun. Now, it seems any given inner planet might have been formed from meteoroids and planetesimals from a wide range of radii, perhaps stretching to the ``snow line'' some 3 to 4 AU from the sun.
It is now possible to follow by computer the motions of a large number of planetesimals. The American planetary scientist George Wetherill has made steadily improving calculations of this kind. He follows the paths of these bodies, and has plausible formulae to decide when a collision will result in a larger object or smaller fragments. He has made models of planetary systems that could form from the coagulation and fragmentation of such bodies, and some resemble the present solar system.
In the late 1970's two hypotheses involving the impact of a meteoroid or planetesimal on the Earth became well known. Possibly the better known of these was for a relatively recent impact, some 65 million years ago. The geologist Walter Alvarez and his father Louis suggested that the extinction of the dinosaurs might be related to the aftermath of the impact of a large meteoroid. This hypothesis is now widely accepted, but with some reservations, because the extinctions could be shown to have taken place both before and after the impact. A great deal has been written on dinosaur extinction, so we need not pursue the matter here.
The other important development involving a giant impact was the "Big Whack" hypothesis for the formation of the Moon. We discussed it in Lecture 19.
Today, meteoroid and planetesimal impacts are being explored as relevant to the chemistry of planets in many ways that were not mentioned several decades ago. Perhaps a majority of planetary astronomers now prefer the ``blasting of the mantle'' hypothesis over condensation as the explanation to Mercury's high density.
Does this mean that Urey's idea was wrong? Most workers are unwilling to abandon the notion completely. Surely processes in the early solar system were more complex than in the simplest equilibrium condensation model explored by Urey. But the planetesimals that formed the terrestrial planets were basically of metal and rocky compositions. And it is very tempting to conclude the lack of icy (volatile) material was due to condensation at a relatively high temperature.
We now know stars are forming in the regions of Giant Molecular Clouds (GMC's). Details of the formation of individual stars have eluded us, as observations become ever more complex. Here, we assume an old picture of self gravitation. The residual gas collapses to a lenticular shape as a result of angular momentum. This is the solar nebula. As this gas cools, solids form. The first solids are generally the more dense. This is the explanation for the (decompressed) density decrease from mercury through Mars advocated by Urey. It is based on the notion of condensation, where the metal and rock ratios change with distance from the sun. If the condensed solids could react with the vapor, the planets would have accreted homogeneously. If the condensed solids quickly formed larger aggregates, there would have been a zoned or heterogeneous accretion. Both models give similar predictions for the density of planets. There was likely more mixing of materials than in the simple Urey model. It is even possible that mercury owes its high density entirely to the loss of a mantle. Nevertheless, condensation is probably the relevant mechanism for the formation of the terrestrial planets. Self gravitation must have dominated in the case of the Jovian planets.
In Lecture 19 we pointed out that the Earth's crust was depleted in a class of elements known as siderophiles. We are now in a position to understand why this depletion took place. Earlier, we explained that the siderophiles preferred to remain in the reduced state, and follow iron into the core.
Reduction was defined in Lecture 11 as the opposite of oxidation. Oxidation, is the loss of electrons. When metallic elements form ionic bonds, they are said to be oxidized, by chemists, whether the gain of their electrons is to oxygen or some other element or complex. Thus, sodium is oxidized when NaCl is formed, just as iron is oxidized to form the mineral troilite, FeS (see Lecture 14). Indeed, the metals are oxidized in all of the common minerals that we discussed in Lecture 14.
One may apply sufficient heat to an oxide, and produce the free or reduced metal. The minerals that are found in the uncombined state, such as native copper, or the nickel-iron alloy of the Earth's core are said to be in the reduced state.
We can be pretty sure that the Earth's core is primarily reduced iron, or an iron-nickel alloy. But we also know that there are minerals that contain oxidized iron. The so-called ferromagnesian minerals that dominate mafic rocks all have oxidized iron. We therefore have the following situation: when the Earth was formed, iron was neither completely oxidized nor completely reduced.
There is certainly more iron in the core of the Earth than in the mantle, but the percentage is not overwhelming. About a fifth of the iron in the Earth is oxidized, and in the mantle. The great Norwegian geochemist Victor Goldschmidt realized the significance of this division of iron in the early decades of the 20th century. There was, evidently, a competition for oxidizing agents within the bulk Earth. Since most of the silicate minerals are oxides, oxygen itself was the primary oxidizing agent.
Since there was a competition for oxygen, and iron got some but not all, what could be said about other common metals? Goldschmidt called upon thermodynamics for an answer. He looked at the Gibbs energies of formation of the common metallic oxides, and arranged them in order. We may look at these Gibbs energies, crudely, as a measure of the depths of the potential wells when a metal oxide forms by the schematic chemical reaction:
The more negative the Gibbs energies, called
 G's
the more tightly bound the oxide.
G's
the more tightly bound the oxide.
Now suppose we have two hypothetical metals, Ma and Mb.
Goldschmidt realized the significance
of a comparison of the  G's for the two reactions:
G's for the two reactions:
and
Suppose the  G were more negative for
MbO than for MaO,
in other words, suppose MbO were more tightly bound than MaO.
Then if there were competition, Mb would take oxygen
away from Ma.
G were more negative for
MbO than for MaO,
in other words, suppose MbO were more tightly bound than MaO.
Then if there were competition, Mb would take oxygen
away from Ma.
Goldschmidt argued that this is an explanation for the depletion
of the siderophiles. These are all elements that are less
easily oxidized than iron--their oxides are less tightly bound than
FeO. To use the language of thermodynamics, their
 G's
for oxide formation
are less negative than those of iron--they like to form oxides even
less than iron does. Thus they remain reduced.
G's
for oxide formation
are less negative than those of iron--they like to form oxides even
less than iron does. Thus they remain reduced.
Goldschmidt argued that elements that are more easily oxidized than iron would eventually form silicates, and remain in the mantle. These elements, he called lithophiles.
The distinction of some elements as siderophiles and others as lithophiles is part of the geochemical or cosmochemical classification of the elements. The other main division is between volatile and refractory elements, which we have discussed in Lectures 20, and 21.
In Lecture 23 we noted that the lunar samples showed that the Moon's crust was depleted in siderophile elements. This was originally thought to imply that the Moon had a metal core. There was some fragmentary evidence from seismic experiments done on the Moon that this might be so. P waves from a seismic disturbance on the far side of the Moon were recorded by Apollo instruments, while S waves were not. This is explicable if the Moon had a molten core. However, there has been no evidence to confirm this, and over the years less and less has been said by lunar experts about a possible molten core.
Alternate explanations to the depletion of the siderophiles are available. If the Moon formed from the Earth's mantle, after core formation, it would naturally be depleted in siderophiles. It is possible that the Moon did form from mantle material that "boiled" off the Earth, either as a result of a rain of meteoroids, or the Big Whack.
In the case of the Big Whack, there is the question of the fraction of material that belonged to the impactor rather than the Earth. Current thinking is that the impactor was also a body that had differentiated into a mantle and a core. If this had been the case, then a Moon formed from Earth's mantle, impactor's mantle, or a mixture would all show a depletion of siderophiles. In this picture, the core of the impactor would have eventually merged with the Earth's core.
Another property of the lunar rocks that was immediately clear from the initial samples from Apollo 11 was their lack of volatiles. As we have pointed out in Lectures 15 and 16, terrestrial rocks have been substantially altered by weathering. Especially the rocks of the upper crust or sial tend to have complex minerals containing water of hydration, that is, minerals with water chemically bound in the crystals.
The lunar rocks do not show this. Indeed, their mineralogy is quite simple. The three families of minerals that we have discussed, olivines, pyroxenes, and feldspars contain the major lunar minerals. This is true for the Earth's mantle as well, at least if we include oxides--MgO must be prevalent when the silicate perovskite forms. (See Lecture 15: Mg2SiO4 ---> MgSiO3 + MgO.)
We have spared the reader the rather complex chemical formulae for minerals that are the typical weathering products of the olivines, pyroxenes, and feldspars. Here is a relatively simple example of a so-called clay mineral that is a typical weathering product of feldspar: Al4[Si4O10](OH)8. It is important to notice the OH group. OH groups, or hydroxide ions, are typical of the weathering products of olivines and pyroxenes as well as feldspars, and the origin of these OH groups is surely water, H2O (or HOH!).
If there is no water, the earlier minerals, in the sense of the Bowen series, can't weather into their typical weathering products. These minerals are very rare on the moon, and when they are found it is more often the case than not, that a Cl or F ion appears in place of the OH.
We have mentioned the calcium phosphate mineral apatite. The terrestrial mineral commonly has the formula Ca5(PO4)3OH. This is much more common on the Earth than whitlockite, Ca3(PO42. The situation is reversed on the Moon. There is more whitlockite than apatite. Moreover, lunar apatite rarely has an OH to supply the last negative unit of valence. On the Moon apatite is more likely to be Ca5(PO4)3F or Ca5(PO4)3Cl.
These are strong indications that the Moon is a dry place.
We believe that the Earth's mantle is surely "dryer" than its crust, but at least one theory for the origin of the atmosphere and oceans of the Earth is that they were cooked from the interior. We also have some indication from volcanic gases that there is still substantial water in the upper mantle. Much of this water could have been carried into the mantle in subduction zones. Still, as we have seen, there is no indication that lunar materials ever had this much contact with water.
As far as we can tell, the general state of oxidation of lunar materials is lower than those of the Earth's mantle. We shall only consider a simple but critical example. Reduced iron is very rare in native terrestrial rocks, but it is common in lunar rocks, though a minor phase. The fragments are often microscopic rather than the clearly visible blebs of iron that can be seen in the cut faces of chondritic meteorites.
There's an iron oxide that shows the relatively high state of oxidation in Earth materials. Did you ever notice that black stuff in beach sand? Next time you go to the beach, take a magnet and run it through the sand. You'll pick up a lot of those black crystals, because many of them are the mineral magnetite, Fe3O4. In this mineral, two of the irons give up 3 electrons, and are Fe+++, while the remaining iron is Fe++.
The mineral hematite, Fe2O3, is even more common than magnetite and has a higher percentage of the triply oxidized iron. While it is not as common as the feldspars, hematite is the most common source of iron ore. This is at the Earth's surface, of course. Within the mantle, the geochemist Ringwood has estimated that the percentage of triply oxidized iron to be about 4%. This is small, but much larger than in lunar samples, where triply ionized iron is essentially absent.
In 1994 a space vehicle named Clementine investigated the composition of the Moon by techniques of remote sensing. The basic technique was to measure the reflectance of the Moon at two different wavelengths in the infrared, and compare these with light reflected from laboratory samples with known composition. Mostly, this experiment was able to resolve materials with a high content of iron, such as the olivines and pyroxenes of the basaltic maria, from the anorthosites of the highlands. Recall that anorthite is CaAl2Si2O8. Mission scientists examined carefully ejecta from large basins, especially the largest lunar basin of all, known as South Pole Aitken.
Rays from large craters are thought to contain material from some depth in the lunar mantle. The overall conclusions from Clementine's measurements is that the anorthositic crust is somewhat deeper than previously supposed. The implication is that the lunar Al/Fe ratio is higher than had been thought.
We have set out four distinctions in lunar and mantle chemistry. There is one intriguing similarity. In Lecture 20, we discussed the three stable isotopes of oxygen. Relative percentages of the isotopes in common terrestrial materials are as follows: 18O, 0.02%; 17O, 0.04%; and the dominant isotope, 16O, 99.76%. Small variations in the isotopic content of oxygen in polar ice, may indicate the conditions under which the ice formed. There is a rule of the thumb that is useful for remembering the way in which isotopes partition during a chemical compound or physical change. Generally speaking, the heavier isotopes are more tightly bound. Using our picture of a potential well, we can say the well is a little deeper for a heavier isotope.
The rule of the thumb says a liquid would be richer in the heavier isotope than the vapor in equilibrium over it. But the higher the temperature, the less this difference would be.
Consider polar ice forming during the summertime of a terrestrial hemisphere. It would be formed from a vapor that would be relatively enriched in 18O relative to 16O. So in the summer, or generally speaking warmer periods, snow would be enriched in the heavy isotope. During colder epochs, H218O is favored to remain in the liquid rather than the vapor, from which the snow forms. So that vapor would be relatively depleted in the heavier oxygen isotope.
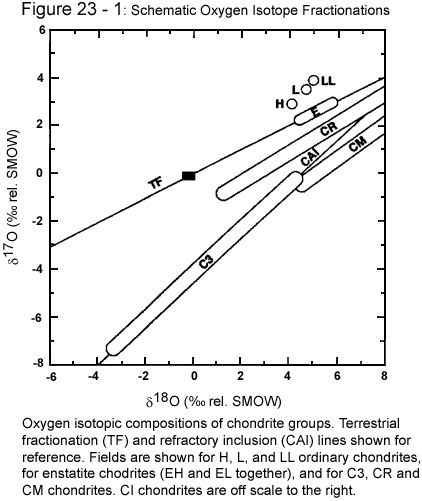
The standard set of isotopic abundances is indicated by a black square in Figure 23-1. Small changes in the relative amounts of the three oxygen isotopes that occur by natural processes, such as those related to temperature, obey a simple law. There are two extra units of atomic mass in 18O relative to 16O, and only one in 17O. Therefore any process that changes the relative abundances of the isotopes would make twice the change to the 18O that it would to the 17O. This is the explanation of the line marked "Terrestrial Fractionation Line" in the figure.
The variations due to natural processes rarely change the relative amounts of the oxygen isotopes by as much as 10% in terrestrial or lunar materials. No difference can be found between the Earth or the moon as far as these isotopes are concerned. Lunar oxygen isotopes are within a few tenths of a per cent of the terrestrial standard, and on the fractionation line.
The situation is different with oxygen isotopes in other cosmic samples, such as the meteorites. On a plot like that of Figure 23-1, meteoritic isotopic abundances are mostly near those of the terrestrial standard, but are generally distinct from it. They do not fall on the terrestrial fractionation line.
It is often assumed that bodies from different positions within the solar system will have distinct isotopic signatures. Indeed, only one class of meteorites has an isotopic signature that is identical to that of the Earth and Moon.
We discussed the theories of the origin of the Moon in Lecture 20. We now return to this topic with the benefit of some of the geochemical background developed subsequently. The historical theories are:
The Big Whack essentially calls for two condensations for the Moon. One would have been for the Earth and impactor separately before the whack, and another one one for the Moon, after the whack. This second condensation would have allowed for additional dispersion of volatiles, and concentration of refractories, which might explain the prevalence of the tough feldspar anorthite on the Moon.
As far as the state of oxidation of the Moon and the Earth are concerned, it is still a bit of a problem. The bulk Earth, mantle plus core, is probably as highly reduced as the moon. Therefore, the Earth's mantle probably never came into equilibrium with its core. If it had, the core would be smaller, and the mantle less oxidized. It is plausible that iron blebs in the proto-Earth fell rapidly through a softened silicate mass, and never equilibrated with it.
One way the Earth's mantle could reach its high state of oxidation is through reactions with water, after the Earth formed. Schematically,
Fe(s) + H2O(l, or g) ---> FeO(s). + H2(g).
This water would have been carried into the bulk of the Earth, adsorbed or imprisoned within planetesimals, and cooked out. After the Big Whack, the water, along with other volatiles, were blown away by the solar wind. This would leave some reduced lunar ion, as is observed.
Victor Goldschmidt introduced a cosmochemical classification of the elements. We discussed siderophiles and lithophiles. Siderophiles prefer the reduced state more than iron, and are favored to end up in the core. Lithophiles prefer the oxidized state, and form the common mantle minerals--silicates and oxides. Competition with iron is the key, since some iron remains oxidized. The lithophiles have a greater affinity for oxidization than iron, and the siderophiles a lesser affinity.
The Moon has (1) less volatiles (2) more reduced iron (3) more refractories (esp. anorthite) than the mantle. These, as well as the Moon's orbit are consistent with the Big Whack hypothesis. But lunar and terrestrial materials have identical oxygen isotopic signatures, for reasons not yet understood.
Mars is about half the size and a tenth the mass of the Earth. In this respect, the bulk of the planet is much less like the Earth than Venus. But people live on the surface of planets, and so from that perspective, it is fair to say that Mars is more like the Earth than Venus. Most of what we shall have to say about Mars concerns its surface. Space probes have landed and orbited Mars. They have sampled its atmosphere and made a start at analyzing its soil and rocky material. Space probes have returned many images of the martian surface. With the return of these images, our view of that planet and the solar system as a whole, changed in a fundamental way.
Mars has been recognized as a planet since the beginning of recorded history, and probably much earlier. But intensive telescopic observations are only a few hundred years old. These observations were made through the Earth's atmosphere, which causes stellar and planetary images to "shimmer." This effect, which the astronomer calls seeing is better at some moments than others. Visual observers could take advantage of moments of the best seeing, while photographic plates would soak up whatever images fell upon them. Visual observers made sketches of what they saw during these favored moments, and some of them led to a great deal of silliness.
The Italian astronomer Schiaparelli referred to some martian features as "canali" (plural) in 1878. The word in Italian may be translated as either canals or simply channels. Unfortunately the Suez Canal, completed in 1869, was too much in people's minds. Some people fixed on these observations as indicating a martian civilization. One of the most enthusiastic of all was the American astronomer, Percival Lowell, who founded an observatory in Flagstaff, Arizona for the express purpose of investigating Mars and its civilization.
Lowell, and perhaps some others, were motivated by wishful thinking. They never convinced mainstream astronomy that they had credible evidence for martian life. Careful observers discovered polar caps, clouds, and seasonal changes that could be attributed to the tilt of Mars's axis of rotation--25o, nearly the same as the Earth's 23.5o. One could, of course, analyze the reflected light from Mars spectroscopically, and in the late 1950's one astronomer announced that he had found the spectroscopic signature of the CH group, which could indicate organic materials. This observation confirmed speculations, not uncommon among astronomers, that some form of vegetation existed on Mars. Some 20 years later, the Viking landers found no trace of organic molecules on the martian surface.
Prior to the space program, Mars and the planets were carefully and successfully investigated as bodies that were subject to Newton's laws. Serious investigations of these objects as worlds in their own right really became possible with the advent of the space program.
The first spacecraft to fly near Mars and return images was Mariner 4. It did not go into orbit about the planet, but merely flew by, taking images as it went past. Two additional probes, Mariners 6 and 7 also performed flybys in 1969. By chance all of the returned images were of the older surfaces in the southern hemisphere.
These images quickly dispelled notions of Mars as a planet with extensive vegetation. It seemed that Mars might be another dead world, like the moon.
Check out the NASA Mars website.
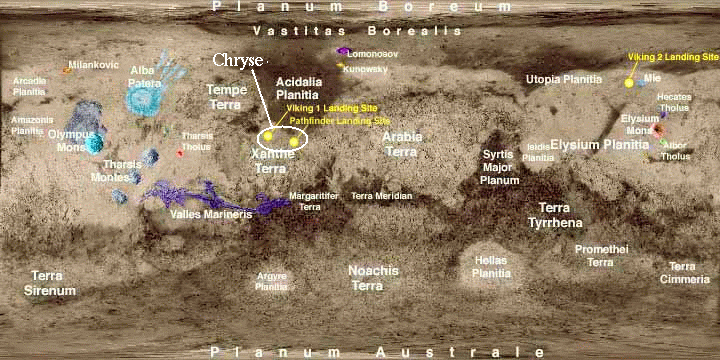
Mariner 9 was the first martian orbiter. When it arrived at the planet in 1971, most of the surface features were hidden by a vast dust storm. It took about two weeks for the dust to clear, and when it finally did, what the mission scientists saw electrified them. Sticking above the dust clouds were the summits of four volcanic mountains! Shortly thereafter, the vast canyon known as Valles Marineris became visible.
As the images from Mariner 9 continued to accumulate, our general view of Mars changed once again. In addition to the mountains and canyons, one whose length would span the continental US, there was extensive evidence that there was once running water on the martian surface. Mars, it seemed, might be dead or dormant now, but its surface had undergone extensive modifications.
The surface of Mars is neither as old as that of the Moon, nor as young as that of the Earth. Crater counts lead us to believe that most of the features we see are nevertheless, more than a billion years old.
After the Mariner 9 mission, the surface of Mars was mapped into four main physiographic provinces.
Maps of the western and eastern hemispheres are coded to show the physiographic classifications of different areas.
There were also attempts to divide the surface into systems, as had been done with the Moon. Indeed, in 1978, the United States Geological Service (USGS) published a Mars map, color coded into three main systems. However, the author of this map wrote me (CRC) only 4 years later that this classification was less satisfactory than for the Moon, and needed revision. We shall therefore content ourselves with the physiographic map, and leave stratigraphic classifications to the future. On the Moon, features changed either because of cratering, or basalt flooding. In the case of Mars, there were additional processes, including water erosion, wind erosion, and wind deposition. In the polar regions, there has been constant seasonal activity.
It appears that useful stratigraphic mapping of Mars requires a closer examination of the surface than is available thus far.
The Viking mission consisted of two orbiters and two landers. The orbiters obtained generally better images than Mariner 9, because of more favorable orbits, but also because many of the Mariner 9 images had been distorted by the dust clouds. Even after the surface features became visible, some haze remained.

Perhaps the most photographed volcanoes are Olympus Mons and three other giant shield volcanoes of the Tharsis Ridge. Most of these volcanoes, though not all, are found in the belt that separates the modified (and volcanic) units of the northern hemisphere from the old cratered plains of the south.
Olympus Mons itself is the largest known volcanic mountain in the solar system. Its basal diameter is perhaps twice that of its nearest rival, Maxwell Montes, a large mountain on Venus. The Hawaiian volcano Mauna Loa, and Mt. Everest have diameters about half that of Maxwell Montes, although their heights are comparable. Olympus Mons is more than double the heights of these three mountains.
When Olympus Mons and its three Tharsis Ridge companions first became visible through the dust storm, Mariner 9 scientists immediately recognized the central calderas as characteristic of volcanism. Calderas are craters, typical of shield volcanoes, and much larger than the central vents of stratovolcanoes. They may have quite flat bottoms, but with additional, smaller craters. The similarity of the central caldera of Olympus Mons to that of the Hawaiian volcano Kilauea is immediately clear from images.
Calderas are formed when the underlying magma chambers are emptied, and support of the overlying layers is lost. The slumping is typically along circular or rounded faults, consistent with the overall shape of shield volcanoes.
The great size of Olympus Mons has been attributed to a hot spot in the martian mantle, similar to that postulated for the Hawaiian volcanic chain. In the latter case, the volcanoes are on a moving plate, so the volcanoes appear in a line as the plate moves over the hot spot. There is no indication of plate tectonics on Mars (or Venus), and this is a possible reason for the size of the martian volcanoes. Lava simply continued to erupt at the same location.
Close images of the flanks of the martian volcanoes show extensive lava flows. These flows took place in leveed channels characteristic of terrestrial lava flows. There is also evidence of collapsed lava tubes, a well-known feature of terrestrial volcanism. Collapsed lava tubes are the best guess at the origin of lunar features called sinuous rilles by astronomers.
On Olympus Mons itself, the lava flows pass over an enigmatic basal scarp, and continue for considerable distance on the Tharsis ridge. The origin of this scarp is quite uncertain. Some have suggested that the central volcanic cone was pushed up. Others speculate that the surrounding landscape has subsided from the weight of the dense volcanic rock that flowed over it. There are no known terrestrial analogues of this scarp. Mauna Loa has a scarp below sea level that may be relevant. But it is only an arc, and does not surround the mountain.
Not all volcanic vents are associated with high mountains. Features known as paterae (sing. patera) show rounded vents with little or know elevation. They can be quite large, and their failure to build mountains is not understood.
The giant Valles Marineris leads eastward from the Tharsis ridge. It is a complex system of canyons that is sometimes compared to our Grand Canyon. This NASA image shows it is not a fair comparison. The square in the center of this picture is already several times the length of the Grand Canyon. Note that Mars itself is only half the size of the Earth.
Surely erosion played a large role in the formation of many of the associated features of Valles Marineris. There are many dendritic feeders at the edges of the canyon, that look much the same as those leading into the Grand Canyon. But it is unclear what forces created a structure so large. At the eastern end of Valles Marineris, the canyon debouches into chaotic terrain which appears connected to flows that lead northward, into the region known as Chryse Planitia, or the ``low plains of Chryse,'' near the site of Viking Lander I.
The frame of Figure 24-3 shows the tear-drop shaped islands in the Chryse region in one of the flow channels. This morphology was noted from the Mariner 9 images, and provided strong support for the hypothesis of extensive water flows in the martian past. In addition to such wide flows, there are numerous elongated sinuous streams with obvious feeders. These are not unlike the lunar sinuous rilles, and in some cases it has been suggested they are formed by groundwater flows, with subsequent collapse. The phenomena would then resemble the collapse of a lava tube, but the liquid would be water rather than magma.
The Viking images showed that the martian craters had a property quite unlike their congeners on the Moon, mercury, or satellites of the Jovian planets. On most size scales, the martian craters were surrounded by a terrace or rampart. This morphology was not noticed in the Mariner 9 images, possibly because of the poorer definition.
The four craters in Figure 24-4 are all relatively near one another, near Chryse. This general morphology may be found for craters as small as 1 km in diameter to those tens of kilometers across. In these four cases, the ramparts are more or less rounded. Yuty Crater, is 18 km in diameter with radial, lobate flows. One can see a smaller, older crater below Yuty, which apparently deflected some--not all--of the outflow in its direction.
Note the rampart on the crater at the top of the ``rounded'' of island of Figure 24-3. Part of the rampart to the left in the image has apparently been washed away. There is a crater at the lower end of the same island that seems to have once had a similar rampart, but most has been obliterated.
Where did all the water go? At present, we do not know. There are a number of lines of evidence that point to the possible presence of water in a kind of permafrost, similar to that found in terrestrial periglacial environments. This might explain the rampart crater morphology. Subsurface ice would be melted by an impact, and cause mud flows that would eventually form the ramparts.
Other features photographed by the Viking landers and orbiters are reminiscent of periglacial phenomena. Some linear surface markings may be the result of seasonal retreats and depositions of an ice sheet. The terrestrial analogue can also be formed by vegetation--which is not to imply a connection with living matter in the martian case.
One intriguing Viking frame shows a region with features resembling patterned ground, which is formed on the Earth in periglacial environments by ice wedges. If the phenomena are related, the martian ``patterns'' are some 200 times larger than are known on the Earth.
Life as we know it would be impossible without liquid water. We will discuss this in detail in Lecture 39. For the present, let us simply note that the possible presence of liquid water at any place in the universe always stimulates questions about the presence of life forms.
The Viking landers performed sensitive experiments to test for life forms in the martian soil. There were four basic experiments. The details need not concern us. We only note that a considerable effort was made to determine whether life forms were present, and that the conclusions were negative.
The actual results from the experiments were somewhat unexpected. In one experiment, for example, a soil sample was ``fed'' a nutrient mixture, and then heated. The basic idea was that microbes in the soil might metabolize the nutrients, giving off characteristic gases, CO2 and water. These could then be detected by instruments in the experimental package.
In fact, gases were released, including some CO2, but because of the absence of any organic molecules, they were attributed to an unusual soil chemistry rather than life forms. The precise nature of the martian soil is still a puzzle, even after results from the Pathfinder-Sojourner (rover) mission of 1997. We have elemental abundances from the rover experiments, but much of the nature of the chemistry and mineralogy of the rocks and soils remain obscure.
Much interest was stimulated in 1996 over the possibility that microscopic traces of past life forms had been discovered in a martian meteorite. The sample itself fell on the Antarctic ice cap in a region known as Allan Hills. It is designated ALH84001.
Here is a link to a NASA site with general information about martian meteorites and putative life. Follow some of the subsequent links to get more detailed information than is given below.
The conclusion that this meteorite originated from the martian surface is based on the following facts. First, the rock is igneous, indeed, an ultramafic (Lecture 16). We know that such materials are produced by melting processes on the Earth and Moon, and presumably other planets. Therefore ALH84001 came from some planet. Its chemistry is not that of either the Earth or Moon, and a sample of the gases cooked out of it in a laboratory experiment, match very closely that of the martian atmosphere.
It is thought that this rock, and several other meteorites were blasted off Mars by impacts. This is plausible. Materials known as tectites were blasted into suborbital flight from the Earth, and returned to the surface bearing the scars of a rapid entrance into the Earth's atmosphere. The tectites have terrestrial chemistry. A few of the Antarctic meteorites show lunar chemistry, and are considered ejecta from impacts on the Moon. All told, it is entirely plausible that the Earth swept up ejecta from a martian impact.
The question of the presence of life forms on Mars, some 3.6 billion years ago is controversial. Advocates point to several lines of evidence, no one of which is convincing, but--they say-- taken together is strong evidence for their hypothesis. We summarize these lines of evidence briefly:
Since the original announcement in the summer of 1996, ALH84001 and possible martian life have been discussed extensively in the popular and scientific literature. Some cogent arguments have been offered against the idea that the "evidence" favors life, and little additional information has been forthcoming. The notion that there was once living matter in ALH84001 seems to be fading.
The martian atmosphere was known to telescopic observers, although definitive measurements were unavailable until the Viking experiments. At ground level, the atmospheric pressure is in the range of 0.006 to 0.009 times that at the Earth's surface. The gas is 95% CO2 with a few per cent nitrogen (N2) and argon (Ar). All other constituents, including the highly variable water vapor, are less than one percent. The martian atmosphere therefore resembles that of Venus, except that it is far thinner. What is notable is that it is so unlike that of the Earth.
The general question of volatiles in the terrestrial planets--water and atmospheric gases--is not well understood. We will take this topic up again in Lecture 39 when we discuss the early Earth.
It has been known since their discovery in 1877 that Mars has two small satellites. The inner one, Phobos is sufficiently close to the planet that its period of revolution is shorter than the martian day, so that it appears to rise in the west, and set in the east. Its mass is only about 10-8 that of the parent planet. Diemos, the outer satellite, is about five times smaller than Phobos.
Both satellites show heavily cratered surfaces, and linear fractures perhaps indicative of fractures of the bodies as a whole. There is no good understanding of the origin of these objects. The orbital properties are well behaved, as one might expect if there had been a kind of co-formation as was once popular for our Moon. This idea has lost its analogy with the dominance of the Big Whack hypothesis of lunar origin. Some have speculated that Phobos and Diemos are captured asteroids. Oxygen isotopic analyses should shed light on this question. This must await a return of samples some time in the next century.
We have already discussed the results of the analyses of martian rock samples by the 1997 Pathfinder mission (cf. Lecture 16). The mineralogy of these rocks was not directly determined, but from the atomic compositions mission scientists inferred compositions intermediate between gabbros (mafic) and granites (felsic). Fine grained rocks of intermediate composition are called andesites. Their coarse-grained congeners are called diorites.
Uninformed and often bizarre speculations about Mars were largely ended by the Mariner and Viking missions. The surface has been more active than the Moon's but is far less active then the Earth's. There are four basic physiographic provinces: Polar, ancient or old-cratered, volcanic, and modified units. There is no evidence of plate tectonic activity. Mars boasts the largest volcano and canyon in the solar system. The modified units include a variety of features, including canyons, modified by running water. There is extensive evidence for river flows, and floods. Water that was once flowing on the surface may currently be located below ground as a kind of permafrost. Many Viking images are reminiscent of periglacial landforms. The Viking lander experiments found no evidence for life. An unusual soil chemistry may account for some anomalous outcomes of these experiments. The Pathfinder-Sojourner mission found andesitic rocks. These indicate a geochemically more evolved surface than the Moon, or even the terrestrial oceanic crust.
Generally speaking, Mars has had considerable surface and geochemical activity. It is still more active than the Moon, but much less so now than in its own past.
Mercury was known to ancients observers. While there is a story that Copernicus had never observed the planet, this is rather hard to imagine. In his time there was little smog, and pollution from city lights could hardly have posed much of a problem.
It is relatively easy to find mercury at favorable times, if you make a little effort. It isn't like Venus, which is a dazzler, but it can still get pretty bright. For northern latitudes, like Ann Arbor's +42o, the best times are:
These times are near or on the equinoxes, the sun will be rising due east and setting due west. The ecliptic plane at these times will rise at an angle of about 71o to the horizon (why?). So one should look for mercury only slightly south of the east or west point on the horizon. It helps if you can look down a road that runs east-west. If you have a good low horizon, you can observe mercury for about a week or 10 days at these favorable times.
Personally, I have only observed it in the east, near the autumnal equinox. It appears as the only bright object on the morning horizon. It's a little hard to spot at first, but once you find it, it's pretty obvious. No nearby stars compare with it in brightness, and as the sky brightens, it is the only object on the horizon.
Until relatively recently, astronomers thought that mercury kept the same face to the sun--that its periods of rotation and revolution were the same. In 1889, the visual observer Schiaparelli, the same fellow who created the flap by discussing martian ``canali,'' announced the discovery of permanent visible markings on mercury. These markings were seen by other observers, among them the American astronomers Barnard and Lowell, who concluded that mercury always faced the sun.
The same conclusion was reached by the experienced French observer A. Dollfus, who described observations he had made in 1950. They showed: That the period of rotation of Mercury is thus found to be equal to the period of revolution, with a precision of better than one in ten thousand. (G. Kuiper and B. Middlehurst eds. The Solar System III: Planets and Satellites, U. Chicago Press 1961, p. 550).
One of the first indications that something was amiss with the notion of the ``phase lock'' of mercury's rotation and revolution came in 1962 when radio astronomers at the University of Michigan observed the planet with their 85-foot telescope, and found it too hot. By 1965, radar observations made at Aricebo, Puerto Rico made it clear that mercury rotates three times for every two revolutions about the sun. Kepler would have loved it.
The strange relation of mercury's periods of rotation and revolution may be roughly explained by imagining that it is "on the way" to a true lock with the sun, that is, to keeping one face to the sun. This is the situation with the Earth and the Moon. Presumably, mercury rotated much faster, so that it's rotational period was much shorter than that of revolution. Tidal forces slowed down this rotation, but now it is stuck so that it still rotates a bit faster than it revolves--turning three times in two revolutions. It seems to be hung in a local minimum of a general energy diagram, where a true phase lock represents the overall minimum. Such a situation is common in nature, but rare in this particular situation--the rotation and revolution of a nearly spherical body.
Newton's magnificent theory does not allow a calculation of the mass of mercury in a first approximation. Recall that for a circular orbit
2
mV GMm
----- = ----
r r^2
from which the mass of the orbiting body, m, cancels. Prior to the space program, the only way to know the mass of mercury was from its disturbance of the orbit of Venus. Now Venus, like mercury, has no satellite, so the only way to know its mass is from the way it perturbs the orbits of mercury and the Earth. In the case of the Earth, we have the Moon's orbit, which allows a rather precise determination of the Earth's mass. The main problem here is the value of the constant of gravitation, G, which is still only known to about 4 decimal places.
In a definitive introductory textbook, Russell, Dugan, and Stuart wrote in 1945 that mercury's density was ``4.1 times that of water.'' By 1961, the canny and knowledgeable Michigan astronomer Dean McLaughlin gave a density of 5 (with no decimal point) in his Introduction to Astronomy (Houghton Mifflin, Co.). The accepted figure today is 5.43.
As far as mercury's orbit is concerned, its density is irrelevant, and its mass almost so. Venus's mass is important, for perturbations of mercury, but that was known much better. It perturbs the Earth-moon system, where the mass has been much more securely known for some time. Thus it appeared, even in at the end of the 19th century, that there was a problem with mercury's orbit that Newton's theory could not account for.
We have mentioned that for the simplest two-body system, the orbit is an ellipse, now and forever. This is when the masses are equivalent to points, and it was known to Newton that if the true masses were spherical, one could treat them as points. If the larger of the two masses is flattened, say, or if there is a third body, then the orbit is no longer an ellipse in perpetuity. In our solar system perturbations from the ideal system are small--at least for the planets. Under these conditions, the orbits are very nearly ellipses. How else would Kepler have found his laws? But over time, the ellipses change slightly. One typical way these ellipses change is that their major axes rotate, with respect to the stars.
Measurements of mercury's orbit show that it rotates at a rate of 574 seconds of arc (0.16 deg) per century. Of this, Newton's theory could account for all but 42 seconds per century. The difference is accounted for by Einstein's (1915) general theory of relativity, and was one of its great triumphs.
Relativistic effects are much greater for mercury than the Earth or Venus, because it is closer to the sun, and because its orbit is more elliptical than those of its congeners. The following table shows the predicted and observed relativistic effects for the three inner terrestrial planets.
Perihelion advance in sec per century
Planet Predicted by Observed
Relativity
mercury 43.03 43.1
Venus 8.6 8.4
Earth 3.8 5.0
(Source: Bless, Discovering the Cosmos 1996)
Mariner 10, launched in 1974 (!!) still provides our best information on the surface features of mercury. They look very much like those of the moon in having basically two physiographic provinces: highlands and lowlands. The Mariner 10 images revealed several prominent escarpments, or scarps, that do not have lunar analogues. There are scarps on the Moon, of course, but not such large ones. An escarpment is a long cliff. In the case of mercury, it is thought that perhaps the crust of the planet shrank, causing parts of it to be or pushed over other regions. Mercury has at least one large multi-ringed impact basin (see below) and it has smoother regions, or plains (lowlands). We are not yet sure if these plains represent basalt flooding or are similar in nature to the Cayley formation of the Moon.
The decompressed density of mercury is the highest in the solar system. We have already discussed the two basic ideas of why this decompressed density is so high:
The latter theory is relatively new, but it is now widely held. Some of its appeal is related to the growing acceptance of impacts as the solution to many puzzles related to the history of the Earth and the solar system. The first steps in the elevation of impacts to a major solver of problems came with their acceptance as the cause of lunar craters. Recent prominence has been given to individual impacts as the origin of the Moon and the cause of the dinosaur extinction.
Just as it might be possible to blast off mercury's mantle, it might be possible to blast off the atmosphere of a terrestrial planet. All of these notions are relatively new and it is too early to know how large a role they may eventually play in our understanding of the history of the solar system.
Since we have no rock samples from mercury, we must estimate ages of its surface from crater counts. Mercury has been divided into systems, like the Moon. We mention only two, the Calorian and the Kupierian.
The Calorian system has about the same age as the lunar Imbrian--3.8 billion years. It is named for the Caloris Basin, the largest, and surely most prominent impact basin on the planet. It is about 10% larger than the lunar Imbrian Basin--on a planet some 40% larger than the Moon. Only a part of Caloris has been photographed, the western half, roughly, lay in an unlit portion of the planet when Mariner 10 flew by.
From casual inspection, Caloris is a garden-variety major impact basin. On closer inspection, inner ring structure can be seen. Caloris seems more like the far side lunar basin Orientale, in this regard, than Imbrium where the basalt flooding has obliterated the inner rings. Close-up images of Caloris show extensive grooves and wrinkles, roughly concentric with the ring structure. These are unlike the basalt-flooded floors of most lunar craters.
We really need more and better images and especially returned samples from mercury. Surely Caloris is an impact basin. But the nature of the smoother plains within the ring system is not well understood.
There has been considerable interest in an area that is antipodal to the Caloris basin. Images of this region are often described as ``weird terrain.'' It is commonly believed that shock waves from the Caloris ``event'' were focused on the opposite side of the planet, and broke up many of the older, smaller craters, so that what are seen are irregular, isolated hills. Note that the rim of the large crater (lower left in the image) is almost completely missing. This crater, and some others in the image, appear to have basalt-flooded floors. Many craters are seen in this region, of course. Presumably they are younger than Caloris.
The Kuiperian system of mercury is named for a small, bright-rayed crater prominent in a series of images from the first approach of Mariner 10 to the planet. A more detailed image shows Kuiper crater, as well as some of the mercurian scarps. The contrast of this image has been decreased, so Kuiper's white rays are not so prominently seen as in the incoming image series. Materials of the Kuperian system are thought to have about the same age as the lunar Copernican, 1 billion years.
Craters on the Moon pass through a sequence of typical shapes that depends approximately on their sizes (diameters, D):
Numerous double-ringed craters may be seen on mercury. The crater Bach appears in the south polar regions of the planet, along with several others. The double-ring structures are not as prominent on the better-known near side of the Moon, but quite a few may be found on the farside as well as polar regions. It seems plausible that this particular structure was obscured or obliterated by the extensive flooding on the lunar near side.
We know very little about the composition of mercury beyond inferences that may be drawn from the bulk density. It is generally assumed that the planet is differentiated into a relatively large metallic core and smaller silicate mantle, although we have no seismic information indicating that this is the case. Mariner 10 scientists were surprised to discover a magnetic field. The surface field is only about 1% that of the Earth's but because of the slow rotation of the planet, no field was expected. The Earth's field is generated by a dynamo mechanism that operates through currents in the liquid outer core. These currents are driven by the Earth's rotation, and it seemed unlikely that anything similar would operate on mercury.
Neither landers nor orbiters have yet visited the planet. About the best inference we may draw about the surface chemistry comes from measurements of the reflectance spectrum in the near infrared.
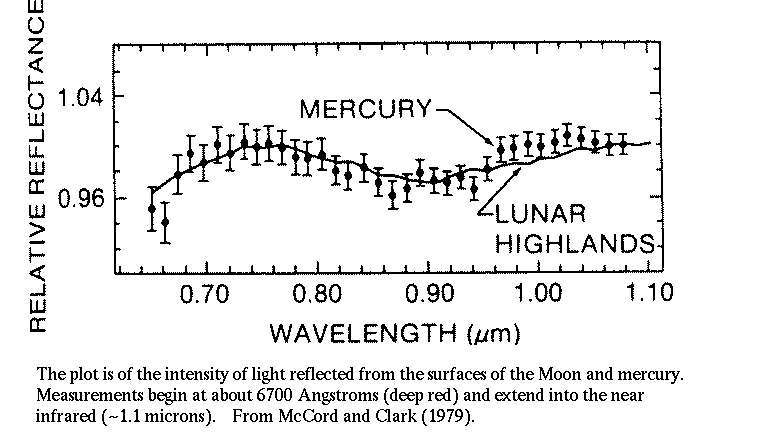
We conclude, from the similarity of the two spectra, that mercury has a (calcic) plagioclase-pyroxene crust. Does this imply global melting and an upward migration of anorthite? At this time we can only keep an open mind until samples are returned (or analyzed in situ).
In an experiment carried out in 1991, radar signals were bounced off mercury and detected by the Very Large Array (VLA) of radio telescopes in New Mexico. The returned signal indicated the possible presence of water ice at mercury's north pole.
Because mercury at one time or another presents all of its surface to the sun, the only possible location for an accumulation of unevaporated ice would be inside a polar crater, where sunlight is never incident. The question of polar ice on mercury remains an intriguing possibility at this time. A similar question exists concerning polar ice on the Moon. We will have a little more to say on this matter in Lecture 34, since comets may have brought ice to the surface of these objects.
Mercury played a key role in the formulation of Einstein's general theory of relativity. Little was known about the planet until well into the 20th century. The 3/2 rotation/revolution relation was discovered by radar measurements in the late 1960's. The Mariner 10 mission flew by the planet 3 times in the mid 1970's. The images revealed a moon-like surface with craters and a giant impact basin known as Caloris. Shock waves from the impact caused ``weird'' terrain in the antipodal regions. Numerous scarps indicate surface pressures possibly derived from crustal shrinking. We know little of the chemistry of the planet. Its core is large relative to its mantle either because (1) dense materials preferentially solidified near the sun, or (2) because a silicate mantle was blasted off the planet. Reflectance spectra from mercury resemble those of the lunar highlands, consistent with a similar chemistry and mineralogy. The possibility of polar ice on mercury as well as the Moon awaits confirmation.
In mass, and radius, Venus is only slightly smaller then the Earth. It's also closer to the Earth than Mars, but what a difference on the surface!
Venus is covered by a thick atmosphere of mostly CO2 gas. At ground level, the pressure is nearly 100 times the Earth's. This atmosphere prevented any information about the surface of Venus until the space age, when experiments finally penetrated the clouds. Landers returned images of a rock-strewn surface, and orbiters have thoroughly mapped the planet using radar.
Even before the radar mapping of orbiters in the late 1970's and 1990's Earth-based radar had revealed that the planet rotated in a direction opposite that of its orbital revolution. Astronomers say the rotation is retrograde, with a sidereal period of 243 days. This is slightly longer than its orbital period, 225 days.
Venus is the only terrestrial planet with a retrograde rotation. The giant outer planet Uranus technically has a retrograde rotation, although its rotational axis is nearly in the plane of its orbit, so its rotation only ``just'' qualifies as being retrograde. Venus, on the other hand, appears to rotate with an axis of rotation within 3.3o of the pole of its orbit.
The standard explanations for the retrograde rotation of Venus and Uranus are two big whacks. To my knowledge, detailed calculations, such as those carried out for the Earth-moon system have not yet been made for Venus. Presumably this situation will change, since we need plausible answers to the questions:
The atmosphere of Venus is 96% CO2 with a little more than 3% N2. The clouds are opaque, due mostly to trace amounts of sulfuric acid (!!), H2SO4, in the form of droplets. Fortunately, there is almost no water, since the acid is more corrosive in solution than in its pure form.
The surface of the planet is truly hot--about 750K. There are also high winds, some 150 miles per hour, as measured by Russian landers. Venus is truly an awful place.
The origins of the atmospheres of the terrestrial planets have been the subject of much speculation. It is generally acknowledged that the region of the solar nebula where they were formed was too hot for the condensation of volatiles such as CO2 or H2O. These molecules either arrived
Both Venus and Mars have atmospheres dominated by CO2, with a few percent of N2, and trace amounts of other gases. The Earth has an atmosphere mostly of N2, about a fifth O2, with enough CO2 to keep the plants alive--for now. Why the difference?
We think the plants are responsible for the plentiful O2 of the Earth's atmosphere, but what happened to the CO2? A plausible solution to the CO2 question on the Earth and Venus goes back to the work of the Nobel Laureate Harold Urey, and to a chemical reaction now named for him.
Consider the follow similar reactions:
or
These chemical reactions express the transformation of silicates to carbonates. They are collectively called "the" Urey reaction(s).
Now we know that there are extensive deposits of carbonate rocks in the Earth, in the form of limestones and related rocks called dolomites [MgCa(CO3)2]. Some time ago a calculation was made to estimate the composition of the Earth's atmosphere if all of the CO2 that is tied up in the limestones and dolomites were released into the atmosphere. The result was that the atmosphere would be comparably thick in CO2 to Venus's atmosphere.
A classical calculation of the amount of CO2 in carbonate rocks gave a figure of 920 x 1020 grams. This figure came from a carefully done study, but it is probably uncertain by a factor of two. Nevertheless, we may compare it to the current mass of the Earth's atmosphere, about 51 x 1020 grams. If all of the CO2 from carbonates were released into the atmosphere, the results would be:
Earth's atmosphere with CO2 from Carbonates Units 10^20 gm oxygen 12.4 nitrogen 38.6 carbon dioxide 920
Thus, the Earth's atmosphere would be thickened by a factor between 10 and 20. If we were to assume the CO2 in rocks had been underestimated by a factor of 2, the increase in mass would be from 51 to 1900 grams, or a factor or 40. This wouldn't get the Earth's atmosphere quite to where Venus's now is, but it's close. Another factor of 2 would do it. We therefore assume that Urey's explanation for the absence of CO2 in the Earth's atmosphere is correct.
Why did this fail to occur on Venus?
There is an interesting history to the story of the Urey reaction as it was applied to Venus. Urey (1952) took the surface temperature of Venus to be 326K or 127 degrees Fahrenheit. This is pretty warm, but nothing like the 750K landers have now measured. We now know that the temperature is actually too high for the Urey reaction to proceed from left to right. A simple calculation shows that for the present conditions of Venus, the Urey reaction would run backwards!!
Urey knew that there was little evidence for water in Venus's atmosphere, so he assumed there were no oceans. Limestones on the Earth are deposited from oceans and lakes, and they are to a large extent the fossil remains of once-living creatures. A good way to view this is to say that living processes catalyzed the Urey reaction on the Earth. Of course, living forms were not classical catalysts, that is, materials that speed a reaction, but are unaffected by it. But they surely sped the transformation of gaseous CO2 on the Earth into carbonate rocks.
You can still read in textbooks that the CO2 has remained in the atmosphere of Venus because there is no water. Now, we know that even if there were water, it's too hot for the Urey reaction to go.
The CO2 on Venus is said to have caused a greenhouse effect. Molecules can very effectively trap infrared radiation, and prevent the cooling. Their rotational and vibrational energy levels have separations commensurate with infrared and microwave photons. The picture is that the more energetic photons from the sun can penetrate the clouds--or could at one time. The planet is much cooler than the sun, and radiates in the infrared. If this radiation is trapped, the planet will heat up.
Environmentalists are concerned that this process is beginning to start on Earth. With the extensive reservoir of CO2 in our limestones, the potential exists for a rapid run away heating.
Venus has now been mapped in great detail by radar measurements taken from orbiters. These measurements have been collected into striking images of mountains and valleys, volcanoes and plains.
Venus has three main physiographic provinces, lowlands, uplands, and highlands. On the map, they are shown in blue, green, and a yellowish brown. (Sorry, in B/W they are gray shades). The map has extensive labeling, but we will only need to know a few. Learn the locations of Ishtar and Aphrodite highlands, including Maxwell Montes, the uplands called Beta and Phoebe Regio (region), and the Atlanta planitia (plain) lowlands. Also learn the Lada Terra uplands. A ``terra'' is an extensive land mass.
Maxwell Montes is the highest point on the planet, and serves as the zero point for Venusian longitude. It is apparently not volcanic in nature, but the result of compression and uplift. There are nearby volcanic mountains in Ishtar. A sketch map with the important names follows. MM is Maxwell Montes.
0 Longitude
--------------------------------------------
| * MM * |
| * Ishtar * Atlanta |
| * * * |
| Beta |
| ite |
| Phoebe A p o d |
| h r |
| |
| L a d a |
---------------------------------------------
The mapping missions of Venus returned an extraordinary amount of information and fabulous images. We refer you to the excellent section on Venus in Calvin Hamilton's Views of the Solar System. Note especially some of his links showing both a false color image and a color-coded physiographic map. There is far too much information from these missions for us to detail them here. We summarize them, following the description of the mission scientists of the Jet Propulsion Laboratory, JPL. This is also a wonderful resource for information on our current views of this planet.
Why are there no plate tectonics on Venus? We really haven't a clue. On the other hand, you may recall that it was a long time before the theory was accepted for the Earth. An eminent geophysicist said it was impossible, and maintained it well into the 1970's. Perhaps it is impossible for Venus.
Venus rotates slowly in a retrograde direction, presumably because of a big whack. Its atmosphere is primarily CO2, and the pressure at ground level is about 90 times that of the Earth. Most of the Earth's compliment of CO2 is in carbonate rocks, and got there by the Urey reaction. This reaction never took place on Venus. It's now so hot the reaction would run backwards. If it were ever much cooler, then there wasn't water and life to catalyze the reaction. Russian landers have revealed rock-strewn fields, not unlike those on Mars. Radar mapping shows extensive volcanic activity. The surface is 0.8 billion or less years old. We listed 3 physiographic provinces, and named 7 surface features: Ishtar and Maxwell Montes, Beta and Phoebe Regio, Atlanta, Aphrodite, and Lada Terra.
The Titius-Bode law (Lecture 4) was enunciated in the late 1700's. It left a gap between Mars and jupiter. What did this gap mean? After the discovery of uranus, in 1781, the Titius-Bode law looked better than ever, and a concerted effort was made by astronomers to find the missing planet between Mars and jupiter.
On the first night of the nineteenth century, 1 January 1801, the Italian astronomer Guiseppe Piazzi observed the minor planet which he later named Ceres. He observed it for more than a month, but was then taken ill. His last observation was on 11 February. When he recovered, it was lost in the morning twilight. More than a month later, the planet might have been visible briefly after sunset in the evening twilight. But Piazzi was unable to find it.
How much of an arc would this object have moved through in the 2 weeks it was observed? A simple P2 = a3 gives a period of 10.5 years, or 547.9 weeks. Piazzi had observed the minor planet for 41 days, or 5.857 weeks. With this period, it would have traversed 5.875/547.9 = 0.0107 of its orbit, or 3.8 degrees as seen from the sun. In actuality, it moved somewhat less than 3 degrees, as observed from the Earth.
Some six weeks later, it would have moved another 4 degrees (again, as seen from the sun). But the Earthbound astronomers could not find it, in spite of assiduous searching. The new planet had moved too far in angle from the last position observed by Piazzi.
Astronomers of the early nineteenth century had a great challenge, to find the new planet. The challenge was answered by the ``prince of mathematicians,'' Carl Friedrich Gauss. Using new analytical methods which bear his name, Gauss was able to determine what astronomers call the orbital parameters of Ceres from the scant observations made by Piazzi. With the help of these, he predicted the position of the planet, which was then rediscovered on the last day of 1801.
Ceres was rediscovered by Von Zach, and on the very next night, independently, by another astronomer, Heinrich Olbers. Olbers is known to astronomers today for posing the question of why the night sky is dark. This question is known as Olbers's Paradox, but the interested reader must read about it in other sources.
Olbers had become so familiar with the stars within the zone of the predicted new planet, that he noted an unfamiliar object near the position where he had found Ceres. This turned out to be a second minor planet, now know as Pallas.
The world had been prepared for one more planet between Mars and Jupiter, but not two. The situation only grew worse, and by 1850 13 minor planets had been discovered. By the turn of the century, the number was about 500, and the process of naming them became embarrassing and downright silly. By 1988, the named or recorded asteroids had reached 3445.
By the end of the nineteenth century, those astronomers who undertook the discovery and orbital determinations of the minor planets must hardly have felt themselves at the forefront of astronomical research. One may imagine the chiding of their colleagues, engaged in the new methods of parallax and radial velocity determinations. ``Yes, another asteroid. So what!''
In 1898, the asteroid eros was discovered. Its mean distance from the sun is 1.458 AU, but its eccentricity is sufficiently large, 0.223, that at perihelion, it is only 105 million miles from the sun. Now if we recall that the AU is 93 million miles, we see that eros approaches the Earth more closely than any other planet. For this reason it became for many years the basis for the fundamental measurement of the astronomical unit itself.
Kepler's third law provides the basis for the relative distances between the planets. However, if we want these distances in miles or kilometers, it is necessary to know one of these distances in absolute units. The basic technique was parallax, the same principle we have discussed in Lecture 7, where the topic was stellar parallax. To get the distance between the Earth and a planet, the only available baseline was the diameter of the Earth itself, or practically speaking, some fraction of it. Astronomers at two separate observatories had to make simultaneous observations of the planet.
Naturally, the accuracy of a parallax measurement increases as the angle itself increases, so it is easier to measure distances to nearby objects than to those far away. In the case of planets, their exact positions are less easy to determine than those of stars. The stars appear as points of light, while the planets appear disk like in the telescope.
Prior to the discovery of eros, astronomers had used observations of Mars and Venus to fix the distance of the AU in miles. Eros offered two advantages. First, it came much closer to the Earth than either of these two planets, and second, it was small enough to appear starlike in the telescopes. For more than half a century, it provided the definitive measurement of the astronomical unit, upon which all astronomical distances are based. Eventually the parallax method was replaced by radar sounding.
Eros has an additional significance for us today. It is one of a class of objects known as the Amor Asteroids, whose orbital paths cross that of the planet Mars. The Apollo Asteroids are of even more interest. Their orbits cross that of the Earth. Some 60 were known in 1990. Finally, a new class of objects was named in 1976; the Aten Asteroids have semi-major axes less than one AU. It has been estimated that perhaps a dozen with diameters of 1 km may exist.
A space probe, NEAR (Near Earth Asteroid Rendezvous) flew by the asteroid eros in late December of 1998. The mission ran into difficulties, but was eventually declared a success. Check the site for details and images.
Calculations show that a body orbiting near the Earth will be swept up in a time period of the order of only 50,000 years. Of course, this is a long time for you or me, but not long in terms of Earth history. The notion of a catastrophic collision with a comet or asteroid has recently become the subject of numerous sci-fi movies and TV programs. There is more than wild speculation behind these ideas. A good, relevant NASA site may be found here. There is also a site that keeps track of near Earth, and possibly dangerous asteroids or comets.
The International Astronomical Union has now approved the "Torino scale" to rate the danger from a near-Earth object. The idea comes from Richter's scale for earthquakes. However, an earthquake's intensity on the Richter scale is directly related to the energy output which can be inferred from by seismological measurements. Seismometers measure the amplitudes of waves, and the energy of a wave is proportional to the square of its amplitude. While it is possible to speak of potential earthquakes, especially in California, the probability of occurrence plays no role in the calculation or estimate of a figure on the Richter scale. For the most part, the Richter scale is applied to actual events.
The Torino scale, on the other hand, deals expressly with potential collisions. The Torino rating is based both on the probability of an Earth collision, and the energy of the impact. As may be seen from the (NASA) diagram, even highly probable impacts have a zero rating on the Torino scale if the energy delivered is small. From a practical point of view, the most dangerous objects would have both a high probability of impact and its impact would be highly energetic. The vertical scale gives the energy of the impact in megatons of TNT (1 metagon is roughly 4 x 1022 ergs or 4 x 1015 Joules). Also given is a rough estimate of the size of an object that would deliver that much energy after falling on the Earth from outer space. Thus an object about 20 meters across would deliver about 1 megaton of energy.
The orbital parameters of asteroids are not random. The semimajor axes of minor planets with well-determined orbits are shown in Figure 27-1.

>p>The traditional interpretation of Figure 27-1 has been in terms of resonances with jupiter's orbit. The fractions on the figure represent the number of times Jupiter revolves, over the number of times asteroids revolve. The idea is that when these fractions are simple whole numbers, it affects the probability that an asteroid will have a given semimajor axis. Let m and n be whole numbers. Then Kepler's third law immediately gives the relevant semimajor axes of interest as:
Each time an asteroid orbits n times, Jupiter has orbited m times and it gets pulled exactly the same way by jupiter's large gravity. This would plausibly move the asteroid from this position. We provide a few numerical values in Table 27-1, since the fractions written on Figure 27-1 may not locate the a-values precisely. The entries are for orbits with fractions (m/n) of a Jovian period.
Table 27-1 a-Values for Orbital Resonance With Jupiter
m n a m n a m n a
2 3 3.970 3 4 4.295 4 5 4.489
2 4 3.278 3 5 3.701 4 7 3.583
2 5 2.824 3 7 2.957 4 9 3.030
2 6 2.510 3 8 2.706
2 7 2.257
2 8 2.065
2 9 1.909
One can see a number of gaps in this plot. These gaps often occur for values of 'a' for which m and n are simple integers. However, a glaring exception is the group at a=3.970 (m=2,n=3). There are arguable missing gaps near 2.257 (m=2, n=7) and 1.909 (m=2, n=9). It turns out that there are circumstances where resonances can actually stabilize orbits, and this explains the group at a=3.970, and possibly a few other positions. However, there is no simple explanation for how this stability arises. An expert with whom I have spoken said that the resonances can interact with one another in such a way that some cause gaps while others lead to stability. Certainly this is the observed situation.
The Trojan asteroids orbit in two clumps, forming equilateral triangles with the sun and jupiter. These two positions, one ahead, and one behind jupiter, are rather well understood from a classical problem in celestial mechanics. It is called the restricted three-body problem. You may recall that the problem of two bodies, sun and jupiter, say, is completely soluble for all time. That is untrue for three bodies, in general, but there is an interesting approximation. If the mass of the third body is so small that it does not disturb the orbits of the other two, additional information can be obtained for this third body that in general is not available for a body with an arbitrary mass. This is the restricted three-body problem.
If the ``third'' or infinitesimal mass is in a position forming an equilateral triangle (all 60o angles) with the other two, it will remain at this position. In terms of a potential, this is like a little, local minimum. So if the infinitesimal mass is jostled slightly from the exact equilibrium position, it will oscillate about that position, but not leave the general area. This is the situation with the Trojan asteroids.
If we simply plot the positions of the asteroids at any definite time, we do not see the gaps in the semimajor axes, because these orbits are elliptical, and their axes are randomly oriented. However, on the link shown, the clustering at two positions on the outer circle is evident. The outer circle is the orbit of jupiter, and the two clusters, of course, are the Trojan asteroids.
The next planet inside Jupiter is Mars, of course. Do note how much ``room'' there is between the two orbits. Very naively, we may think of this as space for the cosmochemical changeover from the terrestrial to the Jovian planets.
As soon as it became known that the asteroid belt contained many objects, speculations began about why there was no true planet in it. One idea from the nineteenth century was that the asteroids were fragments of an exploded planet. But no one, apart from science fiction writers could figure out why an Earth-size planet would explode. Interplanetary collisions are more plausible, and this notion still has some relevance, as we shall see when we discuss meteorites.
The greatest objection to the notion of an exploded planet is the fact that the total mass of asteroids is quite small, of the order or 0.1 to 0.2% of the Earth's mass. Therefore, it seems more likely that the asteroids represent the failure of a planet to form rather than the debris from one that did.
Why would a planet fail to form in the asteroid belt? There are at least two good reasons. First, as we have seen in the previous section, there are numerous resonances with the giant planet Jupiter for objects orbiting in the asteroid belt. These resonances could have prevented coagulation of a sizable planet. They could also have ejected material, sending it either into the inner solar system, or well beyond Neptune.
We must also note that the asteroid belt lies near the snow line, which marks the dividing point between the terrestrial and Jovian planets. Even though we cannot be sure of the precise nature of the formation of these two classes of planets, we can be sure they were quite different. It is therefore not surprising that at the boundary neither mechanism of planet formation was successful.
With modern computers it has been possible to follow orbits of minor bodies using realistic models of the solar system. This means the calculations are not simplified with the assumptions of the classical restricted three-body problem, but include all masses that are thought to be relevant. What these calculations show is that on a long time scale, hundreds of millions, or billions of years, orbits can change their nature in a fundamental way.
Orbits that circle the sun at a nearly constant radius for hundreds or thousands of revolutions, can suddenly change their character in extraordinary ways. They may jump out of the asteroid belt, and enter the inner solar system, or they may loop outwards into the Kuiper belt, beyond pluto. This is chaotic behavior.
Chaotic behavior is sometimes thought to be a departure from deterministic prescriptions--like indeterminacy in quantum mechanics. This is wrong. Chaotic orbits are calculated with Newton's deterministic equations. However, the results are unpredictable in the following sense. Consider the ``initial conditions'' of a calculation. All planets, and an asteroid, have initial positions and velocities. Now if we vary the initial conditions of the asteroid by an imperceptible amount, the net outcome could be completely different.
Exactly the same conditions give exactly the same outcome. What was entirely unexpected about chaos is that with only a tiny change in the initial conditions, huge differences in the outcomes could eventually emerge. It is typical of the calculations that the big differences don't show up right away. Two orbits with tiny differences in the initial conditions could follow one another for a long time. However, once the paths started to diverge, they would do with astounding rapidity.
There are two classes of objects that may cross the Earth's orbit and possibly impact on the Earth. We have already mentioned the Earth-crossing Apollo asteroids. Modern calculations show that if there are around 50 of these bodies, the first 15 would fall onto the Earth at an average rate of one per 170,000 years. We have already mentioned a half life of 50,000 years for a body that orbits "near" the Earth. These figures are of the same order of magnitude. They are entirely in line with our growing realization that impacts with global consequences have and are expected to continue to occur.
Before impacts and big whacks became so fashionable, astronomers took note of them in the textbooks, but seemed to regard them as curiosities with no apparent consequences. This may have been the case in geology too.
The idea that the dinosaurs may have been wiped out by the consequences of a meteoroid impact is largely due to the geologist Walter Alverez. His recent popular book, T.rex and the Crater of Doom (Princeton University Press, 1997) is a fascinating read. His theory is certainly now widely accepted outright, or at least as a leading contender to dinosaur extinction.
Alvarez and his colleagues believe they have located the site of the impact, in Mexico. The crater is actually in the Gulf of Mexico off the Yucatan peninsula. It is named for the nearby Mayan village that does not appear on a large scale map of the region. For additional details, see the paper by Virgil Sharpton on the Chixulub crater or the box by Walter Alvarez.
Alvarez believes some of his colleagues identified his hypothesis of the meteoroid impact with old ``catastrophic'' notions from the geological past. In these ideas, the biblical flood was "the" catastrophe. Hutton and Lyell made their fame in displacing the notions of catastrophe with the principle of uniformatarianism. Naturally, there would have been hesitancy to go ``back to those old concepts." This is ironic, since mainstream geologists now accept the notion that there was a beginning.
The orbital properties of asteroids are interesting enough, and especially when they pose a danger to the Earth and its citizens. Can we say anything about the nature of the objects themselves? Until the methods of remote sensing came into operation, we could only guess that the asteroids were mostly rock. They are mostly too small to show diameters in Earthbound telescopes, although the American astronomer Barnard measured diameters for four of them with the Lick 36-inch and Yerkes 40-inch telescopes.
We now have images of several asteroids from space vehicles well as the Hubble Space Telescope.
Radar observations from the giant radio dish at Arecibo have been a prolific source of information on sizes, rotations, and binarity among asteroids. Yes, some asteroids have been found to be orbiting pairs. From orbits, we get information on masses. Possibly the best information to date comes from the Galileo mission to jupiter, which obtained images of the asteroid Ida and its tiny satellite Dactyl. From Ida's mass and volume, a density of 2.6 plus or minus 0.5 grams per cm3 were obtained by the mission scientists. This density is like that of a typical granite rather than a gabbro (3.0 to 3.2 gm/cm3).
There are several interpretations of a density of 2.6 for Ida. Could the object is porous? Could there be a substantial component of a dense mineral combined with ice? Many more observations and measurements need to be made before we can say that even the basic facts about the composition of even one asteroid are known. And remember, the facts must be known if we are to formulate responsible ideas about the history and evolution of these bodies.
One of the most fruitful techniques for global information about asteroids has been that of remote sensing. In particular, the examination of the reflected light in the visible and near infrared. Spectra of as many as a thousand of these asteroids have been obtained, and classified. Figure 27-2 was adapted from the text of Morrison, Wolff, and Fraknoi (Saunders 1995).
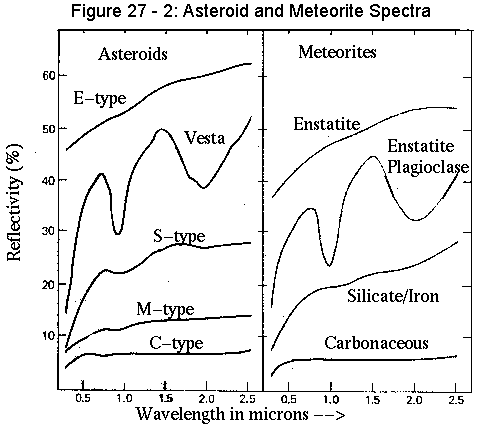
The figure compares spectra of reflected light from asteroids (left) and meteorites (right). The latter, we can study in the laboratory, so that we know their chemical and mineralogical compositions. The most common types of asteroids are type S, thought to be composed of olivine and pyroxene with metal (Fe-Ni alloy) flecks or blebs. The C's are also common. These contain hydrated silicates as well as carbon and organic compounds. They may be related to a meteorite type called carbonaceous, which are composed of anhydrous silicates (e.g. amphiboles, the weathering products of pyroxenes) as well as carbonaceous material.
The asteroid Vesta has a marked signature rather closely matched by the enstatite-plagioclase meteorites. It also resembles reflected light from terrestrial pyroxenes, and is similar to light reflected from the lunar highlands which have a substantial pyroxene component in addition to the plagioclase. Some of the basin basalts also have this spectral signature, displaced to lower reflectivities--the materials are darker, but still with a lot of pyroxene.
Systematics of the orbital parameters and spectral classes show some correlation with large overlaps. This is shown in Figure 26-3 again adapted from Morrison, Wolff, and Fraknoi.
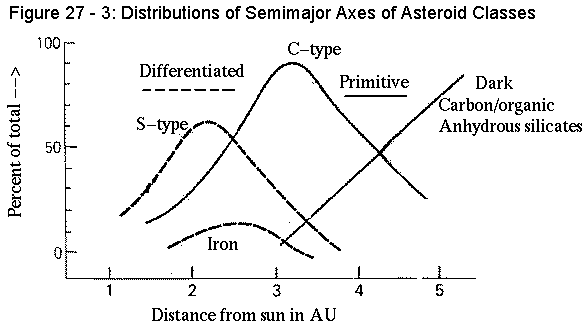
Figure 27-3 has sometimes been interpreted as saying that the most differentiated asteroids occur closer to the sun. This is expected. The snow line falls somewhere within the asteroid belt. If we just use our simple metal-rock-ice recipe for making solar system solids, we realize that a different kind of object is expected beyond the snow line. Asteroids with very low reflectivities, These ``dark'' types may be related to objects of the Kuiper belt and the comets. It is entirely possible that all of the Kuiper belt objects were ejected from the inner solar system. Possibly these dark asteroids were ones left behind, or perhaps even captured again.
A planet predicted between Mars and Jupiter by the Titius-Bode law was discovered in 1801. Soon, others were found. The total mass of these asteroids or minor planets is only 0.1 to 0.2% of the Earth's mass. A large planet never formed in the asteroid belt, either because of perturbations due to jupiter, or perhaps a failure of the methods that formed terrestrial or Jovian planets in the region between the relevant domains. A plot of asteroid semimajor axes reveals gaps that sometimes correspond to resonances with the Jovian period. For some resonances, there is not a gap, but a family of asteroids. The Trojans asteroids have a 1 to 1 resonance with Jupiter. Another little family near a = 4 AU seems happy at a 2 to 3 resonance.
It has been possible to classify the asteroids on the basis of remote sensing. The most fruitful technique uses spectra of light reflected from their surfaces. Most of these spectra can be matched with terrestrial or cosmic materials (meteorites or the lunar surface). The asteroid classes are loosely correlated with distance from the sun.
Detailed calculations show that asteroid orbits can behave chaotically, and there is a real danger to the Earth of a collision. Fortunately, collisions with large asteroids occur once in many tens of thousands of years. The Earth shows scars of past collisions.