We will now develop the core equations used to model the behavior of venom and/or antivenom in the human body. We first study the batch reactor design equations and then apply it along with the techniques used to deal with multiple reactions.

Another important concept we will come across is receptor site coverage by venom and antivenom. The reaction of venom and antivenom molecules with the receptor sites can be modeled as a catalysis problem.

Contents

1. Definitions and Notation
2. Batch Reactor Design
3. Reaction Mechanisms
4. Governing Equations for the Concentration of Components
5. Twitch Height

Definitions and Notation

Site Balance

Believe it or not, but the way in which venom and antivenom interact with receptor sites on the diaphragm muscle may be modeled as a catalytic reaction. The most convenient way to discuss site coverage by either venom or antivenom is to refer to the fraction of sites that are unoccupied (i.e., free sites), occupied by venom, or occupied by antivenom, which are formally defined below. (Oddly enough, the fraction of sites occupied by the venom-antivenom product, f_SP, is never used, since the rate of product leaving a site is considered to be instantaneous.)

f_s = fraction of free sites
f_sa = fraction of sites covered by antivenom
f_sv = fraction of sites covered by venom

Since the total number of sites remains constant with time, the site balance is:
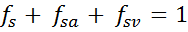
                                           (E-1)

Concentrations

The following notation is used when talking about the concentration of the following components in the blood:

C_v = concentration of venom in the blood
C_a = concentration of antivenom in the blood
C_p = concentration of venom-antivenom product in the blood

The following notation is used when representing receptor sites occupied by venom or antivenom.

C_s0 = initial concentration of receptor sites
C_sv = concentration of sites occupied by antivenom
C_sa = concentration of sites occupied by venom
C_s = concentration of unoccupied free sites

In fact, you can express the concentrations in terms of the fraction of the receptors as shown:

C_sv = f_sv C_s0
C_sa = f_sa C_s0
C_s = f_s C_s0

Batch Reactor Design

The design equation for a batch reactor is:

	(E-2)
If we assume the reactor is well-mixed, then the reaction rate will be constant over every differential portion of volume, and we'll get:
	(E-3)
Where:	= number of moles of venom. = volume of a differential element.
Finally, combining the number of moles of venom in the blood, NV, with the volume will give us the concentration of venom in the blood:
	(E-4)
The development is similar for the other relevant concentrations for antivenom and venom-antivenom product in the blood, CA and CP, respectively.

Reaction Mechanisms

We will now summarize all the reactions that occur along with their appropriate rate constants in the table below.

For your reference,
V = venom
A= antivenom
S = unoccupied receptor site
VS = site occupied by venom
AS = site occupied by antivenom
AV = neutralized product from venom/antivenom reaction

Reaction Number	Description	Mechanism	Rate Constant
1	Adsorption of venom onto the receptor site	V + S → VS	kv
2	Adsorption of antivenom onto the receptor site	A + S ↔ AS	forward: ka backward: kia
3	Reaction of venom with antivenom on the site	V + AS → S + AV	ksa
4	Reaction of antivenom with venom on the site	A + VS → S + AV	ksv
5	Reaction of antivenom and venom in the blood	A + V → AV	kp
6	Removal of antivenom from the system	A → excreted	koa
7	Removal of venom from the system	V → excreted	kov
8	Removal of venom/antivenom conjugate from the system	VA → excreted	kop

Determining the Governing Equations for the Concentration of Components

We will combine material balances and rate laws to determine the governing equations for the following components:

Component	Governing Equation	Derivation
Venom in the Blood
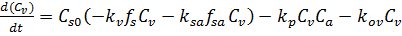Antivenom in the Blood
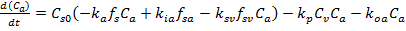Neutralized Venom-Antivenom in the Blood
Sites Occupied by Venom
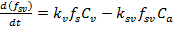Sites Occupied by Antivenom
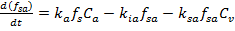Free Sites

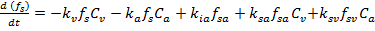Twitch Height (TH)

Another important parameter to explore while studying the pharmacokinetics of snake bites is the twitch height. The twitch height is a measure of muscular function. The blocking of the receptor sites by cobra venom inhibits muscular response to nerve stimulation which can be measured by a decrease in the twitch height. The twitch height decreases due to decrease in muscular response that arises when venom binds to the receptor sites.

The twitch height was calculated from a correlation (D'Hollander 1980) and fitted to experimental data and can be described by the following equation :

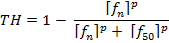
                                            (E-40)

where:         p is the fitted parameter which describes the steepness of the curve
                   f_s0 is the fraction of receptor occupancy which produces a response TH = 0.5
                   f_n is the fraction of sites blocked by antivenom or venom

This suggests that human respiration would get affected by the cobra bite when the twitch height goes below a certain minimum value corresponding to maximum allowable blocking of sites. We will use this to model the muscular responses of a snake bite victim.

The values of p and f_s0 were fitted from the experimental data for various neuromuscular blocking drugs (Mahvina, P.; Hildebrandt, D. ;Fogler, S.H. ;Rubin D., "A Simple Model For The Effectiveness Of Neuromuscular Blocking Drugs" ) An average value of 44.6 and 0.8602 for p and f_s0 respectively will be used by us to model the twitch height.